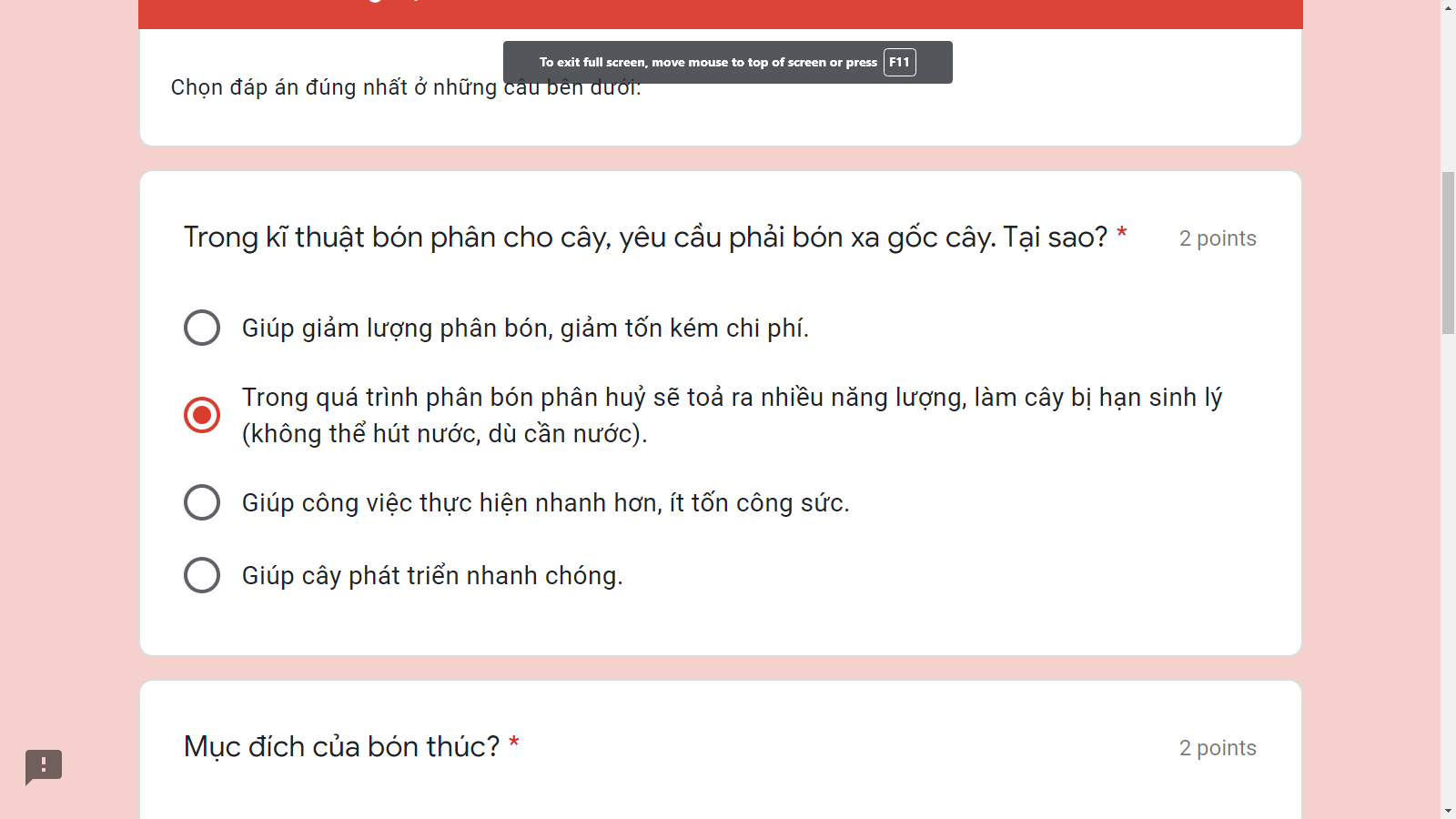
giúp em ạ em cmon
LH
Những câu hỏi liên quan
giúp em với ạ em cmon
1: \(\Leftrightarrow x\left(x+1\right)-x+1=2\)
\(\Leftrightarrow x^2+x-x+1=2\)
\(\Leftrightarrow x^2=1\)
=>x=1(loại) hoặc x=-1(nhận)
2: \(\Leftrightarrow x\left(x+6\right)-x+6=6\)
\(\Leftrightarrow x^2+6x-x+6-6=0\)
=>x(x+5)=0
=>x=0(loại) hoặc x=-5(nhận)
Đúng 2
Bình luận (0)
1: ⇔x(x+1)−x+1=2⇔x(x+1)−x+1=2
⇔x2+x−x+1=2⇔x2+x−x+1=2
⇔x2=1⇔x2=1
=>x=1 hoặc x=-1
2: ⇔x(x+6)−x+6=6⇔x(x+6)−x+6=6
⇔x2+6x−x+6−6=0⇔x2+6x−x+6−6=0
=>x(x+5)=0
=>x=0 hoặc x=-5.
Đúng 0
Bình luận (0)
Bài 1: tính
A=(1/49.44+1/44.39+1/39.34+...+1/14.9+1/9.4) . (1-3-5-7-...-49/89)
giúp em v ạaaa em cmon ạ><
Đặt \(x=\dfrac{1}{49\cdot44}+\dfrac{1}{44\cdot39}+...+\dfrac{1}{14\cdot9}+\dfrac{1}{9\cdot4}\) và y = ... (thừa số thứ hai chưa ghi rõ, nếu ghi rõ thì mới làm được)
Ta có:
\(5x=5\left(\dfrac{1}{49\cdot44}+\dfrac{1}{44\cdot39}+...+\dfrac{1}{14\cdot9}+\dfrac{1}{9\cdot4}\right)\)
\(5x=\dfrac{5}{49\cdot44}+\dfrac{5}{44\cdot39}+...+\dfrac{5}{14\cdot9}+\dfrac{5}{9\cdot4}\)
\(5x=\dfrac{1}{4}-\dfrac{1}{9}+\dfrac{1}{9}-\dfrac{1}{14}+...+\dfrac{1}{39}-\dfrac{1}{44}+\dfrac{1}{44}-\dfrac{1}{49}\)
\(5x=\dfrac{1}{4}-\dfrac{1}{49}=\dfrac{45}{196}\)
\(x=\dfrac{45}{196}\div5=\dfrac{9}{196}\)
Từ đây tự tìm y (thừa số thứ hai)
Suy ra \(A=xy=\dfrac{9}{196}\cdot...=...\)
Đúng 0
Bình luận (0)
mng giải hộ em bài này chi tiết được k v ạ em cmon nhieeufu ạ
\(\orbr{\frac{1}{1-\sqrt{x}}-\frac{1}{\sqrt{x}}]}\div\orbr{\begin{cases}\\\end{cases}(2\sqrt{x}-1)(\frac{1}{1-\sqrt{x}}+\frac{\sqrt{x}}{1-\sqrt{x}+x})]}\)
sori mng em bị lag xíu
mọi người giải giúp em ik ạ pls em gấp lắmmm
Giúp mik vs ạ, cmon nhìu
Cứu em vs em cmon trước
Đề bài của em đâu?
Đúng 0
Bình luận (0)
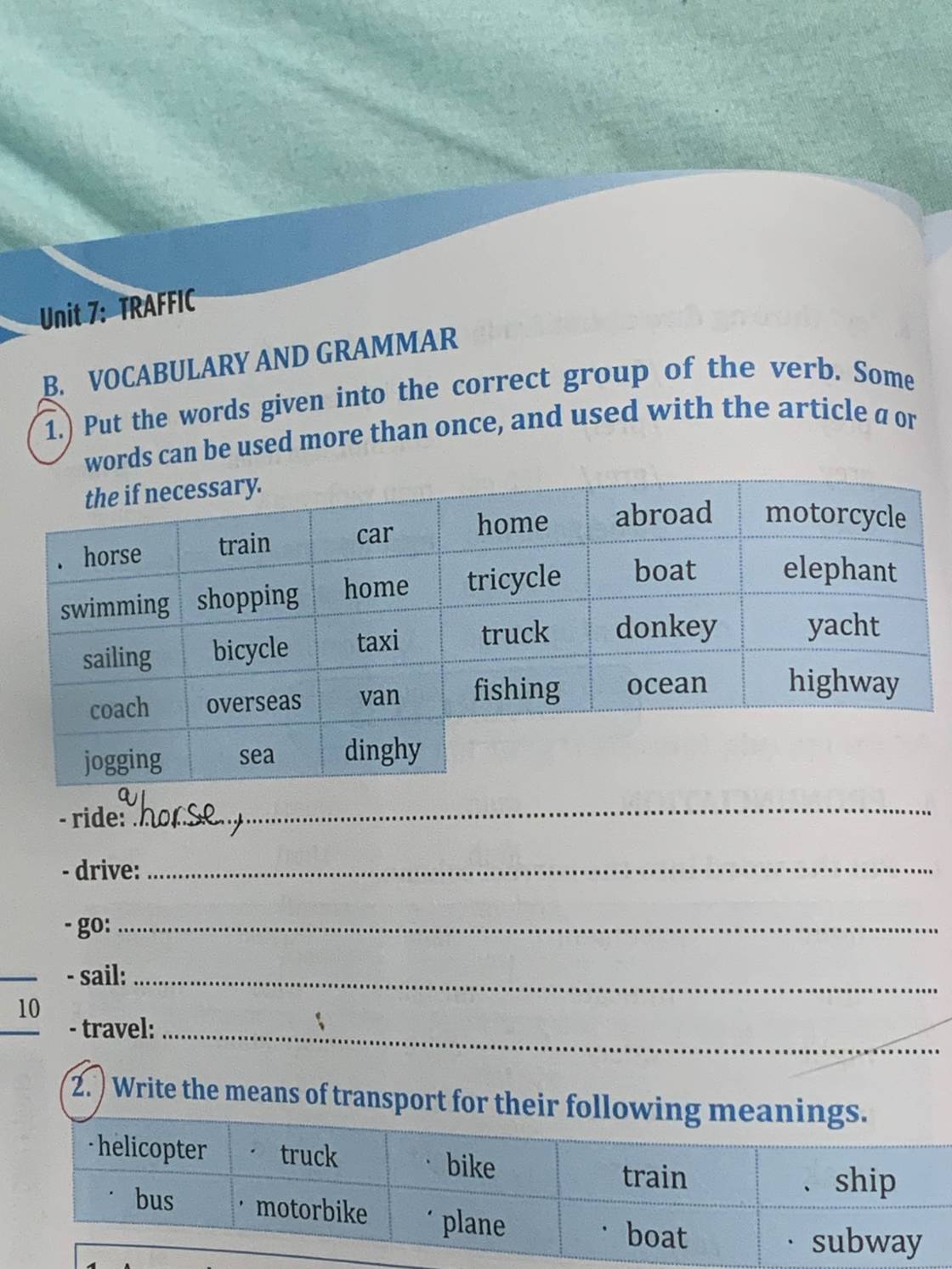 giúp mik vs ạ mik cần gấp a
giúp mik vs ạ mik cần gấp a
mik cmon mn
ride : a horse, a motorbike, a tricycle, an elephent, a bicycle, a donkey,
drive: a car, a train, a taxi, a truck, a coach, a van, on the highway, home
go: home, abroad, swimming, shopping, sailing, overseas, fishing, jogging
sail: a boat, a yatch, a dighy
travel: abroad, overseas, the ocean, the sea
Đúng 0
Bình luận (0)
Cú em 4 câu này i ạa em cmon trướccc
Pt: x^2-2x- căn 3 +1=0 A= x1^2x2^2 -2x1x2-x1-x2 Giúp tớ nhaaaa cmon ạ
\(x^2-2x-\sqrt{3}+1=0\)
\(\Delta'=1^2+\sqrt{3}-1=\sqrt{3}>0\)
⇒ Phương trình có hai nghiệm phân biệt
Theo Viét : \(\left\{{}\begin{matrix}x_1+x_2=2\\x_1.x_2=1-\sqrt{3}\end{matrix}\right.\)
Ta có : \(A=x_1^2.x_2^2-2x_1x_2-x_1-x_2\)
\(=\left(x_1x_2\right)^2-2x_1x_2-\left(x_1+x_2\right)\)
\(=\left(1-\sqrt{3}\right)^2-2\left(1-\sqrt{3}\right)-2=4-2\sqrt{3}-2+2\sqrt{3}-2=0\)
Vậy....
Đúng 3
Bình luận (0)
Giups mình câu này với ạ, mình cmon:(