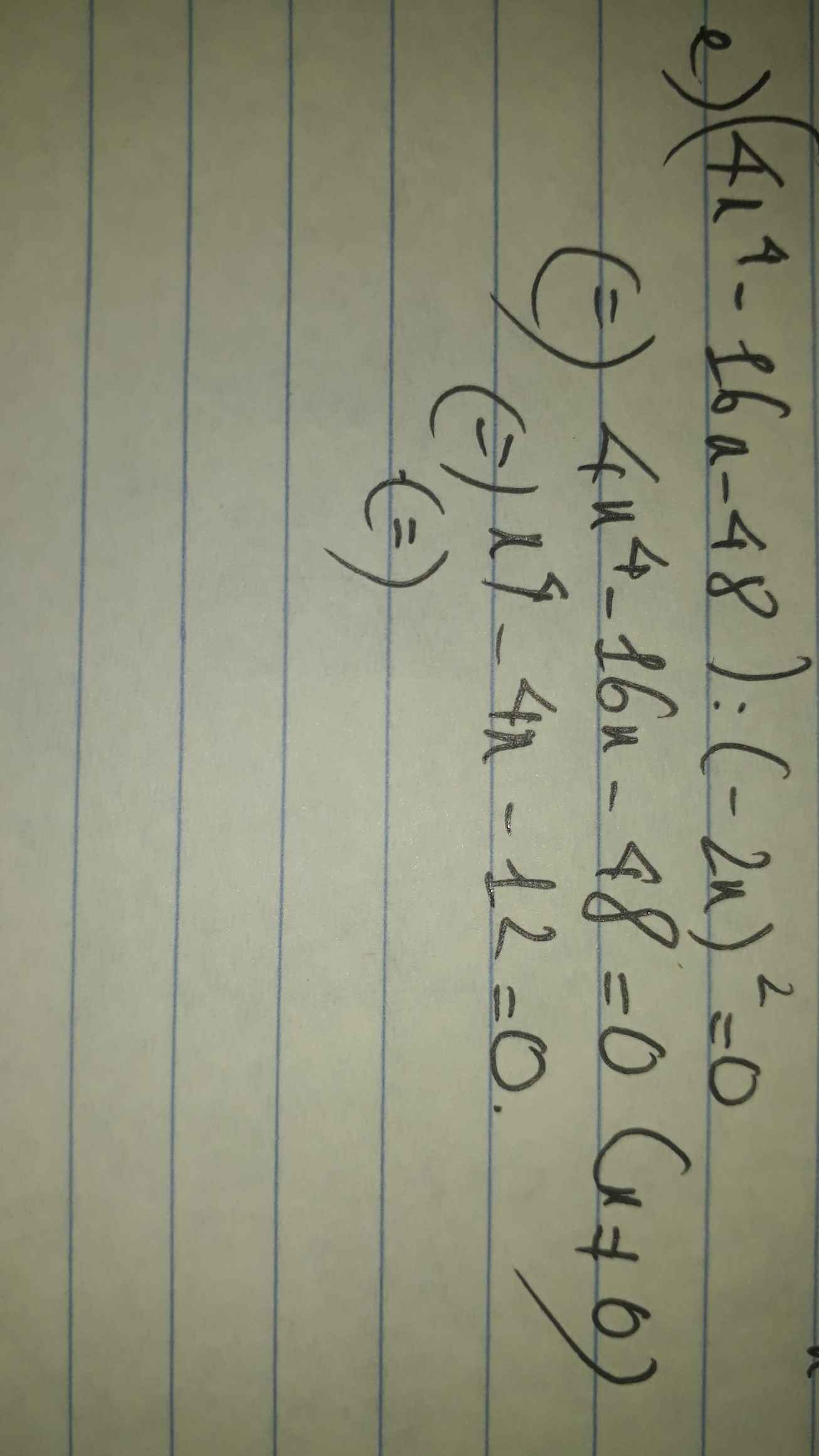X^5+5X^4+2X^3+16X^2+10X+20=0
NQ
Những câu hỏi liên quan
1) x(x-5)-4x+20=0
2) 3(x+1)+x(x+1)
3) 2x^3+x=0
4) x^3-16x=0
5) x^2+6x=-9
6) x^4-2x^3+10x^2-20x=0
7) (2x-3)^2=(x+5)^2
1, x(x - 5) - 4x + 20 = 0
=> x(x - 5) - 4(x - 5) = 0
=> (x - 4)(x - 5) = 0
=> x - 4 = 0 hoặc x - 5 = 0
=> x = 4 hoặc x = 5
=> x thuộc {4; 5}
2, 3(x + 1) + x(x + 1)
= (3 + x)(x + 1)
3, 2x3 + x = 0
=> x(2x2 + 1) = 0
=> x = 0 hoặc 2x2 + 1 = 0
=> x = 0 hoặc 2x2 = -1
=> x = 0 hoặc x2 = -1/2 (vô lí vì x2 > hoặc = 0 với mọi x)
=> x = 0
4, x3 - 16x = 0
=> x(x2 - 16) = 0
=> x = 0 hoặc x2 - 16 = 0
=> x = 0 hoặc x2 = 16
=> x = 0 hoặc x = 4 hoặc x = -4
=> x thuộc {-4; 0; 4}
5, x2 + 6x = -9
=> x2 + 6x + 9 = 0
=> x2 + 2.3.x + 32 = 0
=> (x + 3)2 = 0
=> x + 3 = 0
=> x = -3
6, x4 - 2x3 + 10x2 - 20x = 0
=> x2(x2 + 10) - 2x(x2 + 10) = 0
=> (x2 + 2x)(x2 + 10) = 0
=> x(x +2)(x2 + 10) = 0
-TH1: x = 0
-TH2: x + 2 = 0 => x = -2
-TH3: x2 + 10 = 0 => x2 = -10 (vô lí vì x2 > hoặc = 0 với mọi x)
=> x thuộc {0; -2}
7, (2x - 3)2 = (x + 5)2
-TH1: 2x - 3 = x + 5
=> x = 8
- TH2: - 2x + 3 = x + 5
=> -3x = 2
=> x = \(\frac{-2}{3}\)
- TH3: 2x - 3 = - x - 5
=> 3x = -2
=> x = \(\frac{-2}{3}\)
- TH4: - 2x + 3 = - x - 5
=> -x = -8
=> x = 8`
=> x thuộc {\(\frac{-2}{3}\); 8}
Đúng 0
Bình luận (0)
giải hệ phương trình và phương trinh sau
1, 2x4 - 5x3 + 6x2 - 5x + 2 0
2, 9x4 - 30 x3 + 15x2 - 10x + 1
3 , 8x4 - 10x3 - 6x2 +5x + 2
4 , x4 + 4x3 + x2 + 4x +1 0
5 , 2x4 - 21x3 + 74x2 + 105x + 50 0
6 , 2x4 + x3 - 11x2 + x + 2 0
7 , 2x4 + 3x3 -16x2 +3x + 2 0
8, x4 - 2x3 - 6x2 + 16x + 8 0
Đọc tiếp
giải hệ phương trình và phương trinh sau
1, 2x4 - 5x3 + 6x2 - 5x + 2 = 0
2, 9x4 - 30 x3 + 15x2 - 10x + 1
3 , 8x4 - 10x3 - 6x2 +5x + 2
4 , x4 + 4x3 + x2 + 4x +1 = 0
5 , 2x4 - 21x3 + 74x2 + 105x + 50 = 0
6 , 2x4 + x3 - 11x2 + x + 2 = 0
7 , 2x4 + 3x3 -16x2 +3x + 2 = 0
8, x4 - 2x3 - 6x2 + 16x + 8 = 0
a) (2x+5)(x-3)=(x-4)(3-x)
b) 18x²(x+4)-12(x²+4x)=0
c) 16x²-10x+1=2(5x-1)(3x-4)
d) 3x²-6x+3=(x-1)(4x-5)
a) (2x + 5)(x - 3) = (x - 4)(3 - x)
<=> (2x + 5)(x - 3) + (x - 3)(x - 4) = 0
<=> (2x + 5 + x - 4)(x - 3) = 0
<=> (3x + 1)(x - 3) = 0
<=> \(\left[{}\begin{matrix}3x+1=0\\x-3=0\end{matrix}\right.\)
<=> \(\left[{}\begin{matrix}x=-\frac{1}{3}\\x=3\end{matrix}\right.\)
Vậy S = {-1/3; 3}
b) 18x2(x + 4) - 12(x2 + 4x) = 0
<=> 18x2(x + 4) - 12x(x + 4) = 0
<=> 6x(x + 4)(3x - 2) = 0
<=> \(\left[{}\begin{matrix}x=0\\x+4=0\\3x-2=0\end{matrix}\right.\)
<=> \(\left[{}\begin{matrix}x=0\\x=-4\\x=\frac{2}{3}\end{matrix}\right.\)
Vậy S = {0; -2; 2/3}
Phân tích đa thức thành nhân tửa) x³-3x²+3x-1-8y³b) x⁴-4x³+8x²-16x+16Giải pta) 6(x-3) +(x-1) ²-(x+1) ²2xb) (x+4) ²-(x+8) (x-8) 96c) 4x²-1(2x+1) (3x-5) d) 2x²-x3-6xe) 2x³+5x²-3x0f) x(2x-7) -4x+140g) (2x-5) ²-(x+2) ²0h) (3x+1) (7x+3) (5x-7) (3x+1) i) x²+10x+25-4x(x+5) 0k))(4x-5) ²-2(16x²-25) 0l) (4x+3) ²4(x²-2x+1) m) x²-11x+280n) 3x³-3x²-6x0o) x²-9x+200
Đọc tiếp
Phân tích đa thức thành nhân tử
a) x³-3x²+3x-1-8y³
b) x⁴-4x³+8x²-16x+16
Giải pt
a) 6(x-3) +(x-1) ²-(x+1) ²=2x
b) (x+4) ²-(x+8) (x-8) =96
c) 4x²-1=(2x+1) (3x-5)
d) 2x²-x=3-6x
e) 2x³+5x²-3x=0
f) x(2x-7) -4x+14=0
g) (2x-5) ²-(x+2) ²=0
h) (3x+1) (7x+3) =(5x-7) (3x+1)
i) x²+10x+25-4x(x+5) =0
k))(4x-5) ²-2(16x²-25) =0
l) (4x+3) ²=4(x²-2x+1)
m) x²-11x+28=0
n) 3x³-3x²-6x=0
o) x²-9x+20=0
\(o,x^2-9x+20=0\)
\(\Leftrightarrow x^2-4x-5x+20=0\)
\(\Leftrightarrow x\left(x-4\right)-5\left(x-4\right)=0\)
\(\Leftrightarrow\left(x-4\right)\left(x-5\right)=0\)
\(\Leftrightarrow\orbr{\begin{cases}x-4=0\\x-5=0\end{cases}}\)
\(\Leftrightarrow\orbr{\begin{cases}x=4\\x=5\end{cases}}\)
Đúng 0
Bình luận (0)
\(n,3x^3-3x^2-6x=0\)
\(\Leftrightarrow3x\left(x^2-x-2\right)=0\)
\(\Leftrightarrow3x\left(x^2+x-2x-2\right)=0\)
\(\Leftrightarrow3x\left[x\left(x+1\right)-2\left(x+1\right)\right]=0\)
\(\Leftrightarrow3x\left(x+1\right)\left(x-2\right)=0\)
\(\Leftrightarrow\orbr{\begin{cases}\orbr{\begin{cases}3x=0\\x+1=0\end{cases}}\\x-2=0\end{cases}}\Leftrightarrow\orbr{\begin{cases}\orbr{\begin{cases}x=0\\x=-1\end{cases}}\\x=2\end{cases}}\)
Đúng 0
Bình luận (0)
\(m,x^2-11x+28=0\)
\(\Leftrightarrow x^2-4x-7x+28=0\)
\(\Leftrightarrow x\left(x-4\right)-7\left(x-4\right)=0\)
\(\Leftrightarrow\left(x-4\right)\left(x-7\right)=0\)
\(\Leftrightarrow\orbr{\begin{cases}x-4=0\\x-7=0\end{cases}}\)
\(\Leftrightarrow\orbr{\begin{cases}x=4\\x=7\end{cases}}\)
\(l,\left(4x+3\right)^2=4\left(x^2-2x+1\right)\)
\(\Leftrightarrow16x^2+24x+9=4x^2-8x+4\)
\(\Leftrightarrow16x^2+24x+9-4x^2+8x-4=0\)
\(\Leftrightarrow12x^2+32x+5=0\)
\(\Leftrightarrow\left(x+\frac{1}{6}\right)\left(x+\frac{5}{2}\right)=0\)
\(\Leftrightarrow\orbr{\begin{cases}x+\frac{1}{6}=0\\x+\frac{5}{2}=0\end{cases}\Leftrightarrow}\orbr{\begin{cases}x=-\frac{1}{6}\\x=-\frac{5}{2}\end{cases}}\)
Đúng 0
Bình luận (0)
Xem thêm câu trả lời
Tìm x:
a) x(2-x)+(x2+x)=7
b) (2x+1)2-x(4-5x)=17
c) (4-x)2-(2x+1)2=0
d) (2x3-8x2+10x) : (2x)=0
e) (4x4-16x-48) : (-2x)2=0
a: Ta có: \(x\left(2-x\right)+\left(x^2+x\right)=7\)
\(\Leftrightarrow2x-x^2+x^2+x=7\)
\(\Leftrightarrow3x=7\)
hay \(x=\dfrac{7}{3}\)
b: Ta có: \(\left(2x+1\right)^2-x\left(4-5x\right)=17\)
\(\Leftrightarrow4x^2+4x+1-4x+5x^2=17\)
\(\Leftrightarrow9x^2=16\)
\(\Leftrightarrow x^2=\dfrac{16}{9}\)
hay \(x\in\left\{\dfrac{4}{3};-\dfrac{4}{3}\right\}\)
Đúng 0
Bình luận (0)
c: Ta có: \(\left(x-4\right)^2-\left(2x+1\right)^2=0\)
\(\Leftrightarrow\left(x-4-2x-1\right)\left(x-4+2x+1\right)=0\)
\(\Leftrightarrow\left(x+5\right)\left(x-1\right)=0\)
\(\Leftrightarrow\left[{}\begin{matrix}x=-5\\x=1\end{matrix}\right.\)
d: ta có: \(\dfrac{2x^3-8x^2+10x}{2x}=0\)
\(\Leftrightarrow x^2-4x+5=0\)
\(\Leftrightarrow\left(x-2\right)^2+1=0\)(vô lý)
Đúng 0
Bình luận (0)
Mn giúp mình vs1, x^3-6x^2+10x-402, x^3+2x^2+2sqrt{2}x+2sqrt{2}03, x^4+x^2-sqrt{2}x+20
4, x^4+5x^3-12x^2+5x+105, left(x+5right)left(2x+12right)left(2x+20right)left(x+12right)3x^26, left(x^2-5x+1right)left(x^2-4right)6left(x-1right)^27, x^4-9x^3+16x^2+18x+40
Đọc tiếp
Mn giúp mình vs
1, \(x^3-6x^2+10x-4=0\)
2, \(x^3+2x^2+2\sqrt{2}x+2\sqrt{2}=0\)
3, \(x^4+x^2-\sqrt{2}x+2=0
\)
4, \(x^4+5x^3-12x^2+5x+1=0\)
5, \(\left(x+5\right)\left(2x+12\right)\left(2x+20\right)\left(x+12\right)=3x^2\)
6, \(\left(x^2-5x+1\right)\left(x^2-4\right)=6\left(x-1\right)^2\)
7, \(x^4-9x^3+16x^2+18x+4=0\)
1. \(x^3-6x^2+10x-4=0\)
<=> \(\left(x^3-2x^2\right)-\left(4x^2-8x\right)+\left(2x-4\right)=0\)
<=> \(\left(x-2\right)\left(x^2-4x+2\right)=0\)
<=> \(\orbr{\begin{cases}x=2\\x^2-4x+2=0\left(1\right)\end{cases}}\)
Giải pt (1): \(\Delta=\left(-4\right)^2-4.2=8>0\)
=> pt (1) có 2 nghiệm: \(x_1=\frac{4+\sqrt{8}}{2}=2+\sqrt{2}\)
\(x_2=\frac{4-\sqrt{8}}{2}=2-\sqrt{2}\)
1) Ta có: \(x^3-6x^2+10x-4=0\)
\(\Leftrightarrow\left(x^3-2x^2\right)-\left(4x^2-8x\right)+\left(2x-4\right)=0\)
\(\Leftrightarrow x^2\left(x-2\right)-4x\left(x-2\right)+2\left(x-2\right)=0\)
\(\Leftrightarrow\left(x^2-4x+2\right)\left(x-2\right)=0\)
+ \(x-2=0\)\(\Leftrightarrow\)\(x=2\)\(\left(TM\right)\)
+ \(x^2-4x+2=0\)\(\Leftrightarrow\)\(\left(x^2-4x+4\right)-2=0\)
\(\Leftrightarrow\)\(\left(x-2\right)^2=2\)
\(\Leftrightarrow\)\(x-2=\pm\sqrt{2}\)
\(\Leftrightarrow\)\(\orbr{\begin{cases}x=2+\sqrt{2}\approx3,4142\left(TM\right)\\x=2-\sqrt{2}\approx0,5858\left(TM\right)\end{cases}}\)
Vậy \(S=\left\{0,5858;2;3,4142\right\}\)
4) \(x^4+5x^3-12x^2+5x+1=0\)
<=> \(\left(x^4-x^3\right)+\left(6x^3-6x^2\right)-\left(6x^2-6x\right)-\left(x-1\right)=0\)
<=> \(\left(x^3+6x^2-6x-1\right)\left(x-1\right)=0\)
<=> \(\left[\left(x-1\right)\left(x^2+x+1\right)-6x\left(x-1\right)\right]\left(x-1\right)=0\)
<=> \(\left(x-1\right)^2\left(x^2-5x+1\right)=0\)
<=> \(\orbr{\begin{cases}x=1\\x^2-5x+1=0\left(1\right)\end{cases}}\)
Giải pt (1) ta có: \(\Delta=\left(-5\right)^2-4=21>0\)
=> pt có 2 nghiệm
\(x_1=\frac{5+\sqrt{21}}{2}\); \(x_2=\frac{5-\sqrt{21}}{2}\)
Xem thêm câu trả lời
tim x
x^2-5x-4(x-5)=0
2x(x+6)=7x+42
x^3-5x^2+x-5=0
x^4-2x^3+10x^2-20x=0
(2x-3)-x^2+10x-25=0
\(x^2-5x-4\left(x-5\right)=0\)
\(\Leftrightarrow\)\(x\left(x-5\right)-4\left(x-5\right)=0\)
\(\Leftrightarrow\)\(\left(x-5\right)\left(x-4\right)=0\)
\(\Leftrightarrow\)\(\orbr{\begin{cases}x-5=0\\x-4=0\end{cases}}\)
\(\Leftrightarrow\)\(\orbr{\begin{cases}x=5\\x=4\end{cases}}\)
Vậy....
\(2x\left(x+6\right)=7x+42\)
\(\Leftrightarrow\)\(2x\left(x+6\right)-7x-42=0\)
\(\Leftrightarrow\)\(2x\left(x+6\right)-7\left(x+6\right)=0\)
\(\Leftrightarrow\)\(\left(x+6\right)\left(2x-7\right)=0\)
\(\Leftrightarrow\)\(\orbr{\begin{cases}x+6=0\\2x-7=0\end{cases}}\)
\(\Leftrightarrow\)\(\orbr{\begin{cases}x=-6\\x=\frac{7}{2}\end{cases}}\)
Vậy......
\(x^3-5x^2+x-5=0\)
\(\Leftrightarrow\)\(x^2\left(x-5\right)+\left(x-5\right)=0\)
\(\Leftrightarrow\)\(\left(x-5\right)\left(x^2+1\right)=0\)
\(\Leftrightarrow\)\(x-5=0\)
\(\Leftrightarrow\)\(x=5\)
\(x^4-2x^3+10x^2-20x=0\)
\(\Leftrightarrow\)\(x^3\left(x-2\right)+10x\left(x-2\right)=0\)
\(\Leftrightarrow\)\(x\left(x-2\right)\left(x^2+10\right)=0\)
\(\Leftrightarrow\)\(\orbr{\begin{cases}x=0\\x-2=0\end{cases}}\)
\(\Leftrightarrow\)\(\orbr{\begin{cases}x=0\\x=2\end{cases}}\)
Vậy...
Đúng 0
Bình luận (0)
Tính giá trị của biểu thức
a) Ax3-30x2-31+1 tại x31
b) Bx5-15x4+16x3-29x2+13x tại x14
c) Cx14-10x13+10x12-10x11+...+10x2-10x+10 tại x9
d) D7x(x-5)+3(x-2) tại x0
e) E5-4x(x-2)+4x2 tại x4
f) F4x2-2x+3x(x-5) tại x-1
g) G(5x-7)(7x+3)-(7x+2)(5x-4) tại x-3
h) H(-5x+4)(3x-2)+(-2x+3)(x-2) tại x-2
Đọc tiếp
Tính giá trị của biểu thức
a) A=x3-30x2-31+1 tại x=31
b) B=x5-15x4+16x3-29x2+13x tại x=14
c) C=x14-10x13+10x12-10x11+...+10x2-10x+10 tại x=9
d) D=7x(x-5)+3(x-2) tại x=0
e) E=5-4x(x-2)+4x2 tại x=4
f) F=4x2-2x+3x(x-5) tại x=-1
g) G=(5x-7)(7x+3)-(7x+2)(5x-4) tại x=-3
h) H=(-5x+4)(3x-2)+(-2x+3)(x-2) tại x=-2
Mấy bài kia phá tung tóe rồi rút gọn hết sức xong thay x vào, làm câu c thôi nhé:
c) \(C=x^{14}-10x^{13}+10x^{12}-10x^{11}+...+10x^2-10x+10\)
riêng câu này ta thay x = 9 vào luôn, vậy ta có:
\(C=9^{14}-10\cdot9^{13}+10\cdot9^{12}-10\cdot9^{11}+...+10\cdot9^2-10\cdot9+10\)
\(=9^{14}-\left(9+1\right)\cdot9^{13}+\left(9+1\right)\cdot9^{12}-\left(9+1\right)\cdot9^{11}+...+\left(9+1\right)\cdot9^2-\left(9+1\right)\cdot9+10\)
\(=9^{14}-9^{14}-9^{13}+9^{13}+9^{12}-9^{12}-9^{11}+...+9^3+9^2-9^2-9+10\)
\(=-9+10\)
\(=1\)
Đúng 0
Bình luận (1)
tìm x:
x^3-16x=0
x^4-2x^3+10x^2-20x=0
(2x-3)^2=(x+5)^2
x^2(x-1)-4x^2+8x-4=0
*\(\left(2x-3\right)^2=\left(x+5\right)^2\)
\(\Rightarrow\left(2x-3\right)^2-\left(x+5\right)^2=0\)
\(\Rightarrow\left(2x-3-x-5\right)\left(2x-3+x+5\right)=0\)
\(\Rightarrow\left(x-8\right)\left(3x+2\right)=0\)
\(\Rightarrow\left[{}\begin{matrix}x=8\\x=-\dfrac{2}{3}\end{matrix}\right.\)
Đúng 0
Bình luận (0)
* \(x^3-16x=0\)
\(\Rightarrow x\left(x^2-16\right)=0\)
\(\Rightarrow x\left(x^2-4^2\right)=0\)
\(\Rightarrow x\left(x-4\right)\left(x+4\right)=0\)
\(\Rightarrow\left[{}\begin{matrix}x=0\\x=4\\x=-4\end{matrix}\right.\)
Đúng 0
Bình luận (0)
*\(x^4-2x^3+10x^2-20x=0\)
\(\Rightarrow\left(x^4+10x^2\right)-\left(2x^3+20x\right)=0\)
\(\Rightarrow x^2\left(x^2+10\right)-2x\left(x^2+10\right)=0\)
\(\Rightarrow\left(x^2+10\right)\left(x^2-2x\right)=0\)
\(\Rightarrow\left[{}\begin{matrix}x=\varnothing\\x=2\end{matrix}\right.\)
Đúng 0
Bình luận (1)
Xem thêm câu trả lời