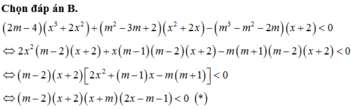Chứng minh phương trình sau vô nghiệm x4 - x3 +2x2 -x + 1 = 0
PA
Những câu hỏi liên quan
Bài 1: Giải phương trình:
a) ( x+1)2 (x+2) + ( x – 1)2 ( x- 2) = 12
b) x4 + 3x3 + 4x2 + 3x + 1 = 0
c) x5 – x4 + 3x3 + 3x2 –x + 1 = 0
Bài 2: Chứng minh rằng các phương trình sau vô nghiệm
a) x4 – x3 + 2x2 – x + 1 = 0
b) x4 + x3 + x2 + x + 1 = 0
c) x4 – 2x3 +4x2 – 3x +2 = 0
d) x6+ x5+ x4 + x3 + x2 + x + 1 = 0
1.
a/ \(\Leftrightarrow\left(x+1\right)\left(x^2+3x+2\right)+\left(x-1\right)\left(x^2-3x+2\right)-12=0\)
\(\Leftrightarrow\left(x+1\right)\left(x^2+2\right)+3x\left(x+1\right)-3x\left(x-1\right)+\left(x-1\right)\left(x^2+2\right)-12=0\)
\(\Leftrightarrow2x\left(x^2+2\right)+6x^2-12=0\)
\(\Leftrightarrow x^3+3x^2+2x-6=0\)
\(\Leftrightarrow\left(x-1\right)\left(x^2+4x+6\right)=0\Rightarrow x=1\)
b/ Nhận thấy \(x=0\) ko phải nghiệm, chia 2 vế cho \(x^2\)
\(x^2+\frac{1}{x^2}+3\left(x+\frac{1}{x}\right)+4=0\)
Đặt \(x+\frac{1}{x}=t\Rightarrow x^2+\frac{1}{x^2}=t^2-2\)
\(t^2-2+3t+4=0\Rightarrow t^2+3t+2=0\Rightarrow\left[{}\begin{matrix}t=-1\\t=-2\end{matrix}\right.\)
\(\Rightarrow\left[{}\begin{matrix}x+\frac{1}{x}=-1\\x+\frac{1}{x}=-2\end{matrix}\right.\) \(\Rightarrow\left[{}\begin{matrix}x^2+x+1=0\left(vn\right)\\x^2+2x+1=0\end{matrix}\right.\) \(\Rightarrow x=-1\)
1c/
\(\Leftrightarrow x^5+x^4-2x^4-2x^3+5x^3+5x^2-2x^2-2x+x+1=0\)
\(\Leftrightarrow x^4\left(x+1\right)-2x^3\left(x+1\right)+5x^2\left(x+1\right)-2x\left(x+1\right)+x+1=0\)
\(\Leftrightarrow\left(x+1\right)\left(x^4-2x^3+5x^2-2x+1\right)=0\)
\(\Leftrightarrow\left[{}\begin{matrix}x=-1\\x^4-2x^3+5x^2-2x+1=0\left(1\right)\end{matrix}\right.\)
\(\left(1\right)\Leftrightarrow x^4-2x^3+x^2+x^2-2x+1+3x^2=0\)
\(\Leftrightarrow\left(x^2-x\right)^2+\left(x-1\right)^2+3x^2=0\)
\(\Leftrightarrow\left\{{}\begin{matrix}x^2-x=0\\x-1=0\\x=0\end{matrix}\right.\) \(\Rightarrow\) không tồn tại x thỏa mãn
Vậy pt có nghiệm duy nhất \(x=-1\)
2.
a. \(x^4-x^3+x^2+x^2-x+1=0\)
\(\Leftrightarrow x^2\left(x^2-x+1\right)+x^2-x+1=0\)
\(\Leftrightarrow\left(x^2+1\right)\left(x^2-x+1\right)=0\)
\(\Leftrightarrow\left[{}\begin{matrix}x^2+1=0\left(vn\right)\\x^2-x+1=0\Leftrightarrow\left(x-\frac{1}{2}\right)^2+\frac{3}{4}=0\left(vn\right)\end{matrix}\right.\)
Vậy pt vô nghiệm
b.
\(x^4+x^3+x^2+x+1=0\)
\(\Leftrightarrow x\left(x^3+1\right)+x^3+1+x^2=0\)
\(\Leftrightarrow\left(x+1\right)\left(x^3+1\right)+x^2=0\)
\(\Leftrightarrow\left(x+1\right)^2\left(x^2-x+1\right)+x^2=0\)
Mà \(\left\{{}\begin{matrix}\left(x+1\right)^2\left(x^2-x+1\right)\ge0\\x^2\ge0\end{matrix}\right.\)
Nên dấu "=" xảy ra khi và chỉ khi: \(\left\{{}\begin{matrix}x+1=0\\x=0\end{matrix}\right.\) ko tồn tại x thỏa mãn
Xem thêm câu trả lời
chứng minh phương trình sau vô nghiệm:
x4-2x3+10x+30=0
Giải:
Tập xác định của phương trình
x\(\varepsilon\) (\(\infty\);\(\infty\)
cho x1, x2 là 2 nghiệm dương của phương trình ax^2+bx+c=0
chứng minh phương trình cx^2+ax+b=0 cũng có 2 nghiệm dương x3,x4 và x1+x2+x3+x4>4 ?
a) ax^2 + bx + c = 0
Để phương trình thỏa mãn điều kiện có 2 nghiệm dương phân biệt.
∆ > 0
=> b^2 - 4ac > 0
x1 + x2 = -b/a > 0
=> b và a trái dấu
x1.x2 = c/a > 0
=> c và a cùng dấu
Từ đó ta xét phương trình cx^2 + bx^2 + a = 0
∆ = b^2 - 4ac >0
x3 + x4 = -b/c, vì a và c cùng dấu mà b và a trái dấu nên b và c trái dấu , vì vậy -b/c >0
x3.x4 = a/c, vì a và c cùng dấu nên a/c > 0
=> phương trình cx^2 + cx + a có 2 nghiệm dương phân biệt x3 và x4
Vậy nếu phương trình ax^2 + bx + c = 0 có 2 nghiệm dương phân biệt thì phương trình cx^2 + bx + a = 0 cũng có 2 nghiệm dương phân biệt.
b) Ta có, vì x1, x2, x3, x4 không âm, dùng cô si.
x1 + x2 ≥ 2√( x1.x2 )
x3 + x4 ≥ 2√( x3x4 )
=> x1 + x2 + x3 + x4 ≥ 2[ √( x1.x2 ) + √( x3x4 ) ] (#)
Tiếp tục côsi cho 2 số không âm ta có
√( x1.x2 ) + √( x3x4 ) ≥ 2√[√( x1.x2 )( x3.x4 ) ] (##)
Theo a ta có
x1.x2 = c/a
x3.x4 = a/c
=> ( x1.x2 )( x3.x4 ) = 1
=> 2√[√( x1.x2 )( x3.x4 ) ] = 2
Từ (#) và (##) ta có
x1 + x2 + x3 + x4 ≥ 4
Đúng 0
Bình luận (0)
cho phương trình (x+1)(x+2)(x+3)(x+4)=m
biết rằng phương trình đã cho có 4 nghiệm phân biệt x1,x2,x3,x4x1,x2,x3,x4
chứng minh x1.x2.x3.x4=24−m
Gọi x1, x2 là nghiệm của phương trình x^2+2009x+1=0,
x3,x4 là nghiệm của phương trình x^2+2010x+1=0.
Tính giá trị biểu thức (x1+x3)(x2+x3)(x1-x4)(x2-x4)
Chứng minh đa thức x4+2x2+1 vô nghiệm
x4+2x2+1
Ta có :
x4 ≥ 0 ∀ x
x2 ≥ 0 ∀ x => 2x2 ≥ 0 ∀ x
=> x4+2x2+1 ≥ 1 >0
Suy ra đa thức trên vô nghiệm
Đúng 1
Bình luận (0)
Cho hai phương trình x2+2022x+1=0 (1) và x2+2023x+1 (2).Gọi x1,x2 là nghiệm của phương trình (1) ; x3,x4 là nghiệm của phương trình (2).Giá trị của biểu thức P=(x1+x3)(x2+x3)(x1-x4)(x2-x4) là
A.4045 B.-1 C.1 D.0
Gọi n là số các giá trị của tham số m để bất phương trình
(
2
m
-
4
)
(
x
3
+
2
x
2
)
+
(
m
2
-
3
m
+
2
)
-
(
m
3
–
m
2
-
2
m...
Đọc tiếp
Gọi n là số các giá trị của tham số m để bất phương trình ( 2 m - 4 ) ( x 3 + 2 x 2 ) + ( m 2 - 3 m + 2 ) - ( m 3 – m 2 - 2 m ) ( x + 2 ) < 0 vô nghiệm. Giá trị của n bằng
A. 5
B. 1
C. 4
D. 2
Tìm m để phương trình x^4-2(m+1)x^2+m+5=0 có 4 nghiệm thỏa mãn x1<x2<x3<x4;x1-x2=x2-x3=x3-x4
Đặt x2−2x+m=tx2−2x+m=t, phương trình trở thành t2−2t+m=xt2−2t+m=x
Ta có hệ {x2−2x+m=tt2−2t+m=x{x2−2x+m=tt2−2t+m=x
⇒(x−t)(x+t−1)=0⇒(x−t)(x+t−1)=0
⇔[x=tx=1−t⇔[x=tx=1−t
⇔[x=x2−2x+mx=1−x2+2x−m⇔[x=x2−2x+mx=1−x2+2x−m
⇔[m=−x2+3xm=−x2+x+1⇔[m=−x2+3xm=−x2+x+1
Phương trình hoành độ giao điểm của y=−x2+x+1y=−x2+x+1 và y=−x2+3xy=−x2+3x:
−x2+x+1=−x2+3x−x2+x+1=−x2+3x
⇔x=12⇒y=54⇔x=12⇒y=54
Đồ thị hàm số y=−x2+3xy=−x2+3x và y=−x2+x+1y=−x2+x+1:
Chứng minh phương trình sau vô nghiệm :
1+x+x^2+x^3+....+x^2020=0
Ta có:\(1+x+x^2+x^3+...+x^{2020}=0\)
\(\Leftrightarrow1+\left(x+x^2\right)+\left(x^3+x^4\right)+...+\left(x^{2019}+x^{2020}\right)=0\)
Mà \(x+x^2\ge0\forall x\)
\(x^3+x^4\ge0\forall x\)
........
\(x^{2019}+x^{2020}\ge0\forall x\)
\(\Leftrightarrow1+\left(x+x^2\right)+\left(x^3+x^4\right)+...+\left(x^{2019}+x^{2020}\right)\ge1\forall x\)
Theo bài ra:\(1+\left(x+x^2\right)+\left(x^3+x^4\right)+...+\left(x^{2019}+x^{2020}\right)=0\)
\(\Rightarrow\)Vô nghiệm