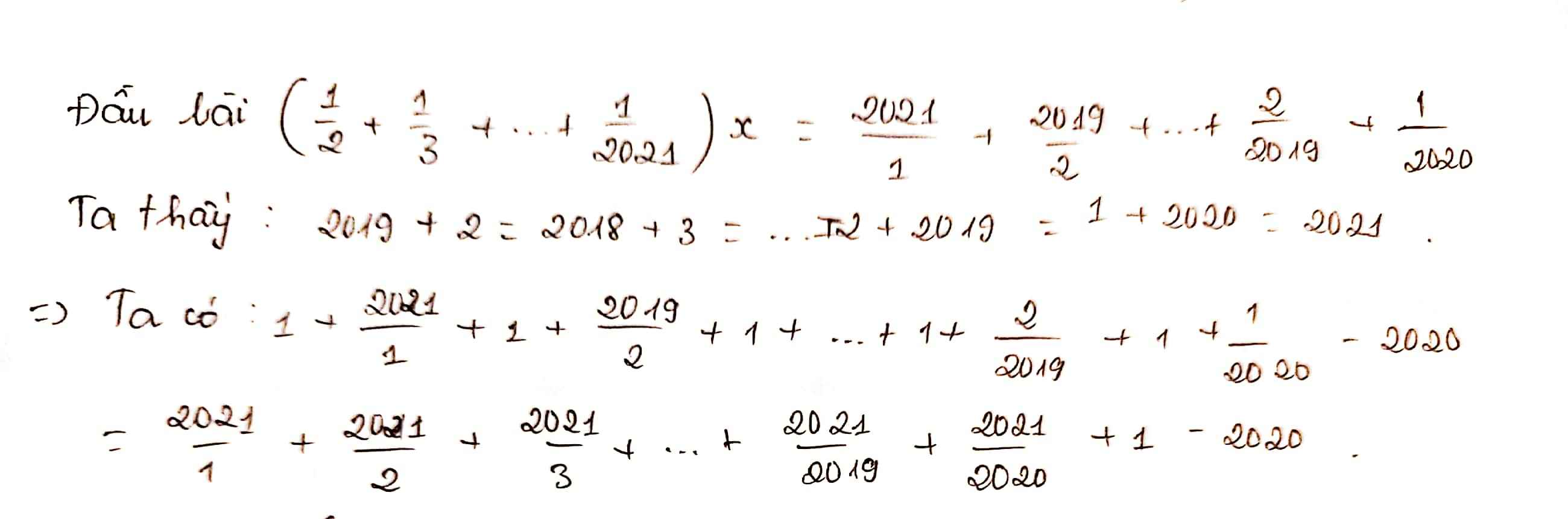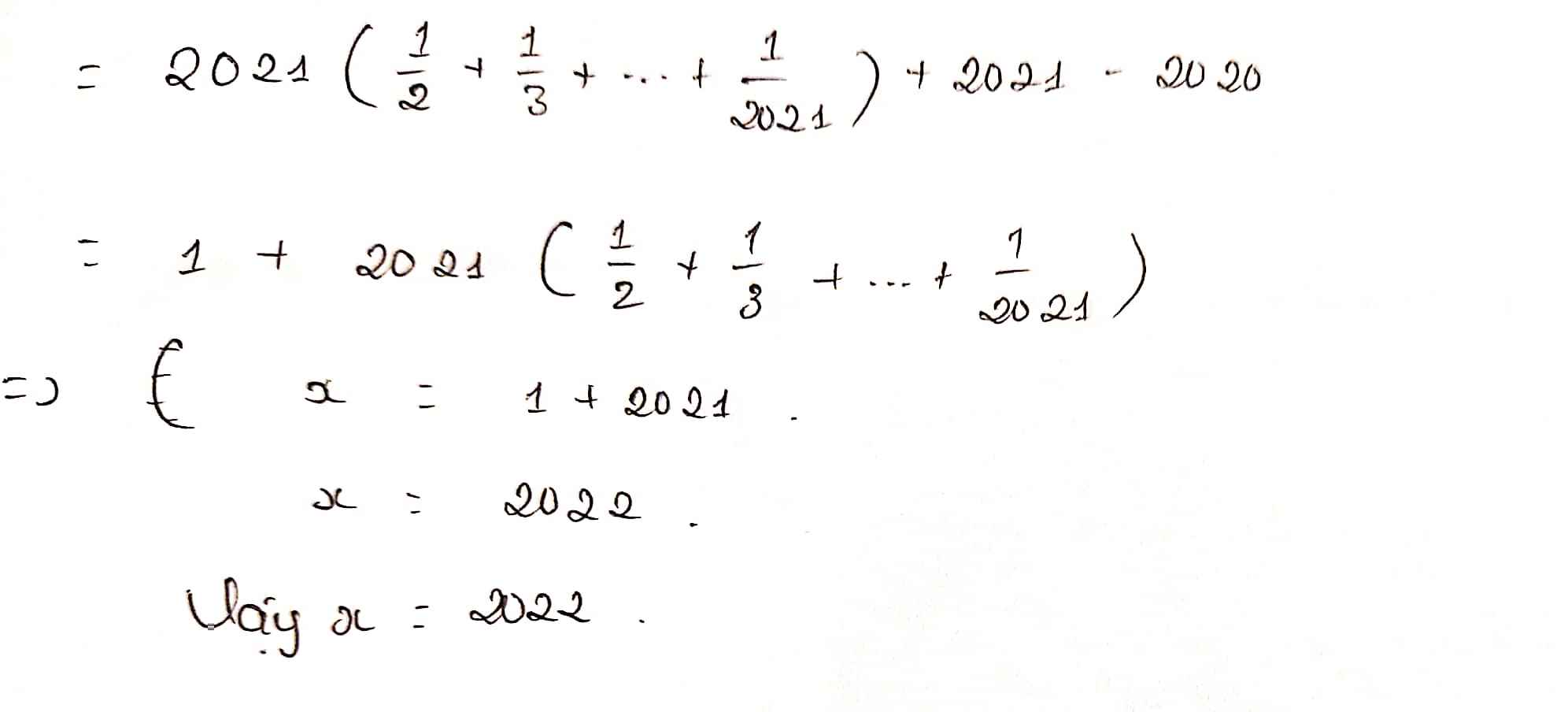tìm x biết :|x+1/2021|+|x+2/2021|+...+|x+2020/2021|=2021x
PK
Những câu hỏi liên quan
Tính giá trị của biểu thức A= x2021-2021x2020+2021x2019-2021x2018+....-2021x2+2021x-2021 khi x=2020
Ta có x = 2020
=> x + 1 = 2021
A = x2021 - 2021x2020 + .... + 2021x - 2021
= x2021 - (x + 1)x2020 + .... + (x + 1)x - (x + 1)
= x2021 - x2021 - x2020 + .... + x2 + x - x + 1
= 1
Vậy A = 1
Ta có : \(x=2020\Rightarrow x+1=2021\)
\(A=x^{2021}-\left(x+1\right)x^{2020}+\left(x+1\right)x^{2019}-\left(x+1\right)x^{2018}+...-\left(x+1\right)x^2+\left(x+1\right)x-2021\)
= x2021 - x2021 - x2020 + x2020 + x2019 - x2019 - x2018 + ... - x3 - x2 + x2 + x - 2021 = x - 2021
mà x = 2020 hay 2020 - 2021 = -1
Vậy với x = 2020 thì A = -1
Rút gọn:
a) A=(5-2x)2-4x(x-5)
b) B= (4-3x)(4+3x)+(3x+1)2
c) C= (x+1)3-x(x2+3x+3)
d) D=(2021x-2020)2-2(2021x-2020)(2020x-2021)+(2020x-2021)
a: \(A=\left(2x-5\right)^2-4x\left(x-5\right)\)
\(=4x^2-20x+25-4x^2+20x\)
=25
b: \(B=\left(4-3x\right)\left(4+3x\right)+\left(3x+1\right)^2\)
\(=16-9x^2+9x^2+6x+1\)
=6x+17
c: \(C=\left(x+1\right)^3-x\left(x^2+3x+3\right)\)
\(=x^3+3x^2+3x+1-x^3-3x^2-3x\)
=1
d: \(D=\left(2021x-2020\right)^2-2\left(2021x-2020\right)\left(2020x-2021\right)+\left(2020x-2021\right)^2\)
\(=\left(2021x-2020-2020x+2021\right)^2\)
\(=\left(x+1\right)^2\)
\(=x^2+2x+1\)
Đúng 0
Bình luận (0)
Cho x = 2020, tính giá trị:
P(x) = x^2021-2021x^2020+2021x^2019-2021x^2018+...+2021x-2020
x=2020 nên x+1=2021
\(P\left(x\right)=x^{2021}-x^{2020}\left(x+1\right)+x^{2019}\left(x+1\right)-....+x\left(x+1\right)-2020\)
\(=x^{2021}-x^{2021}-x^{2020}+x^{2020}-...+x^2+x-2020\)
=x-2020=0
Đúng 0
Bình luận (0)
tìm x biết:
2021-x+2021x.(1-2021x)=0
Tìm x biết:2021-x+2021x(1-2020x)=0
2021 - x + 2021(x - 2020x) = 0
<=> 2021 - x + 2021 - 4082420 = 0
<=> -x - 4082420 = 0
<=> x = -4082420
Đúng 1
Bình luận (1)
1 ) Tìm x biết : a) ( x - 1 ) ( 2x + 3 ) - 2x2 = 7
b) x2 - 2021x - x + 2021 = 0
a: \(\Leftrightarrow x-3=7\)
hay x=10
Đúng 0
Bình luận (0)
Tìm x biết:
( 1/2 + 1/3 + ... + 1/2021 ).x = 2021/1 +2019/2 + ... + 2/2019 + 1/2020
Tìm x biết:
( 1/2 + 1/3 + ... + 1/2021 ).x = 2021/1 +2019/2 + ... + 2/2019 + 1/2020
Với xx là số thực bất kì, mệnh đề nào sau đây sai?
A.
(2021x)2=(2021)x2.
B.
22021x=2021x2.
C.
(2021x)2=(2021)2x
D.
√2021x=(√2021)x