Tìm x biết :
x2 + 17x +72 =0
2x2 -7x +3
giải pt:
a) (x2-3x)(x2+7x+10)=216
b) (2x2-7x+3)(2x2+x-3)+9=0
a) \(\left(x^2-3x\right)\left(x^2+7x+10\right)=216\Rightarrow x\left(x-3\right)\left(x+2\right)\left(x+5\right)=216\)
\(\Rightarrow x\left(x+2\right)\left(x-3\right)\left(x+5\right)=216\Rightarrow\left(x^2+2x\right)\left(x^2+2x-15\right)=216\)
Đặt \(t=x^2+2x\Rightarrow\) pt trở thành \(t\left(t-15\right)=216\Rightarrow t^2-15t-216=0\)
\(\Rightarrow\left(t+9\right)\left(t-24\right)=0\Rightarrow\left[{}\begin{matrix}t=-9\\t=24\end{matrix}\right.\Rightarrow\left[{}\begin{matrix}x^2+2x=-9\\x^2+2x=24\end{matrix}\right.\)
\(TH_1:x^2+2x=-9\Rightarrow x^2+2x+9=0\Rightarrow\left(x+1\right)^2+8=0\) (vô lý)
\(TH_2:x^2+2x=24\Rightarrow x^2+2x-24=0\Rightarrow\left(x-4\right)\left(x+6\right)=0\)
\(\Rightarrow\left[{}\begin{matrix}x=4\\x=-6\end{matrix}\right.\)
b) \(\left(2x^2-7x+3\right)\left(2x^2+x-3\right)+9=0\)
\(\Rightarrow\left(x-3\right)\left(2x-1\right)\left(x-1\right)\left(2x+3\right)+9=0\)
\(\Rightarrow\left(x-3\right)\left(2x+3\right)\left(x-1\right)\left(2x-1\right)+9=0\)
\(\Rightarrow\left(2x^2-3x-9\right)\left(2x^2-3x+1\right)+9=0\)
Đặt \(t=2x^2-3x-9\Rightarrow\) pt trở thành \(t\left(t+10\right)+9=0\)
\(\Rightarrow t^2+10t+9=0\Rightarrow\left(t+1\right)\left(t+9\right)=0\Rightarrow\left[{}\begin{matrix}t=-1\\t=-9\end{matrix}\right.\)
\(TH_1:t=-1\Rightarrow2x^2-3x-9=-1\Rightarrow2x^2-3x-8=0\)
\(\Delta=\left(-3\right)^2-4\left(-8\right).2=73\Rightarrow\left[{}\begin{matrix}x=\dfrac{-b-\sqrt{\Delta}}{2a}=\dfrac{3-\sqrt{73}}{4}\\x=\dfrac{-b+\sqrt{\Delta}}{2a}=\dfrac{3+\sqrt{73}}{4}\end{matrix}\right.\)
\(TH_2:t=-9\Rightarrow2x^2-3x-9=-9\Rightarrow2x^2-3x=0\Rightarrow x\left(2x-3\right)=0\)
\(\Rightarrow\left[{}\begin{matrix}x=0\\x=\dfrac{3}{2}\end{matrix}\right.\)
Tìm x biết
a,(x+4)(3x-5)=0
b, x2-2x+10x-20=0
c, 2x2+7x+3=0
GIÚP MÌNH VỚI NHA
Với mỗi phương trình sau, đã biết một nghiệm (ghi kèm theo), hãy tìm nghiệm kia:
a ) 12 x 2 − 8 x + 1 = 0 ; x 1 = 1 2 b ) 2 x 2 − 7 x − 39 = 0 ; x 1 = − 3 c ) x 2 + x − 2 + 2 = 0 ; x 1 = − 2 d ) x 2 − 2 m x + m − 1 = 0 ; x 1 = 2
Theo định lý Vi-et ta có: phương trình
a
x
2
+
b
x
+
c
= 0 có hai nghiệm
x
1
;
x
2
thì: 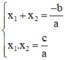
Ta sử dụng một trong hai biểu thức trên để tìm nghiệm còn lại.
Ở bài giải dưới đây ta sẽ sử dụng điều kiện: 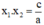
(Các bạn có thể làm cách 2 sử dụng điều kiện 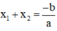 ).
).
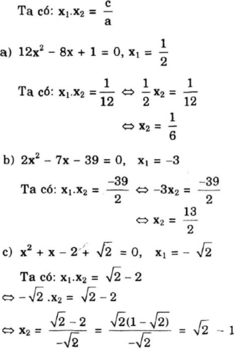
d) x 2 - 2 m x + m - 1 = 0 ( 1 )
Vì x 1 = 2 là một nghiệm của pt (1) nên:
2 2 - 2 m . 2 + m - 1 = 0
⇔ 4- 4 m+ m – 1 = 0
⇔ 3- 3m = 0
⇔ m = 1
Khi m = 1 ta có: x 1 . x 2 = m - 1 (hệ thức Vi-ét)
⇔ 2 . x 2 = 0 ( v ì x 1 = 2 và m = 1)
⇔ x 2 = 0
Cho E = {x ≤ Z||x| ≤ 5}, F = {x ∈ N ||x| ≤ 5} và
B = {x ∈ Z|(x – 2)(x + 1)(2x2 – x – 3) = 0}. Chứng minh A ⊂ E và B⊂E
Cho A = {x ∈ R | x2+ x – 12 = 0 và 2x2 – 7x + 3 = 0}
B = {x ∈ Z | 3x2 – 13x + 12 =0 hoặc x2 – 3x = 0}
Cho hàm số y = f x = 2 x 2 − 7 x + 6 x − 2 k h i x < 2 a + 1 − x 2 + x k h i x ≥ 2 . Biết a là giá trị để hàm số f(x) liên tục tại x 0 = 2 , tìm nghiệm nguyên của bất phương trình − x 2 + a x + 7 4 > 0 .
A. 1
B. 4
C. 3
D. 2
Đáp án D
Ta có lim x → 2 − f x = lim x → 2 − 2 x 2 − 7 x + 6 x − 2 = lim x → 2 − 2 x 2 − 7 x + 6 x − 2 = lim x → 2 − − 2 x − 3 = − 1
Và lim x → 2 − f x = lim x → 2 − a + 1 − x 2 + x = a − 1 4 ; f 2 = a − 1 4 .
Theo bài ra, ta có lim x → 2 + f x = lim x → 2 − f x = f 2 ⇒ a = − 3 4
Do đó, bất phương trình − x 2 + a x + 7 4 > 0 ⇔ − x 2 − 3 4 x + 7 4 > 0 ⇔ − 7 4 < x < 1.
Giair phương trình
1) 2x2-3x-2=0 7) (2x2-3x-4)2=(x2-x)2
2) 4x2-7x-2=0 8) \(\dfrac{2}{x+1}-\dfrac{3}{x+2}=\dfrac{1}{3x+3}\)
3) 4x2+5x-6=0 9) \(\dfrac{x}{x-3}=\dfrac{1}{x+2}\)
4) 4x2+5x-9=0 10) \(\dfrac{4}{2x-3}-\dfrac{7}{3x-5}=0\)
5) 5x2-18x-8=0 11) \(\dfrac{7}{x+2}+\dfrac{2}{x+3}=\dfrac{1}{x^2+5x+6}\)
6) (3x2+2x+4)2=(x2-4)2 12) \(\dfrac{4}{x-2}+\dfrac{x}{x+1}=\dfrac{x^2-2}{x^2-x-2}\)
Giúp em vs em đag cần câu tl gấp![]() em c.ơn trước
em c.ơn trước
giải phương trình làm dell gì, lớp 8 mà
Số?
a)12 x 4= ? x 12 (17x5)x2=17x(5x?)
106 x 3 =3 x ? 86x2x5=86 x(2x?)
b)7x ? =7 519 x ? =0 ? x0=0
432 x? =432 1x ? = 0 ?x1 =3456
a)
12 × 4 = 4 × 12
106 × 3 = 3 × 106
(17 × 5) × 2 = 17 × (5 × 2)
86 × 2 × 5 = 86 × (2 × 5)
b)
7 × 1 = 7
432 × 1 = 432
519 × 0 = 0
1 × 0 = 0
2 × 0 = 0
3 456 × 1 = 3 456
Thực hiện phép tính:
a)2x(3x2 - 5x + 3) b)-2x2(x2 + 5x - 3) c)-1/2x2(2x3 - 4x + 3)
d) (2x - 1)(x2 +5- 4) c) 7x(x - 4) - (7x + 3)(2x2 - x + 4).
a: \(=6x^3-10x^2+6x\)
b: \(=-2x^4-10x^3+6x^2\)
c: \(=-x^5+2x^3-\dfrac{3}{2}x^2\)
d: \(=2x^3+10x^2-8x-x^2-5x+4=2x^3+9x^2-13x+4\)
tìm x
x2−6x+5=0x2−6x+5=0
2x2+7x+9=02x2+7x+9=0
4x2−7x+3=04x2−7x+3=0
2(x+5)=x2+5x
\(x^2-6x+5=0\)
\(\Leftrightarrow x^2-x-5x+5=0\)
\(\Leftrightarrow x\left(x-1\right)-5\left(x-1\right)=0\)
\(\Leftrightarrow\left(x-1\right)\left(x-5\right)=0\)
\(\Leftrightarrow\orbr{\begin{cases}x-1=0\\x-5=0\end{cases}}\)
\(\Leftrightarrow\orbr{\begin{cases}x=1\\x=5\end{cases}}\)
\(2x^2+7x+9=0\)
Đề sai??
\(4x^2-7x+3=0\)
\(\Leftrightarrow4x^2-4x-3x+3=0\)
\(\Leftrightarrow4x\left(x-1\right)-3\left(x-1\right)=0\)
\(\Leftrightarrow\left(x-1\right)\left(4x-3\right)=0\)
\(\Leftrightarrow\orbr{\begin{cases}x-1=0\\4x-3=0\end{cases}}\Leftrightarrow\orbr{\begin{cases}x=1\\x=\frac{3}{4}\end{cases}}\)
\(2\left(x+5\right)=x^2+5x\)
\(\Leftrightarrow2x+10=x^2+5x\)
\(\Leftrightarrow x^2+5x-2x-10=0\)
\(\Leftrightarrow x^2+3x-10=0\)
\(\Leftrightarrow x^2+5x-2x-10=0\)
\(\Leftrightarrow x\left(x+5\right)-2\left(x+5\right)=0\)
\(\Leftrightarrow\left(x+5\right)\left(x-2\right)=0\)
\(\Leftrightarrow\orbr{\begin{cases}x+5=0\\x-2=0\end{cases}\Leftrightarrow}\orbr{\begin{cases}x=-5\\x=2\end{cases}}\)