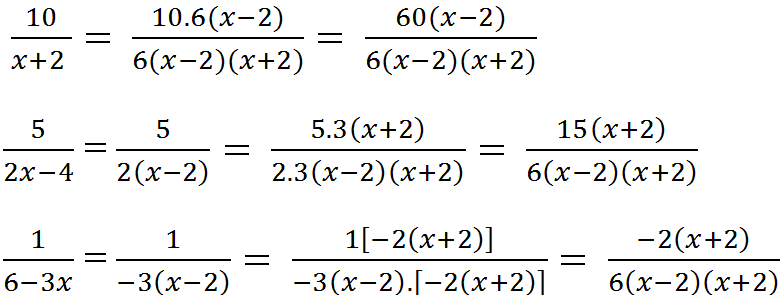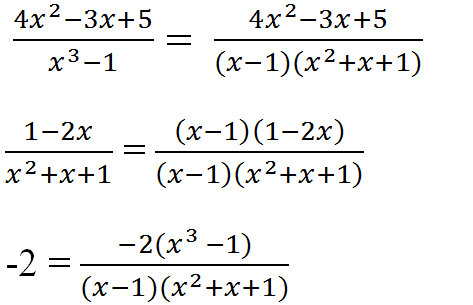Mẫu chung của các phân thức \(\dfrac{1-4x}{2x^2+6x};\dfrac{4-x}{x^2-9};\dfrac{2x+1}{2}\)là
DH
Những câu hỏi liên quan
Mẫu chung của các phân thức \(\dfrac{1-4x}{2x^2+6x};\dfrac{4-x}{x^2-9};\dfrac{2x+1}{2}\)là
\(\frac{1-4x}{2x^2+6x}\)= \(\frac{1-4x}{2x\left(x+3\right)}\)
\(\frac{4-x}{x^2-9}\)= \(\frac{4-x}{\left(x-3\right)\left(x+3\right)}\)
=> MTC: 2x(x-3)(x+3)
Đúng 0
Bình luận (0)
quy đồng mẫu thức của các phân thức
\(\dfrac{1}{x+2};\dfrac{-3x}{x-2};\dfrac{3}{x^2-4x+4}\)
\(\dfrac{-1}{2x+2};\dfrac{3}{2-2x};\dfrac{5}{4x^2+4x+1}\)
cho mình hỏi là giữa khác phân số với nhua là phải có dấu như là công, trừ, nhân hay chia chứ?
Đúng 1
Bình luận (0)
Quy đồng mẫu thức các phân thức sau (có thể áp dụng quy tắc đổi dấu với một phân thức để tìm mẫu thức chung thuận tiện hơn)
a) \(\dfrac{4x^2-3x+5}{x^3-1},\dfrac{1-2x}{x^2+x+1},-2\)
b) \(\dfrac{10}{x+2},\dfrac{5}{2x-4},\dfrac{1}{6-3x}\)
a) Tìm MTC: x3 – 1 = (x – 1)(x2 + x + 1)
Nên MTC = (x – 1)(x2 + x + 1)
Nhân tử phụ:
(x3 – 1) : (x3 – 1) = 1
(x – 1)(x2 + x + 1) : (x2 + x + 1) = x – 1
(x – 1)(x2+ x + 1) : 1 = (x – 1)(x2 + x + 1)
Qui đồng:
b) Tìm MTC: x + 2
2x – 4 = 2(x – 2)
6 – 3x = 3(2 – x)
MTC = 6(x – 2)(x + 2)
Nhân tử phụ:
6(x – 2)(x + 2) : (x + 2) = 6(x – 2)
6(x – 2)(x + 2) : 2(x – 2) = 3(x + 2)
6(x – 2)(x + 2) : -3(x – 2) = -2(x + 2)
Qui đồng:
Đúng 0
Bình luận (1)
Câu 1 Quy đồng mẫu thức của các phân thức sau::(2 điểm)
a/ \(\dfrac{3}{4x^3y^2}\) và \(\dfrac{2}{3xy^3}\) b/ \(\dfrac{5}{x^2-6x+9}\) và \(\dfrac{3}{x^2-3x}\)
a) MTC: \(12x^3y^3\)
\(\dfrac{3}{4x^3y^2}=\dfrac{3\cdot3y}{4x^3y^2\cdot3y}=\dfrac{9y}{12x^3y^3}\)
\(\dfrac{2}{3xy^3}=\dfrac{2\cdot4x^2}{3xy^3\cdot4x^2}=\dfrac{8x^2}{12x^3y^3}\)
b) MTC: \(x\left(x-3\right)^2\)
\(\dfrac{5}{x^2-6x+9}=\dfrac{5}{\left(x-3\right)^2}=\dfrac{5x}{x\left(x-3\right)^2}\)
\(\dfrac{3}{x^2-3x}=\dfrac{3}{x\left(x-3\right)}=\dfrac{3\left(x-3\right)}{x\left(x-3\right)^2}=\dfrac{3x-9}{x\left(x-3\right)^2}\)
Đúng 1
Bình luận (0)
Quy đồng mẫu thức các phân thức :
a) dfrac{7x-1}{2x^2+6x};dfrac{5-3x}{x^2-9}
b) dfrac{x+1}{x-x^2};dfrac{x+2}{2-4x+2x^2}
c) dfrac{4x^2-3x+5}{x^3-1};dfrac{2x}{x^2+x+1};dfrac{6}{x-1}
d) dfrac{7}{5x};dfrac{4}{x-2y};dfrac{x-y}{8y^2-2x^2}
e) dfrac{5x^2}{x^3+6x^2+12x+8};dfrac{4x}{x^2+4x+4};dfrac{3}{2x+4}
Đọc tiếp
Quy đồng mẫu thức các phân thức :
a) \(\dfrac{7x-1}{2x^2+6x};\dfrac{5-3x}{x^2-9}\)
b) \(\dfrac{x+1}{x-x^2};\dfrac{x+2}{2-4x+2x^2}\)
c) \(\dfrac{4x^2-3x+5}{x^3-1};\dfrac{2x}{x^2+x+1};\dfrac{6}{x-1}\)
d) \(\dfrac{7}{5x};\dfrac{4}{x-2y};\dfrac{x-y}{8y^2-2x^2}\)
e) \(\dfrac{5x^2}{x^3+6x^2+12x+8};\dfrac{4x}{x^2+4x+4};\dfrac{3}{2x+4}\)
Cộng các phân thức khác mẫu thức :
a) \(\dfrac{5}{6x^2y}+\dfrac{7}{12xy^2}+\dfrac{11}{18xy}\)
b) \(\dfrac{4x+2}{15x^3y}+\dfrac{5y-3}{9x^2y}+\dfrac{x+1}{5xy^3}\)
c) \(\dfrac{3}{2x}+\dfrac{3x-3}{2x-1}+\dfrac{2x^2+1}{4x^2-2x}\)
d) \(\dfrac{x^3+2x}{x^3+1}+\dfrac{2x}{x^2-x+1}+\dfrac{1}{x+1}\)
Quy đồng mẫu các phân thức sau:
a)\(\dfrac{7x-1}{2x^2+6x};\dfrac{5-3x}{x^2-9}\)
\(a,\dfrac{7x-1}{2x^2+6x}=\dfrac{\left(7x-1\right)\left(x-3\right)}{2x\left(x+3\right)\left(x-3\right)}=\dfrac{7x^2-22x+3}{2x\left(x-3\right)\left(x+3\right)}\\ \dfrac{5-3x}{x^2-9}=\dfrac{2x\left(5-3x\right)}{2x\left(x-3\right)\left(x+3\right)}=\dfrac{10x-6x^2}{2x\left(x-3\right)\left(x+3\right)}\)
Đúng 5
Bình luận (0)
tìm điều kiện để các phân thức sau có nghĩa và tìm mẫu chung của chúng:
a,\(\dfrac{5}{2x-4};\dfrac{4}{3x-9};\dfrac{7}{50-25x}\)
b,\(\dfrac{3}{2x+6};\dfrac{x-2}{x^2+6x+9}\)
c,\(\dfrac{x^4+1}{x^2-1};x^2+1\)
Cộng các phân thức cùng mẫu thức :
a) \(\dfrac{1-2x}{6x^3y}+\dfrac{3+2y}{6x^3y}+\dfrac{2y-4}{6x^3y}\)
b) \(\dfrac{x^2-2}{x\left(x-1\right)^2}+\dfrac{2-x}{x\left(x-1\right)^2}\)
c) \(\dfrac{3x+1}{x^2-3x+1}+\dfrac{x^6-6x}{x^2-3x+1}\)
d) \(\dfrac{x^2+38x+4}{2x^2+17x+1}+\dfrac{3x^2-4x-2}{2x^2+17x+1}\)
a: \(=\dfrac{1-2x+3+2y+2y-4}{6x^3y}=\dfrac{-2x+4y}{6x^3y}=\dfrac{-2\left(x-2y\right)}{6x^3y}=\dfrac{-x+2y}{3x^3y}\)
b: \(=\dfrac{x^2-2+2-x}{x\left(x-1\right)^2}=\dfrac{x\left(x-1\right)}{x\left(x-1\right)^2}=\dfrac{1}{x-1}\)
c: \(=\dfrac{3x+1+x^6-3x}{x^2-3x+1}\)
\(=\dfrac{x^6+1}{x^2-3x+1}\)
d: \(=\dfrac{x^2+38x+4+3x^2-4x-2}{2x^2+17x+1}\)
\(=\dfrac{4x^2+34x+2}{2x^2+17x+1}=2\)
Đúng 0
Bình luận (0)