tính hộ mình vs ạ ! 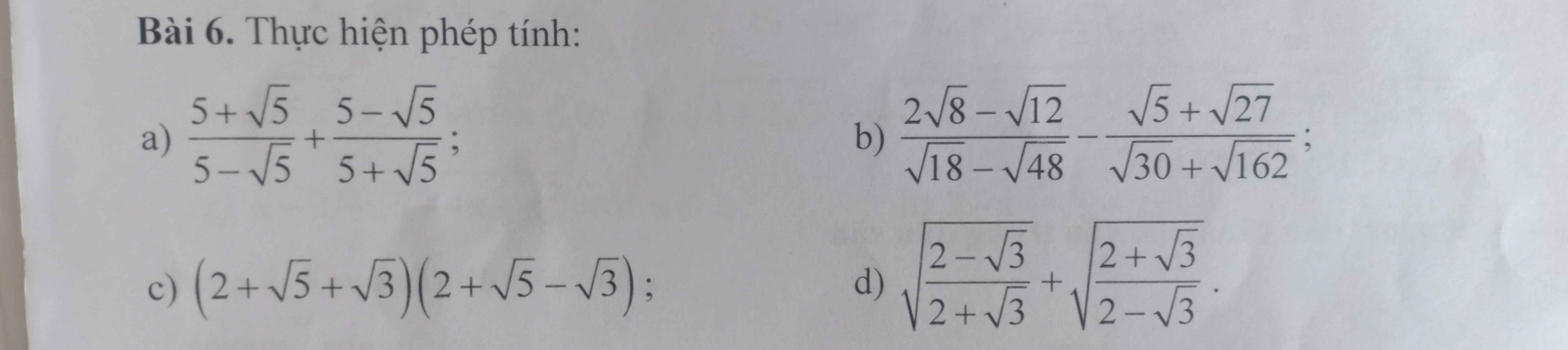
H24
Những câu hỏi liên quan
tính hộ mình vs ạ !
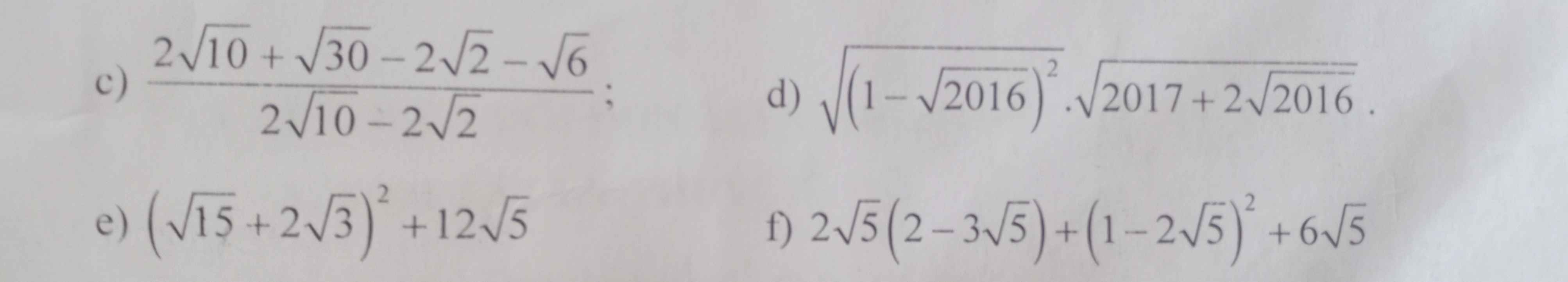
c) \(\dfrac{2\sqrt{10}+\sqrt{30}-2\sqrt{2}-\sqrt{6}}{2\sqrt{10}-2\sqrt{2}}=1+\dfrac{\sqrt{30}-\sqrt{6}}{2\sqrt{10}-2\sqrt{2}}=1+\dfrac{\sqrt{6}\left(\sqrt{5}-1\right)}{2\sqrt{2}\left(\sqrt{5}-1\right)}\)
\(=1+\dfrac{\sqrt{6}}{2\sqrt{2}}=1+\dfrac{\sqrt{3}}{2}=\dfrac{2+\sqrt{3}}{2}\)
d) \(\sqrt{\left(1-\sqrt{2016}\right)^2}.\sqrt{2017+2\sqrt{2016}}=\left|1-\sqrt{2016}\right|\sqrt{\left(\sqrt{2016}+1\right)^2}\)
\(=\left(\sqrt{2016}+1\right)\left(\sqrt{2016}-1\right)=2015\)
e) \(\left(\sqrt{15}+2\sqrt{3}\right)^2+12\sqrt{5}=27+12\sqrt{5}+12\sqrt{5}=27+24\sqrt{5}\)
f) \(2\sqrt{5}\left(2-3\sqrt{5}\right)+\left(1-2\sqrt{5}\right)^2+6\sqrt{5}\)
\(=4\sqrt{5}-30+21-4\sqrt{5}+6\sqrt{5}=6\sqrt{5}-9\)
Đúng 1
Bình luận (0)
Tính hộ mình vs ạ !
\(2\sqrt{5}\left(2-3\sqrt{5}\right)+\left(1-2\sqrt{5}\right)^2+6\sqrt{5}=4\sqrt{5}-30+20+1-4\sqrt{5}+6\sqrt{5}\)
\(=6\sqrt{5}-9\)
Đúng 1
Bình luận (0)
 giải hộ mình mấy bài này vs ạ !
giải hộ mình mấy bài này vs ạ !
giải hộ mình mấy bài này vs ạ !
Bài 5 hình 1: (tự vẽ hình nhé bạn)
a) Xét ΔABD và ΔACB ta có:
\(\widehat{BAD}\)= \(\widehat{BAC}\) (góc chung)
\(\widehat{ABD}\)= \(\widehat{ACB}\) (gt)
=> ΔABD ~ ΔACB (g-g)
=> \(\dfrac{AB}{AC}\) = \(\dfrac{BD}{CB}\) = \(\dfrac{AD}{AB}\) (tsđd)
b) Ta có: \(\dfrac{AB}{AC}\) = \(\dfrac{AD}{AB}\) (cm a)
=> \(AB^2\) = AD.AC
=> \(2^2\) = AD.4
=> AD = 1 (cm)
Ta có: AC = AD + DC (D thuộc AC)
=> 4 = 1 + DC
=> DC = 3 (cm)
c) Xét ΔABH và ΔADE ta có:
\(\widehat{AHB}\) = \(\widehat{AED}\) (=\(90^0\))
\(\widehat{ADB}\) = \(\widehat{ABH}\) (ΔABD ~ ΔACB)
=> ΔABH ~ ΔADE
=> \(\dfrac{AB}{AD}\) = \(\dfrac{AH}{AE}\) = \(\dfrac{BH}{DE}\) (tsdd)
Ta có: \(\dfrac{S_{ABH}}{S_{ADE}}\) = \(\left(\dfrac{AB}{AD}\right)^2\)= \(\left(\dfrac{2}{1}\right)^2\)= 4
=> đpcm
Đúng 2
Bình luận (0)
Tiếp bài 5 hình 2 (tự vẽ hình)
a) Xét ΔABC vuông tại A ta có:
\(BC^2\) = \(AB^2\) + \(AC^2\)
\(BC^2\) = \(21^2\) + \(28^2\)
BC = 35 (cm)
b) Xét ΔABC và ΔHBA ta có:
\(\widehat{BAC}\) = \(\widehat{AHB}\) ( =\(90^0\))
\(\widehat{ABC}\) = \(\widehat{ABH}\) (góc chung)
=> ΔABC ~ ΔHBA (g-g)
=> \(\dfrac{AB}{BH}\) = \(\dfrac{BC}{AB}\) (tsdd)
=> \(AB^2\) = BH.BC
=> \(21^2\) = 35.BH
=> BH = 12,6 (cm)
c) Xét ΔABC ta có:
BD là đường p/g (gt)
=> \(\dfrac{AD}{DC}\) = \(\dfrac{AB}{BC}\) (t/c đường p/g)
Xét ΔABH ta có:
BE là đường p/g (gt)
=> \(\dfrac{HE}{AE}\) = \(\dfrac{BH}{AB}\) (t/c đường p/g)
Mà: \(\dfrac{AB}{BC}\) = \(\dfrac{BH}{AB}\) (cm b)
=> đpcm
d) Ta có: \(\left\{{}\begin{matrix}\widehat{HBE}+\widehat{BEH}=90^0\\\widehat{ABD}+\widehat{ADB=90^0}\\\widehat{HBE}=\widehat{ABD}\end{matrix}\right.\)
=> \(\widehat{BEH}=\widehat{ADB}\)
Mà \(\widehat{BEH}=\widehat{AED}\) (2 góc dd)
Nên \(\widehat{ADB}=\widehat{AED}\)
=> đpcm
Đúng 2
Bình luận (0)
 giải hộ mình vs ạ mình cần gấp ạ
giải hộ mình vs ạ mình cần gấp ạ
1)x>=0
2)v8+5v2-v32+6v1/2=2v2+5v2-4v2+3v2=9v2
3)vx+1=2
x+1=4=>x=3
Đúng 0
Bình luận (0)
\(\dfrac{10+2\sqrt{10}}{\sqrt{5}+\sqrt{2}}+\dfrac{8}{1-\sqrt{5}}\)
Tính hộ mình vs ạ
\(=\dfrac{2\sqrt{5}\left(\sqrt{5}+\sqrt{2}\right)}{\sqrt{5}+\sqrt{2}}-\dfrac{8\left(\sqrt{5}+1\right)}{4}\\ =2\sqrt{5}-2\sqrt{5}-2=-2\)
Đúng 1
Bình luận (0)
Hộ mình câu 1b vs 3b vs ạ

giải hộ mình vs ạ!!! mình cảm ơn
Bài 4:
a: Thay x=36 vào A, ta được:
\(A=\dfrac{6+3}{6-2}=\dfrac{9}{4}\)
b: Ta có: \(B=\dfrac{1}{\sqrt{x}+2}+\dfrac{\sqrt{x}}{\sqrt{x}-2}+\dfrac{2}{x-4}\)
\(=\dfrac{\sqrt{x}-2+x+2\sqrt{x}+2}{\left(\sqrt{x}+2\right)\left(\sqrt{x}-2\right)}\)
\(=\dfrac{x+3\sqrt{x}}{x-4}\)
Đúng 0
Bình luận (0)
Giải hộ mình vs ạ !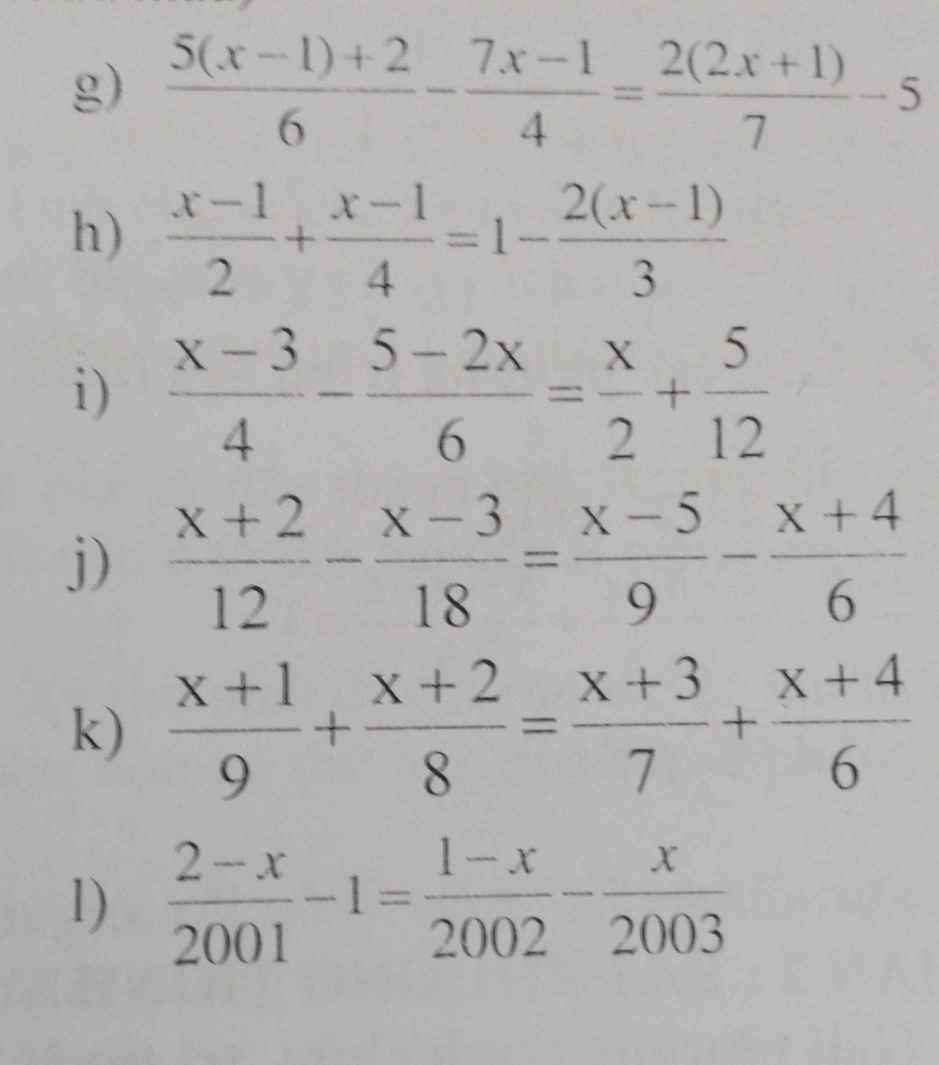
Giải hộ mình vs ạ !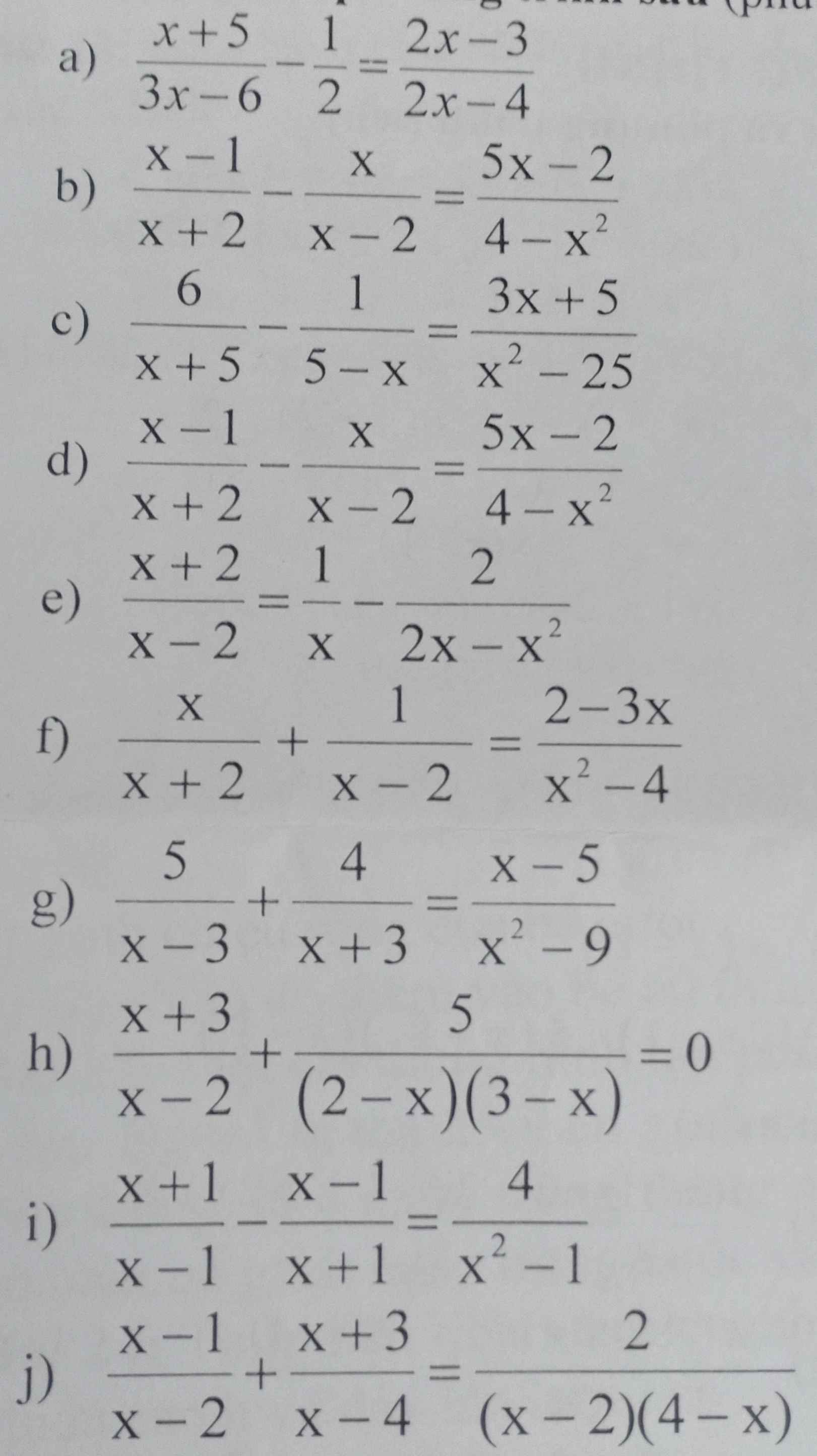
a) \(\dfrac{x+5}{3x-6}-\dfrac{1}{2}=\dfrac{2x-3}{2x-4}\) (1)
ĐKXĐ: x≠ 2
(1) ⇔ \(\dfrac{2x-10}{6\left(x-2\right)}-\dfrac{3x-6}{6\left(x-2\right)}=\dfrac{6x-9}{6\left(x-2\right)}\)
⇒ 2x - 10 - 3x + 6 = 6x - 9
⇔ -7x = -5
⇔ x = \(\dfrac{5}{7}\) (TMĐKXĐ)
Vậy S=\(\left\{\dfrac{5}{7}\right\}\)
b)\(\dfrac{x-1}{x+2}-\dfrac{x}{x-2}=\dfrac{5x-2}{4-x^2}\) (2)
ĐKXĐ: x≠ \(\pm2\)
(2) ⇒ x2 - 3x +2 - x2 - 2x = 5x - 2
⇔ -10x = -4
⇔ x = \(\dfrac{2}{5}\) (TMĐKXĐ)
Vậy S= \(\left\{\dfrac{2}{5}\right\}\)
c) \(\dfrac{6}{x+5}-\dfrac{1}{5-x}=\dfrac{3x+5}{x^2-25}\) (3)
ĐKXĐ: x ≠ \(\pm5\)
(3) ⇒ 6x - 30 -x +5 = 3x + 5
⇔ 2x = 30
⇔ x = 15 (TMĐKXĐ)
Vậy S= \(\left\{15\right\}\)
Đúng 0
Bình luận (0)
d) ĐKXĐ: x≠ \(\pm2\)
⇒ x2 - 3x + 2 - x2 - 2x = 2 - 5x
⇔ 0x = 0 (TMĐKXĐ)
Vậy PT có vô số nghiệm
e) ĐKXĐ: x≠ 0; x≠ 2
⇒ x2 + 2x = x - 2 + 2
⇔ x2 + x = 0
⇔ x(x + 1) = 0
⇔\(\left[{}\begin{matrix}x=0\\x+1=0\end{matrix}\right.\) ⇔ \(\left[{}\begin{matrix}x=0\left(KTMĐKXĐ\right)\\x=-1\left(TMĐKXĐ\right)\end{matrix}\right.\)
Vậy S = \(\left\{-1\right\}\)
f) ĐKXĐ: x≠ \(\pm2\)
⇒ x2 - 2x + x + 2 = 2 - 3x
⇔ x2 + 4x = 0
⇔ x(x+4) = 0
⇔\(\left[{}\begin{matrix}x=0\\x+4=0\end{matrix}\right.\) ⇔ \(\left[{}\begin{matrix}x=0\left(TMĐKXĐ\right)\\x=-4\left(TMĐKXĐ\right)\end{matrix}\right.\)\(\)
Vậy S=\(\left\{-4;0\right\}\)
Đúng 0
Bình luận (0)







