Cho S=\(\frac{2}{3-\sqrt{x}}\)với x> hoặc =0, x #4. Tìm x để S= 1
BL
Những câu hỏi liên quan
rút gọn biểu thức với lớn hơn hoặc bằng 0: A=\(\left(1-\frac{1}{\sqrt{x+1}}\right)\left(x+\sqrt{x}\right)\)
P=\(\left(\frac{3}{x-\sqrt{x-2}}+\frac{1}{\sqrt{x+1}}\right)\left(\sqrt{x-2}\right)\) với x lớn hơn hoặc bằng 0 và x khác 4
tìm GTLN của biểu thức:M=\(\left(\frac{2x+3\sqrt{x}}{2x+5\sqrt{x}+3}+\frac{2}{\sqrt{x}+1}\right):\frac{\sqrt{x}+2}{\sqrt{x}+2018}\)với x lớn hơn hoặc bàng 0
\(M=\left[\frac{\sqrt{x}\left(2\sqrt{x}+3\right)}{2x+2\sqrt{x}+3\sqrt{x}+3}+\frac{2}{\sqrt{x}+1}\right].\frac{\sqrt{x}+2018}{\sqrt{x}+2}\)
\(=\left[\frac{\sqrt{x}\left(2\sqrt{x}+3\right)}{\left(\sqrt{x}+1\right)\left(2\sqrt{x}+3\right)}+\frac{2}{\sqrt{x}+1}\right].\frac{\sqrt{x}+2018}{\sqrt{x}+2}\)
\(=\frac{\sqrt{x}+2}{\sqrt{x}+1}.\frac{\sqrt{x}+2018}{\sqrt{x}+2}\)
\(=\frac{\sqrt{x}+2018}{\sqrt{x}+1}\)
Đúng 0
Bình luận (0)
\(\frac{\sqrt{x}+2018}{\sqrt{x}+1}=1+\frac{2017}{\sqrt{x}+1}\le2018\)
Dấu "=" xảy ra \(\Leftrightarrow\)
...
Đúng 0
Bình luận (0)
rút gọn biểu thức với lớn hơn hoặc bằng 0: A=\(\left(1-\frac{1}{\sqrt{x+1}}\right)\left(x+\sqrt{x}\right)\)
P=\(\left(\frac{3}{x-\sqrt{x-2}}+\frac{1}{\sqrt{x+1}}\right)\left(\sqrt{x-2}\right)\) với x lớn hơn hoặc bằng 0 và x khác 4
Toán lớp 9
CHO BIỂU THỨC
B=\(\frac{1}{3-\sqrt{x}}+\frac{\sqrt{x}}{3+\sqrt{x}}-\frac{x+9}{x-9}\)
{với x khác 9 ;x lớn hơn hoặc bằng 0}
\(ĐKXĐ:\hept{\begin{cases}x\ne9\\x\ge0\end{cases}}\)
\(B=\frac{1}{3-\sqrt{x}}+\frac{\sqrt{x}}{3+\sqrt{x}}-\frac{x+9}{x-9}\)
\(\Leftrightarrow B=\frac{3+\sqrt{x}}{9-x}+\frac{\sqrt{x}\left(3-\sqrt{x}\right)}{9-x}+\frac{x+9}{9-x}\)
\(\Leftrightarrow B=\frac{3+\sqrt{x}+3\sqrt{x}-x+x+9}{9-x}\)
\(\Leftrightarrow B=\frac{4\sqrt{x}+12}{\left(3-\sqrt{x}\right)\left(3+\sqrt{x}\right)}\)
\(\Leftrightarrow B=\frac{4\left(\sqrt{x}+3\right)}{\left(3-\sqrt{x}\right)\left(\sqrt{x}+3\right)}\)
\(\Leftrightarrow B=\frac{4}{3-\sqrt{x}}\)
các bạn giải chi tiết giúp mk nhé. Cảm ơn 1. a Rút gọn biểu thức sau : A 5left(frac{1}{sqrt{2-sqrt{3}}}+sqrt{3-sqrt{5}}-frac{sqrt{10}}{2}right)^2+ left(frac{1}{sqrt{2+sqrt{3}}}+sqrt{3-sqrt{5}}-frac{sqrt{6}}{2}right)^2b) Cho biểu thức B left(frac{sqrt{x}+1}{sqrt{x}-1}-frac{sqrt{x}-1}{sqrt{x+1}}-frac{8sqrt{x}}{x-1}right):left(frac{sqrt{x}-x-3}{x-1}-frac{1}{sqrt{x}-1}right)Rút gọn biểu thức B và chứng minh B nhỏ hơn hoặc bằng 1 với mọi x lớn hơn hoặc bằng 0 và x khác 1
Đọc tiếp
các bạn giải chi tiết giúp mk nhé. Cảm ơn
1. a> Rút gọn biểu thức sau : A= \(5\left(\frac{1}{\sqrt{2-\sqrt{3}}}+\sqrt{3-\sqrt{5}}-\frac{\sqrt{10}}{2}\right)^2\)+ \(\left(\frac{1}{\sqrt{2+\sqrt{3}}}+\sqrt{3-\sqrt{5}}-\frac{\sqrt{6}}{2}\right)^2\)
b) Cho biểu thức B= \(\left(\frac{\sqrt{x}+1}{\sqrt{x}-1}-\frac{\sqrt{x}-1}{\sqrt{x+1}}-\frac{8\sqrt{x}}{x-1}\right):\left(\frac{\sqrt{x}-x-3}{x-1}-\frac{1}{\sqrt{x}-1}\right)\)
Rút gọn biểu thức B và chứng minh B nhỏ hơn hoặc bằng 1 với mọi x lớn hơn hoặc bằng 0 và x khác 1
Cho biêut thức P= \(\frac{\sqrt{x}}{\sqrt{x}-2}-\frac{2}{\sqrt{x}+2}\)-\(\frac{4\sqrt{x}}{x-4}\)với x lớn hoưn hoặc bằng 0, x khác 4
Rút gọn P
1/ Cho Dfrac{x^2-sqrt{x}}{x+sqrt{x}+1}-frac{x^2+sqrt{x}}{x-sqrt{x}+1}với 0≤x≤1
a) Rút gọn
b) CMinh 1-sqrt{D+x+1}sqrt{x}
2/Cho Efrac{15sqrt{x}-11}{x+2sqrt{x}-3}+frac{3sqrt{x}-2}{1-sqrt{x}}-frac{2sqrt{x}+3}{sqrt{x}+3}với x≥0 và x≠1
a) Rút gọn
b) Tìm giá trị của x để E frac{1}{2}
c) So sánh E với frac{2}{3}
3/Cho Gleft(frac{x-3sqrt{x}}{x-9}-1right):left(frac{9-x}{x+sqrt{x}-6}+frac{sqrt{x}-3}{sqrt{x}-2}-frac{sqrt{x}+2}{sqrt{x}+3}right)với x≥0,x≠4,x≠9
a) Rút gọn
b) Tìm x để G1
Đọc tiếp
1/ Cho D=\(\frac{x^2-\sqrt{x}}{x+\sqrt{x}+1}-\frac{x^2+\sqrt{x}}{x-\sqrt{x}+1}\)với 0≤x≤1
a) Rút gọn
b) CMinh 1\(-\sqrt{D+x+1}=\sqrt{x}\)
2/Cho E=\(\frac{15\sqrt{x}-11}{x+2\sqrt{x}-3}+\frac{3\sqrt{x}-2}{1-\sqrt{x}}-\frac{2\sqrt{x}+3}{\sqrt{x}+3}\)với x≥0 và x≠1
a) Rút gọn
b) Tìm giá trị của x để E = \(\frac{1}{2}\)
c) So sánh E với \(\frac{2}{3}\)
3/Cho G=\(\left(\frac{x-3\sqrt{x}}{x-9}-1\right):\left(\frac{9-x}{x+\sqrt{x}-6}+\frac{\sqrt{x}-3}{\sqrt{x}-2}-\frac{\sqrt{x}+2}{\sqrt{x}+3}\right)\)với x≥0,x≠4,x≠9
a) Rút gọn
b) Tìm x để G<1
Cho biêut thức P= \(\frac{\sqrt{x}}{\sqrt{x}-2}-\frac{2}{\sqrt{x}+2}\)-\(\frac{4\sqrt{x}}{x-4}\)với x lớn hoưn hoặc bằng 0, x khác 4
Tìm số nguyên x để P là số nguyên
\(ĐKXĐ:\hept{\begin{cases}x\ge0\\x\ne\pm2\end{cases}}\)
\(P=\frac{\sqrt{x}}{\sqrt{x}-2}-\frac{2}{\sqrt{x}+2}-\frac{4\sqrt{x}}{x-4}\)
\(\Leftrightarrow P=\frac{x+2\sqrt{x}-2\sqrt{x}+4-4\sqrt{x}}{\left(\sqrt{x}-2\right)\left(\sqrt{x}+2\right)}\)
\(\Leftrightarrow P=\frac{x-4\sqrt{x}+4}{\left(\sqrt{x}-2\right)\left(\sqrt{x}+2\right)}\)
\(\Leftrightarrow P=\frac{\sqrt{x}-2}{\sqrt{x}+2}\)
Để P là số nguyên \(\Leftrightarrow\frac{\sqrt{x}-2}{\sqrt{x}+2}\)là số nguyên
\(\Leftrightarrow\sqrt{x}-2⋮\sqrt{x}+2\)
\(\Leftrightarrow4⋮\sqrt{x}+2\)
\(\Leftrightarrow\sqrt{x}+2\inƯ\left(4\right)=\left\{\pm1;\pm2;\pm4\right\}\)
\(\Leftrightarrow\sqrt{x}\in\left\{-3;-1;-4;0;-6;2\right\}\)
Loại những giá trị \(\sqrt{x}\in\left\{-3;-1;-4;-6;2\right\}\)
\(\Leftrightarrow\sqrt{x}=0\)
\(\Leftrightarrow x=0\)
Vậy để P là số nguyên \(\Leftrightarrow x=0\)
Cho mình sửa 1 chút nhé :
\(ĐKXĐ:\hept{\begin{cases}x\ge0\\x\ne4\end{cases}}\)
Cho M=\(\frac{\sqrt{x}}{\sqrt{x}+1}\) với x > hoặc = 0. Khi M=\(\sqrt{x}-2\)thì x bằng bao nhiêu?
Học tốt:))
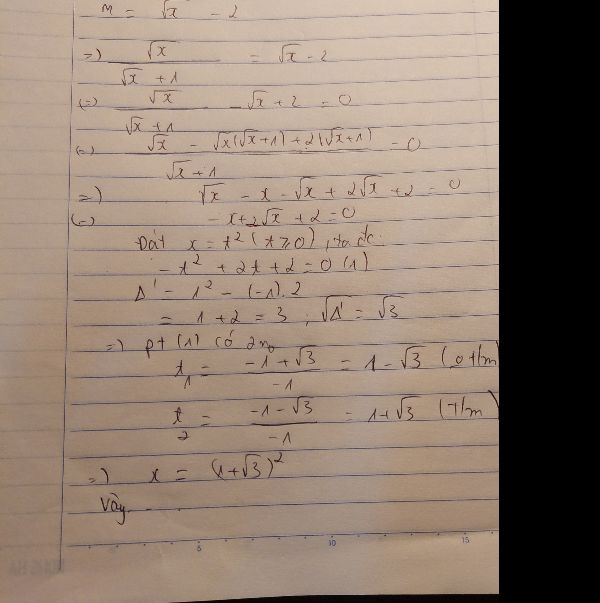
\(M=\frac{\sqrt{x}}{\sqrt{x}+1}\left(x\ge0\right)\)
Khi \(M=\sqrt{x}-2\)
\(\Rightarrow\frac{\sqrt{x}}{\sqrt{x}+1}=\sqrt{x}-2\)
\(\Leftrightarrow\sqrt{x}=\left(\sqrt{x}-2\right)\left(\sqrt{x}+1\right)\)
\(\Leftrightarrow\sqrt{x}=x+\sqrt{x}-2\sqrt{x}-2\)
\(\Leftrightarrow\sqrt{x}=x-\sqrt{x}-2\)
\(\Leftrightarrow x-\sqrt{x}-\sqrt{x}-2=0\)
\(\Leftrightarrow x-2\sqrt{x}+1-3=0\)
\(\Leftrightarrow\left(\sqrt{x}-1\right)^2=3\)
\(\Leftrightarrow\left(\sqrt{x}-1\right)^2=\left(\pm\sqrt{3}\right)^2\)
\(\Leftrightarrow\sqrt{x}-1=\pm\sqrt{3}\)
\(\Leftrightarrow\sqrt{x}=\pm\sqrt{3}+1\)
\(\Leftrightarrow\orbr{\begin{cases}x=\left(\sqrt{3}+1\right)^2\\x=\left(-\sqrt{3}+1\right)^2\end{cases}}\)
\(\Leftrightarrow\orbr{\begin{cases}x=3+2\sqrt{3}+1\\1-2\sqrt{3}+3\end{cases}}\)
\(\Leftrightarrow\orbr{\begin{cases}x=4+2\sqrt{3}\\x=4-2\sqrt{3}\end{cases}}\)
Vậy \(x\in\left\{4\pm2\sqrt{3}\right\}\)khi \(M=\sqrt{x}-2\)
Bạn kia làm sai rồi:)) khi căn x bằng 1 - căn 3 ko thể bình phương lên được vì 1- căn 3


