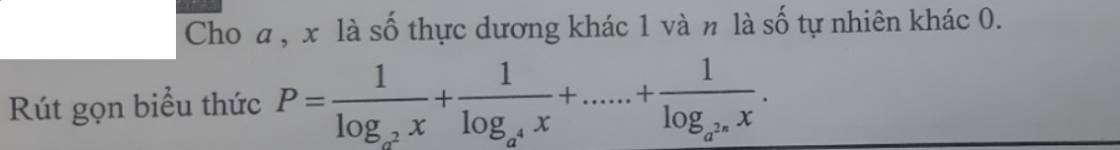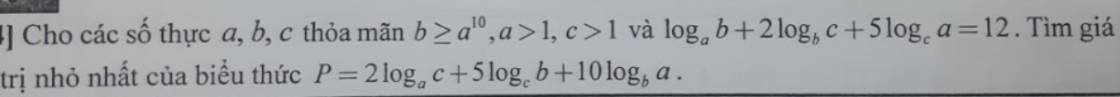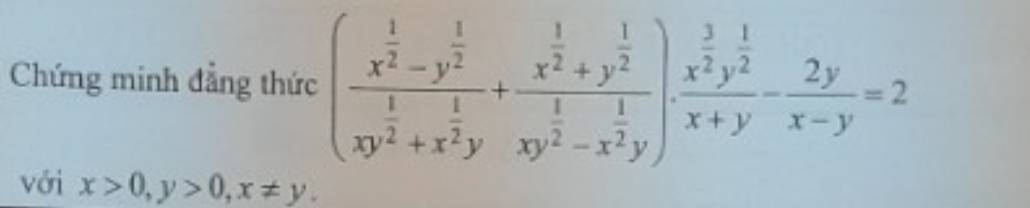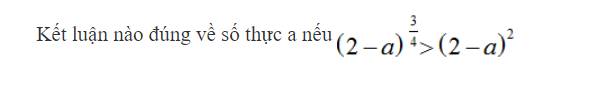
Bài 1: Lũy thừa

Do \(\dfrac{3}{4}\) không nguyên nên trước hết \(2-a>0\Rightarrow a< 2\)

Sau đó \(\left(2-a\right)^{\dfrac{3}{4}}>\left(2-a\right)^2\) mà \(2>\dfrac{3}{4}\Rightarrow2-a< 1\Rightarrow a>1\)

\(\Rightarrow1< a< 2\)

A sai luôn; do ab<0 nên \(lna\) và \(lnb\) không tồn tại

\(P=\dfrac{1}{\dfrac{1}{2}log_ax}+\dfrac{1}{\dfrac{1}{4}log_ax}+...+\dfrac{1}{\dfrac{1}{2n}log_ax}\)

\(=\dfrac{2+4+...+2n}{log_ax}=\dfrac{n\left(n+1\right)}{log_ax}\)

\(log_ab=\dfrac{3}{2}\Rightarrow b=a^{\dfrac{3}{2}}=\left(\sqrt[]{a}\right)^3\)

Do b nguyên \(\Rightarrow\sqrt[]{a}\) nguyên \(\Rightarrow\sqrt[]{a}=x\Rightarrow a=x^2\)

\(log_cd=\dfrac{5}{4}\Rightarrow d=\left(\sqrt[4]{c}\right)^5\Rightarrow\sqrt[4]{c}\) nguyên \(\Rightarrow\sqrt[4]{c}=y\Rightarrow c=y^4\)

\(log_3\left(a-c\right)=2\Rightarrow a-c=9\)

\(\Rightarrow x^2-y^4=9\Rightarrow\left(x-y^2\right)\left(x+y^2\right)=9=1.9=9.1=3.3\)

Pt trên có cặp nghiệm nguyên dương duy nhất \(\left(x;y\right)=\left(5;2\right)\)

\(\Rightarrow log_3\left(b-d\right)=log_3\left(5^3-2^5\right)\)

\(log_{18}4200=\dfrac{log_24200}{log_218}=\dfrac{log_2\left(2^3.3.5^2.7\right)}{log_2\left(2.3^2\right)}=\dfrac{3+log_23+2log_25+log_27}{1+2log_23}\)

\(log_412=log_{2^2}\left(2^2.3\right)=\dfrac{1}{2}log_2\left(2^2.3\right)=\dfrac{1}{2}\left(2+log_23\right)=c\)

\(\Rightarrow log_23=2c-2\)

\(log_25=\dfrac{log_95}{log_92}=log_95.log_29=2log_23.log_95=2a\left(2c-2\right)=4ac-4a\)

Thế vào trên: \(log_{18}4200=\dfrac{3+2c-2+2\left(4ac-4a\right)+b}{1+2\left(2c-2\right)}\)

\(log\dfrac{1}{2}+log\dfrac{2}{3}+...+log\dfrac{149}{150}=log\left(\dfrac{1.2...149}{2.3...150}\right)=log\dfrac{1}{150}=-log150\)

\(\Rightarrow I=\dfrac{log150}{log126}=\dfrac{log_2150}{log_2126}=\dfrac{1+log_23+2log_25}{1+2log_23+log_27}\)

\(log_25=\dfrac{log_35}{log_32}=log_35.log_23=ac\)

\(\Rightarrow I=\dfrac{1+c+2ac}{1+2c+b}\)

\(log_{ab}b=\dfrac{1}{log_bab}=\dfrac{1}{log_ba+1}=\dfrac{1}{\dfrac{1}{log_ab}+1}=\dfrac{log_ab}{log_ab+1}\)

Đặt \(log_ab=x\) (cho gọn biểu thức)

\(P=\left(x+\dfrac{1}{x}+2\right)\left(x-\dfrac{x}{x+1}\right).\dfrac{1}{x}-1\)

\(=\left(\dfrac{x^2+2x+1}{x}\right)\left(\dfrac{x}{x+1}\right).\dfrac{1}{x}-1\)

\(=\dfrac{x+1}{x}-1=\dfrac{1}{x}=log_ba\)

Đặt \(\left\{{}\begin{matrix}x=log_ab\ge10\\y=log_bc>0\\z=log_ca>0\end{matrix}\right.\) \(\Rightarrow\left\{{}\begin{matrix}xyz=1\\x+2y+5z=12\end{matrix}\right.\) 

\(P=\dfrac{10}{x}+\dfrac{5}{y}+\dfrac{2}{z}=\dfrac{10}{x}+\dfrac{2y+5z}{yz}=\dfrac{10}{x}+x\left(12-x\right)\)

Thật kì lạ, ko hiểu người ta cho điều kiện \(b\ge a^{10}\) làm gì, nó khiến cho P ko tồn tại min (chỉ tồn tại max =21)

\(log_a\left(ac^2\right)=log_c\left(b^3c\right)\Leftrightarrow1+2log_ac=1+3log_cb\)

\(\Leftrightarrow\left\{{}\begin{matrix}2log_ac-3log_cb=0\\2log_ac+log_cb=8\end{matrix}\right.\)

\(\Rightarrow\left\{{}\begin{matrix}log_ac=3\\log_cb=2\end{matrix}\right.\)

\(\Rightarrow P=log_ab+log_ca+2log_cb=\dfrac{log_cb}{log_ca}+log_ca+2log_cb=log_ca.log_cb+log_ca+2log_cb\)

\(VT=\left(\dfrac{x^{\dfrac{1}{2}}-y^{\dfrac{1}{2}}}{xy^{\dfrac{1}{2}}+x^{\dfrac{1}{2}}y}+\dfrac{x^{\dfrac{1}{2}}+y^{\dfrac{1}{2}}}{xy^{\dfrac{1}{2}}-x^{\dfrac{1}{2}}y}\right)\cdot\dfrac{x^{\dfrac{3}{2}}y^{\dfrac{1}{2}}}{x+y}-\dfrac{2y}{x-y}\)

\(=\left(\dfrac{\sqrt{x}-\sqrt{y}}{x\sqrt{y}+y\sqrt{x}}+\dfrac{\sqrt{x}+\sqrt{y}}{x\sqrt{y}-y\sqrt{x}}\right)\cdot\dfrac{x^{\dfrac{1}{2}}y^{\dfrac{1}{2}}\cdot x}{x+y}-\dfrac{2y}{x-y}\)

\(=\left(\dfrac{\sqrt{x}-\sqrt{y}}{\sqrt{xy}\left(\sqrt{x}+\sqrt{y}\right)}+\dfrac{\sqrt{x}+\sqrt{y}}{\sqrt{xy}\left(\sqrt{x}-\sqrt{y}\right)}\right)\cdot\dfrac{\sqrt{xy}\cdot x}{x+y}-\dfrac{2y}{x-y}\)

\(=\dfrac{\left(\sqrt{x}-\sqrt{y}\right)^2+\left(\sqrt{x}+\sqrt{y}\right)^2}{\sqrt{xy}\left(\sqrt{x}-\sqrt{y}\right)\left(\sqrt{x}+y\right)}\cdot\dfrac{x\sqrt{xy}}{x+y}-\dfrac{2y}{x-y}\)

\(=\dfrac{2\left(x+y\right)}{x-y}\cdot\dfrac{x}{x+y}-\dfrac{2y}{x-y}\)

\(=\dfrac{2x\left(x+y\right)-2y\left(x+y\right)}{\left(x-y\right)\left(x+y\right)}=\dfrac{2x^2+2xy-2xy-2y^2}{x^2-y^2}\)

\(=\dfrac{2x^2-2y^2}{x^2-y^2}=2\)