\(x^2+y^2+2\left(x+y\right)-xy=0\)

\(\Leftrightarrow4x^2-4xy+4y^2+8\left(x+y\right)=0\)

\(\Leftrightarrow\left(2x-y\right)^2+4\left(2x-y\right)+4+3y^2+12y+12=-16\)

\(\Leftrightarrow\left(2x-y+2\right)^2+3\left(y+2\right)^2=-16\)

Dễ thấy VT \(\ge0\) ; VP < 0 nên phương trình vô nghiệm 

\(x^2+y^2-2\left(x+y\right)=xy\)

\(\Rightarrow x^2-2x+1+y^2-2y+1=2+xy\)

\(\Rightarrow\left(x-1\right)^2+\left(y-1\right)^2=2+xy\)

Ta lại có : \(\left(x-1\right)^2+\left(y-1\right)^2\ge2\left(x-1\right)\left(y-1\right)\) (Bất đẳng thức Cauchy)

Tiếp tục phần tiếp theo

Dấu bằng xảy ra khi \(\left\{{}\begin{matrix}x=2\\y=2\end{matrix}\right.\)

\(\Rightarrow\left(x-1\right)^2+\left(y-1\right)^2=2+xy\) (vô lý vì 2=2+2.2)

⇒ Không có cặp (x;y) nguyên dương nào thỏa mãn đề bài

3x+4y+xy=1

ó x(3-y) + 4y = 1

ó x(3-y) -12 + 4y = 1 - 12

ó x(3-y) - 4(3-y) = -13

ó (x - 4 )( 3 - y ) = -13

Ta có bảng:

Vậy bn tự kết luận gt x,y

lỗi chữ, chỗ từ ó kia là dấu <=> nha

\(\Leftrightarrow\left(x-y\right)\left(x+y\right)=2017=1.2017\)

\(\Leftrightarrow\left[{}\begin{matrix}\left\{{}\begin{matrix}x-y=1\\x+y=2017\end{matrix}\right.\\\left\{{}\begin{matrix}x-y=-1\\x+y=-2017\end{matrix}\right.\end{matrix}\right.\)

\(\Leftrightarrow\left[{}\begin{matrix}\left\{{}\begin{matrix}x=1009\\y=1008\end{matrix}\right.\\\left\{{}\begin{matrix}x=-1009\\y=-1008\end{matrix}\right.\end{matrix}\right.\)

x² - xy + 3x - y = 5

\(\Leftrightarrow\) x(x - y) + x - y + 2x = 5

\(\Leftrightarrow\) (x - y)(x + 1) + 2x + 2 = 7

\(\Leftrightarrow\) (x - y)(x + 1) + 2(x + 1) = 7

\(\Leftrightarrow\) (x - y + 2)(x + 1) = 7

Vì x, y \(\in\) Z nên (x - y + 2)(x + 1) \(\in\) Z

Xét các TH:

TH1: \(\left\{{}\begin{matrix}x-y+2=7\\x+1=1\end{matrix}\right.\)

\(\Leftrightarrow\) \(\left\{{}\begin{matrix}2-y=7\\x=0\end{matrix}\right.\)

\(\Leftrightarrow\) \(\left\{{}\begin{matrix}x=0\\y=-5\end{matrix}\right.\) (TM)

TH2: \(\left\{{}\begin{matrix}x-y+2=-7\\x+1=-1\end{matrix}\right.\)

\(\Leftrightarrow\) \(\left\{{}\begin{matrix}-2-y+2=-7\\x=-2\end{matrix}\right.\)

\(\Leftrightarrow\) \(\left\{{}\begin{matrix}x=-2\\y=7\end{matrix}\right.\) (TM)

TH3: \(\left\{{}\begin{matrix}x-y+2=1\\x+1=7\end{matrix}\right.\)

\(\Leftrightarrow\) \(\left\{{}\begin{matrix}6-y+2=1\\x=6\end{matrix}\right.\)

\(\Leftrightarrow\) \(\left\{{}\begin{matrix}x=6\\y=7\end{matrix}\right.\) (TM)

TH4: \(\left\{{}\begin{matrix}x-y+2=-1\\x+1=-7\end{matrix}\right.\)

\(\Leftrightarrow\) \(\left\{{}\begin{matrix}-8-y+2=-1\\x=-8\end{matrix}\right.\)

\(\Leftrightarrow\) \(\left\{{}\begin{matrix}x=-8\\y=-5\end{matrix}\right.\) (TM)

Vậy ...

Chúc bn học tốt!

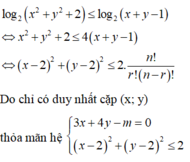
nên đường thẳng 3x + 4y - m = 0 là tiếp tuyến của đường tròn (x – 2)² + (y – 2)² = 2.

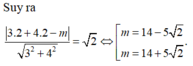
Chọn C.

x=1

y=1

hết rồi

Vãi mình hỏi cách làm mà