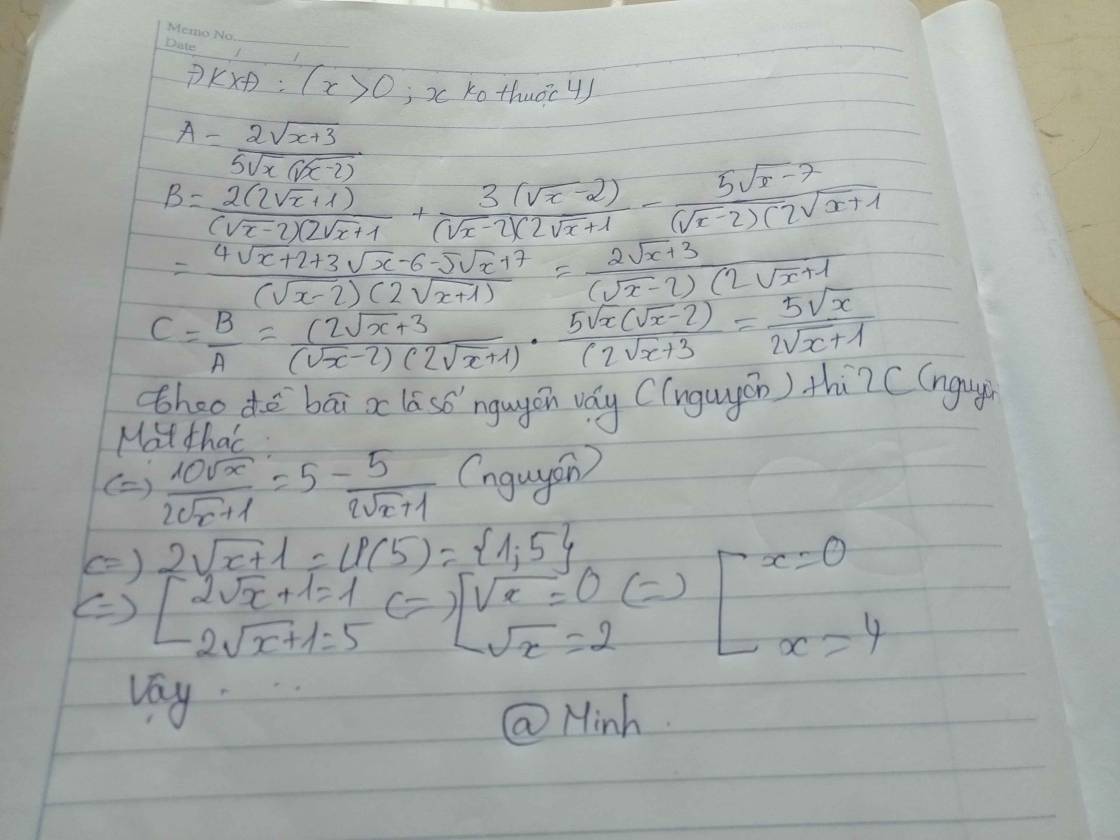b: Ta có: \(B=\left(\dfrac{\sqrt{x}+2}{x+2\sqrt{x}+1}-\dfrac{\sqrt{x}-2}{x-1}\right)\cdot\left(\dfrac{x\sqrt{x}-1}{\sqrt{x}-1}+\dfrac{x+\sqrt{x}}{\sqrt{x}+1}\right)\)

\(=\dfrac{\left(\sqrt{x}+2\right)\left(\sqrt{x}-1\right)-\left(\sqrt{x}-2\right)\left(\sqrt{x}+1\right)}{\left(\sqrt{x}+1\right)^2\cdot\left(\sqrt{x}-1\right)}\cdot\left(x+\sqrt{x}+1+\sqrt{x}\right)\)

\(=\dfrac{x+\sqrt{x}-2-x+\sqrt{x}+2}{\sqrt{x}-1}\)

\(=\dfrac{2\sqrt{x}}{\sqrt{x}-1}\)

\(a,x=16\Rightarrow A=\dfrac{\sqrt{16}+2}{\sqrt{16}-3}=\dfrac{4+2}{4-3}=6\)

\(b,B=\dfrac{\sqrt{x}+5}{\sqrt{x}+1}+\dfrac{\sqrt{x}-7}{1-x}\left(dk:x\ge0,x\ne1,x\ne9\right)\\ =\dfrac{\sqrt{x}+5}{\sqrt{x}+1}-\dfrac{\sqrt{x}-7}{\left(\sqrt{x}-1\right)\left(\sqrt{x}+1\right)}\\ =\dfrac{\left(\sqrt{x}+5\right)\left(\sqrt{x}-1\right)-\left(\sqrt{x}-7\right)}{x-1}\\ =\dfrac{x+4\sqrt{x}-5-\sqrt{x}+7}{x-1}\\ =\dfrac{x+3\sqrt{x}+2}{x-1}\\ =\dfrac{\left(\sqrt{x}+2\right)\left(\sqrt{x}+1\right)}{\left(\sqrt{x}-1\right)\left(\sqrt{x}+1\right)}\\ =\dfrac{\sqrt{x}+2}{\sqrt{x}-1}\left(dpcm\right)\)

\(c,\dfrac{4A}{A}\le\dfrac{x}{\sqrt{x}-3}\Leftrightarrow\dfrac{4\left(\sqrt{x}+2\right)}{\sqrt{x}-3}:\dfrac{\sqrt{x}+2}{\sqrt{x}-3}\le\dfrac{x}{\sqrt{x}-3}\)

\(\Leftrightarrow\dfrac{4\left(\sqrt{x}+2\right)}{\sqrt{x}-3}.\dfrac{\sqrt{x}-3}{\sqrt{x}+2}\le\dfrac{x}{\sqrt{x}-3}\)

\(\Leftrightarrow4-\dfrac{x}{\sqrt{x}-3}\le0\)

\(\Leftrightarrow\dfrac{4\sqrt{x}-12-x}{\sqrt{x}-3}\le0\)

\(\Leftrightarrow\) Pt vô nghiệm

Vậy không có giá trị x thỏa yêu cầu đề bài.

a: Thay x=9 vào A, ta được:

\(A=\dfrac{3+2}{3-5}=\dfrac{5}{-2}=\dfrac{-5}{2}\)

\(B=\dfrac{3\sqrt{x}-15+20-2\sqrt{x}}{x-25}=\dfrac{\sqrt{x}+5}{x-25}=\dfrac{1}{\sqrt{x}-5}\)

b: Để \(A=B\cdot\left|x-4\right|\) thì \(\left|x-4\right|=\dfrac{A}{B}=\dfrac{\sqrt{x}+2}{\sqrt{x}-5}:\dfrac{1}{\sqrt{x}-5}=\sqrt{x}+2\)

\(\Leftrightarrow x-4=\sqrt{x}+2\)

\(\Leftrightarrow x-\sqrt{x}-6=0\)

=>x=9

`A)đk:x>=0,x ne 25`

`A=9=>A=(3+2)/(3-5)=-5/2`

`B)B=(3sqrtx-15+20-2sqrtx)/(x-25)`

`=(sqrtx+5)/(x-25)`

`=1/(sqrtx-5)`

`A=B.|x-4|`

`<=>A/B=|x-4|`

`<=>\sqrtx+2=|x-4|`

`<=>\sqrtx+2=(sqrtx+2)|sqrtx-2|`

`<=>|sqrtx-2|=1`

`+)sqrtx-2=1<=>x=9(tm)`

`+)sqrtx-2=-1<=>x=1(tm)`

Vậy `S={1,9}`

a, Thay x=9 vào biểu thức A ta có

\(A=\dfrac{\sqrt{9}+2}{\sqrt{9}-5}\)

\(A=\dfrac{3+2}{3-5}=\dfrac{5}{-2}=-2,5\)

Vậy A =-2,5 khi x=9

a. A=\(\dfrac{\sqrt{x}+2}{\sqrt{x}-5}\)

=\(\dfrac{\sqrt{9}+2}{\sqrt{9}-5}=\dfrac{-5}{2}\)

a: ta có: \(M=\dfrac{a}{\sqrt{ab}+b}+\dfrac{b}{\sqrt{ab}-a}-\dfrac{a+b}{\sqrt{ab}}\)

\(=\dfrac{a\left(\sqrt{ab}-a\right)+b\left(\sqrt{ab}+b\right)}{\left(\sqrt{ab}+b\right)\left(\sqrt{ab}-a\right)}-\dfrac{a+b}{\sqrt{ab}}\)

\(=\dfrac{-\sqrt{ab}\left(a+b\right)+\left(a-b\right)\left(a+b\right)}{\sqrt{b}\left(\sqrt{a}+\sqrt{b}\right)\cdot\sqrt{a}\cdot\left(\sqrt{a}-\sqrt{b}\right)}-\dfrac{a+b}{\sqrt{ab}}\)

\(=\dfrac{-\sqrt{ab}\left(a+b\right)+\left(a-b\right)\left(a+b\right)}{\sqrt{ab}\left(a-b\right)}-\dfrac{a^2-b^2}{\sqrt{ab}\left(a-b\right)}\)

\(=\dfrac{-\sqrt{ab}}{\sqrt{ab}\left(a-b\right)}\)

\(=-\dfrac{1}{a-b}\)

b: Thay \(a=\sqrt{5}+1\) và \(b=\sqrt{5}-1\) vào M, ta được:

\(M=\dfrac{-1}{\sqrt{5}+1-\sqrt{5}+1}=\dfrac{-1}{2}\)

1: Thay x=16 vào A, ta được:

\(A=\dfrac{6-2\cdot4}{4-5}=\dfrac{-2}{-1}=2\)

a: Thay \(x=6-2\sqrt{5}\) vào A, ta được:

\(A=1-\dfrac{\sqrt{5}-1}{\sqrt{5}-1+1}=1-\dfrac{\sqrt{5}-1}{\sqrt{5}}=\dfrac{\sqrt{5}}{5}\)

b: Ta có: P=A:B

\(=\left(1-\dfrac{\sqrt{x}}{\sqrt{x}+1}\right):\left(\dfrac{\sqrt{x}-1}{\sqrt{x}-2}-\dfrac{\sqrt{x}+2}{\sqrt{x}-3}+\dfrac{5\sqrt{x}-10}{x-5\sqrt{x}+6}\right)\)

\(=\dfrac{1}{\sqrt{x}+1}:\dfrac{x-4\sqrt{x}+3-x+4+5\sqrt{x}-10}{\left(\sqrt{x}-2\right)\left(\sqrt{x}-3\right)}\)

\(=\dfrac{1}{\sqrt{x}+1}:\dfrac{1}{\sqrt{x}-2}\)

\(=\dfrac{\sqrt{x}-2}{\sqrt{x}+1}\)

a: Thay \(x=\dfrac{1}{4}\) vào A, ta được:

\(A=\left(\dfrac{1}{2}+1\right):\left(\dfrac{1}{2}-2\right)=\dfrac{3}{2}:\dfrac{-3}{2}=-1\)

b: Ta có: \(B=\dfrac{\sqrt{x}+2}{\sqrt{x}-3}+\dfrac{\sqrt{x}-8}{x-5\sqrt{x}+6}\)

\(=\dfrac{x-4+\sqrt{x}-8}{\left(\sqrt{x}-3\right)\left(\sqrt{x}-2\right)}\)

\(=\dfrac{x+\sqrt{x}-12}{\left(\sqrt{x}-3\right)\left(\sqrt{x}-2\right)}\)

\(=\dfrac{\sqrt{x}+4}{\sqrt{x}-2}\)

c: Để B là số tự nhiên thì \(\sqrt{x}+4⋮\sqrt{x}-2\)

\(\Leftrightarrow\sqrt{x}-2\in\left\{1;2;3;6\right\}\)

\(\Leftrightarrow\sqrt{x}\in\left\{3;4;5;8\right\}\)

hay \(x\in\left\{16;25;64\right\}\)