giups minhf vs aj

Cacs banj owi giups minhf vowis mays minhf ko ddanhs daaus ddc!
Minhf ko vieets ddc daaus banj owi!
giups minhf
1 The Temple of Literature is about 10 minutes away from Hoan Kiem Lake
2 There are five courtyards
3 It''s used for street signs of Ha Noi
4 We can find the stone tablets above tortoise backs with thenames of doctors in the third courtyard
EX4
1 : My town was visited by hundreds of tourists last year
tìm x y
x^3-3x^2+7x-21=2y
xy-2x-3y=5
2xy-3x+5y=8
giups minhf vs
giups minhf voiws
giups minhf baif 4
a) \(\dfrac{-8}{15}-\left(\dfrac{1}{9}+\dfrac{7}{15}-\dfrac{5}{18}\right)\)
\(=\dfrac{-8}{15}-\dfrac{1}{9}-\dfrac{7}{15}+\dfrac{5}{18}\\ =\left(-\dfrac{8}{15}-\dfrac{7}{15}\right)+\left(\dfrac{5}{18}-\dfrac{1}{9}\right)\\ =-1+\dfrac{1}{6}=-\dfrac{5}{6}\)
b) \(\dfrac{1}{2}.\dfrac{-1}{14}+\dfrac{1}{2}.\dfrac{9}{14}+\dfrac{-5}{14}.\dfrac{1}{2}\)
\(=\dfrac{1}{2}.\left(\dfrac{-1}{14}+\dfrac{9}{14}+\dfrac{-5}{14}\right)\\ =\dfrac{1}{2}.\dfrac{3}{14}=\dfrac{3}{28}\)
Minhf caanf gaasp aj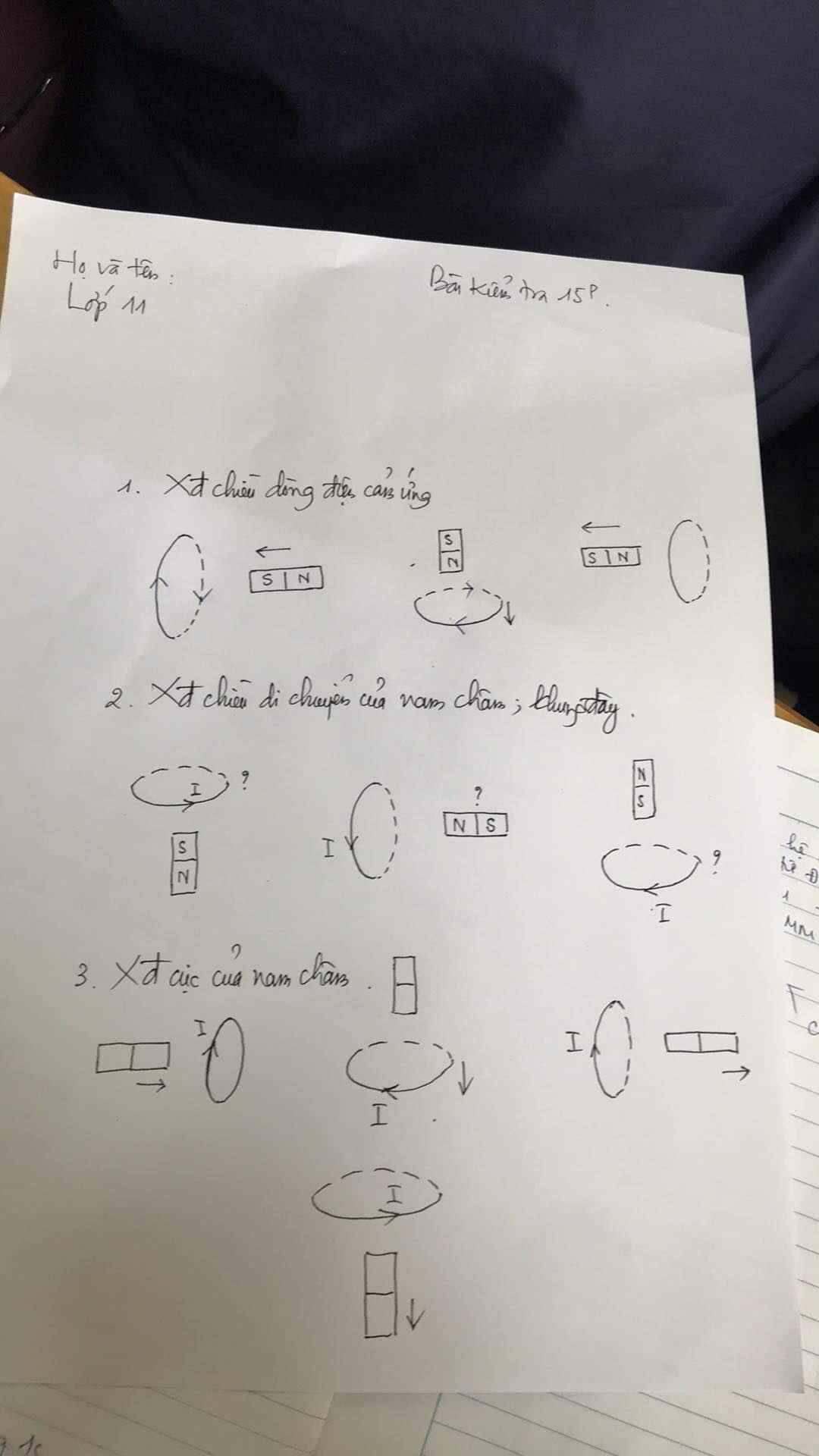
giups minhf voiws cacs banj!!!! 🙏😭🥺
GIAIR DDAAYF DDUR GIUPS MINHF VOIWS AJ
MINHF CAMR OWN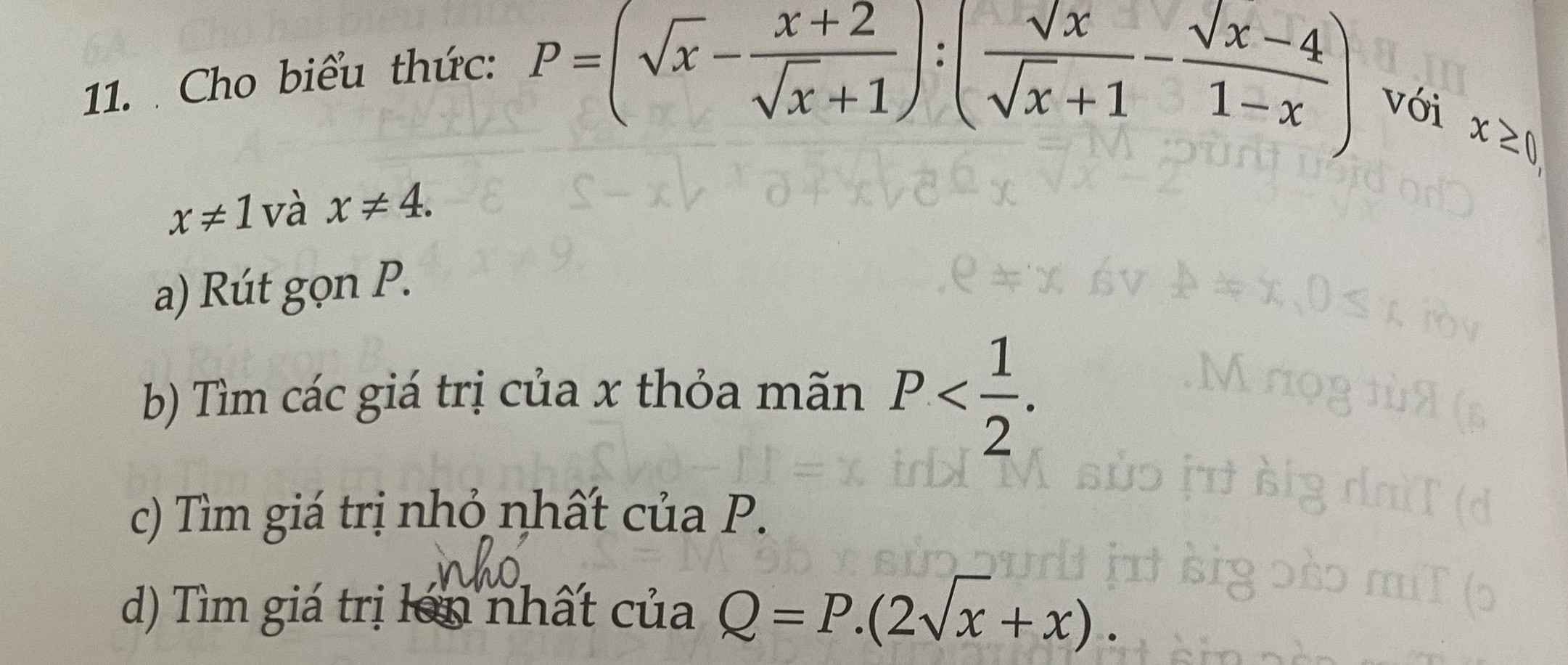
a, \(P=\left(\sqrt{x}-\dfrac{x+2}{\sqrt{x}+1}\right):\left(\dfrac{\sqrt{x}}{\sqrt{x}+1}-\dfrac{\sqrt{x}-4}{1-x}\right)\)
\(=\left(\dfrac{x+\sqrt{x}}{\sqrt{x}+1}-\dfrac{x+2}{\sqrt{x}+1}\right):\left[\dfrac{\sqrt{x}\left(\sqrt{x}-1\right)}{\left(\sqrt{x}+1\right)\left(\sqrt{x}-1\right)}+\dfrac{\sqrt{x}-4}{\left(\sqrt{x}+1\right)\left(\sqrt{x}-1\right)}\right]\)
\(=\dfrac{\sqrt{x}-2}{\sqrt{x}+1}:\dfrac{x-4}{\left(\sqrt{x}+1\right)\left(\sqrt{x}-1\right)}\)
\(=\dfrac{\sqrt{x}-2}{\sqrt{x}+1}.\dfrac{\left(\sqrt{x}+1\right)\left(\sqrt{x}-1\right)}{\left(\sqrt{x}+2\right)\left(\sqrt{x}-2\right)}\)
\(=\dfrac{\sqrt{x}-1}{\sqrt{x}+2}\)
b, \(P< \dfrac{1}{2}\Leftrightarrow\dfrac{\sqrt{x}-1}{\sqrt{x}+2}< \dfrac{1}{2}\)
\(\Leftrightarrow2\sqrt{x}-2< \sqrt{x}+2\)
\(\Leftrightarrow\sqrt{x}< 4\)
\(\Leftrightarrow0\le x< 16\)
Vậy \(0\le x< 16;x\ne1;x\ne4\).
a: ta có: \(P=\left(\sqrt{x}-\dfrac{x+2}{\sqrt{x}+1}\right):\left(\dfrac{\sqrt{x}}{\sqrt{x}+1}+\dfrac{\sqrt{x}-4}{x-1}\right)\)
\(=\dfrac{x+\sqrt{x}-x-2}{\left(\sqrt{x}+1\right)}:\dfrac{x-\sqrt{x}+\sqrt{x}-4}{\left(\sqrt{x}+1\right)\left(\sqrt{x}-1\right)}\)
\(=\dfrac{\sqrt{x}-2}{1}\cdot\dfrac{\sqrt{x}-1}{\left(\sqrt{x}-2\right)\left(\sqrt{x}+2\right)}\)
\(=\dfrac{\sqrt{x}-1}{\sqrt{x}+2}\)