4x^2 - (x+1)=0
JS
Những câu hỏi liên quan
(x-3)^3 +(x+3)^3=0
(x+1)^3-(x-1)^3=0
x^2-4x+3=0
4x^2 +4x +1=0
(x+2)^2-(x+3)^2=0
\(\left(x-3\right)^3+\left(x+3\right)^3=0\)
\(\Leftrightarrow x^3-9x^2+27x-27+x^3+9x^2+27x+27=0\)\(\Leftrightarrow2x^3+54x^2=0\)
\(\Leftrightarrow x^2\left(2x+54\right)=0\)
\(\Rightarrow\left[{}\begin{matrix}x=0\\2x+54=0\end{matrix}\right.\Rightarrow\left[{}\begin{matrix}x=0\\x=-27\end{matrix}\right.\)
\(b,\left(x+1\right)^3-\left(x-1\right)^3=0\)
\(\Leftrightarrow x^3+3x^2+3x+1-x^3+3x^2-3x+1=0\)\(\Leftrightarrow6x^2+2=0\)
\(\Leftrightarrow6x^2=-2\)
\(\Leftrightarrow x^2=-3\) ( vô lí)
Vậy pt vô nghiệm
\(c,x^2-4x+3=0\)
\(\Leftrightarrow x^2-3x-x+3=0\)
\(\Leftrightarrow x\left(x-3\right)-\left(x-3\right)=0\)
\(\Leftrightarrow\left(x-1\right)\left(x-3\right)=0\)
\(\Leftrightarrow\left[{}\begin{matrix}x-1=0\\x-3=0\end{matrix}\right.\Rightarrow\left[{}\begin{matrix}x=1\\x=3\end{matrix}\right.\)
\(d,4x^2+4x+1=0\)
\(\Leftrightarrow\left(2x+1\right)^2=0\)
\(\Rightarrow2x+1=0\)
\(\Leftrightarrow2x=-1\Rightarrow x=-\dfrac{1}{2}\)
\(e,\left(x+2\right)^2-\left(x+3\right)^2=0\)
\(\Leftrightarrow\left(x+2-x-3\right)\left(x+2+x+3\right)=0\)
\(\Leftrightarrow-\left(2x+5\right)=0\)
\(\Leftrightarrow-2x-5=0\)
\(\Leftrightarrow-2x=5\Rightarrow x=-\dfrac{5}{2}\)
Học tốt nha you <3
Đúng 0
Bình luận (0)
(x-3)^3 +(x+3)^3=0
(x+1)^3-(x-1)^3=0
x^2-4x+3=0
4x^2 +4x +1=0
(x+2)^2-(x+3)^2=0
\(\left(x-3\right)^3+\left(x+3\right)^3=0\)
\(\Leftrightarrow x^3-9x^2+27x-27+x^3+9x^2+27x+27=0\)\(\Leftrightarrow2x^3+54x^2=0\)
\(\Leftrightarrow x^2\left(2x+54\right)=0\)
\(\Rightarrow\left[{}\begin{matrix}x=0\\2x+54=0\end{matrix}\right.\Rightarrow\left[{}\begin{matrix}x=0\\x=-27\end{matrix}\right.\)
\(b,\left(x+1\right)^3-\left(x-1\right)^3=0\)
\(\Leftrightarrow x^3+3x^2+3x+1-x^3+3x^2-3x+1=0\)\(\Leftrightarrow6x^2+2=0\)
\(\Leftrightarrow6x^2=-2\)
\(\Leftrightarrow x^2=-3\) ( vô lí)
Vậy pt vô nghiệm
\(c,x^2-4x+3=0\)
\(\Leftrightarrow x^2-3x-x+3=0\)
\(\Leftrightarrow x\left(x-3\right)-\left(x-3\right)=0\)
\(\Leftrightarrow\left(x-1\right)\left(x-3\right)=0\)
\(\Leftrightarrow\left[{}\begin{matrix}x-1=0\\x-3=0\end{matrix}\right.\Rightarrow\left[{}\begin{matrix}x=1\\x=3\end{matrix}\right.\)
\(d,4x^2+4x+1=0\)
\(\Leftrightarrow\left(2x+1\right)^2=0\)
\(\Rightarrow2x+1=0\)
\(\Leftrightarrow2x=-1\Rightarrow x=-\dfrac{1}{2}\)
\(e,\left(x+2\right)^2-\left(x+3\right)^2=0\)
\(\Leftrightarrow\left(x+2-x-3\right)\left(x+2+x+3\right)=0\)
\(\Leftrightarrow-\left(2x+5\right)=0\)
\(\Leftrightarrow-2x-5=0\)
\(\Leftrightarrow-2x=5\Rightarrow x=-\dfrac{5}{2}\)
Học tốt nha you <3
Đúng 0
Bình luận (0)
(x-3)^3 +(x+3)^3=0
(x+1)^3-(x-1)^3=0
x^2-4x+3=0
4x^2 +4x +1=0
(x+2)^2-(x+3)^2=0
\(\left(x-3\right)^3+\left(x+3\right)^3=0\)
\(\Leftrightarrow x^3-9x^2+27x-27+x^3+9x^2+27x+27=0\)\(\Leftrightarrow2x^3+54x^2=0\)
\(\Leftrightarrow x^2\left(2x+54\right)=0\)
\(\Rightarrow\left[{}\begin{matrix}x=0\\2x+54=0\end{matrix}\right.\Rightarrow\left[{}\begin{matrix}x=0\\x=-27\end{matrix}\right.\)
\(b,\left(x+1\right)^3-\left(x-1\right)^3=0\)
\(\Leftrightarrow x^3+3x^2+3x+1-x^3+3x^2-3x+1=0\)\(\Leftrightarrow6x^2+2=0\)
\(\Leftrightarrow6x^2=-2\)
\(\Leftrightarrow x^2=-3\) ( vô lí)
Vậy pt vô nghiệm
\(c,x^2-4x+3=0\)
\(\Leftrightarrow x^2-3x-x+3=0\)
\(\Leftrightarrow x\left(x-3\right)-\left(x-3\right)=0\)
\(\Leftrightarrow\left(x-1\right)\left(x-3\right)=0\)
\(\Leftrightarrow\left[{}\begin{matrix}x-1=0\\x-3=0\end{matrix}\right.\Rightarrow\left[{}\begin{matrix}x=1\\x=3\end{matrix}\right.\)
\(d,4x^2+4x+1=0\)
\(\Leftrightarrow\left(2x+1\right)^2=0\)
\(\Rightarrow2x+1=0\)
\(\Leftrightarrow2x=-1\Rightarrow x=-\dfrac{1}{2}\)
\(e,\left(x+2\right)^2-\left(x+3\right)^2=0\)
\(\Leftrightarrow\left(x+2-x-3\right)\left(x+2+x+3\right)=0\)
\(\Leftrightarrow-\left(2x+5\right)=0\)
\(\Leftrightarrow-2x-5=0\)
\(\Leftrightarrow-2x=5\Rightarrow x=-\dfrac{5}{2}\)
Học tốt nha you <3
Đúng 1
Bình luận (0)
Bài 1: Tìm x biết a) x^3 - 4x^2 - x + 4= 0 b) x^3 - 3x^2 + 3x + 1=0 c) x^3 + 3x^2 - 4x - 12=0 d) (x-2)^2 - 4x +8 =0
a: \(x^3-4x^2-x+4=0\)
=>\(\left(x^3-4x^2\right)-\left(x-4\right)=0\)
=>\(x^2\left(x-4\right)-\left(x-4\right)=0\)
=>\(\left(x-4\right)\left(x^2-1\right)=0\)
=>\(\left[{}\begin{matrix}x-4=0\\x^2-1=0\end{matrix}\right.\Leftrightarrow\left[{}\begin{matrix}x=4\\x^2=1\end{matrix}\right.\Leftrightarrow x\in\left\{2;1;-1\right\}\)
b: Sửa đề: \(x^3+3x^2+3x+1=0\)
=>\(x^3+3\cdot x^2\cdot1+3\cdot x\cdot1^2+1^3=0\)
=>\(\left(x+1\right)^3=0\)
=>x+1=0
=>x=-1
c: \(x^3+3x^2-4x-12=0\)
=>\(\left(x^3+3x^2\right)-\left(4x+12\right)=0\)
=>\(x^2\cdot\left(x+3\right)-4\left(x+3\right)=0\)
=>\(\left(x+3\right)\left(x^2-4\right)=0\)
=>\(\left(x+3\right)\left(x-2\right)\left(x+2\right)=0\)
=>\(\left[{}\begin{matrix}x+3=0\\x-2=0\\x+2=0\end{matrix}\right.\Leftrightarrow\left[{}\begin{matrix}x=-3\\x=2\\x=-2\end{matrix}\right.\)
d: \(\left(x-2\right)^2-4x+8=0\)
=>\(\left(x-2\right)^2-\left(4x-8\right)=0\)
=>\(\left(x-2\right)^2-4\left(x-2\right)=0\)
=>\(\left(x-2\right)\left(x-2-4\right)=0\)
=>(x-2)(x-6)=0
=>\(\left[{}\begin{matrix}x-2=0\\x-6=0\end{matrix}\right.\Leftrightarrow\left[{}\begin{matrix}x=2\\x=6\end{matrix}\right.\)
Đúng 0
Bình luận (0)
a,x^2-9x+20=0
b,x^3-4x^2+5x=0
c,x^2=2x-15=0
d,(x^2-1)^2=4x+1
e,4x^3-9x^2+6x-1=0
f,x^4-4x^3-x^2+16x-12=0
a) Ta có: \(x^2-9x+20=0\)
\(\Leftrightarrow x^2-5x-4x+20=0\)
\(\Leftrightarrow x\left(x-5\right)-4\left(x-5\right)=0\)
\(\Leftrightarrow\left(x-5\right)\left(x-4\right)=0\)
\(\Leftrightarrow\left[{}\begin{matrix}x-5=0\\x-4=0\end{matrix}\right.\Leftrightarrow\left[{}\begin{matrix}x=5\\x=4\end{matrix}\right.\)
Vậy: x∈{4;5}
b) Ta có: \(x^3-4x^2+5x=0\)
\(\Leftrightarrow x\left(x^2-4x+5\right)=0\)(1)
Ta có: \(x^2-4x+5\)
\(=x^2-4x+4+1=\left(x-2\right)^2+1\)
Ta có: \(\left(x-2\right)^2\ge0\forall x\)
\(\Rightarrow\left(x-2\right)^2+1\ge1>0\forall x\)
hay \(x^2-4x+5>0\forall x\)(2)
Từ (1) và (2) suy ra x=0
Vậy: x=0
c) Sửa đề: \(x^2-2x-15=0\)
Ta có: \(x^2-2x-15=0\)
\(\Leftrightarrow x^2+3x-5x-15=0\)
\(\Leftrightarrow x\left(x+3\right)-5\left(x+3\right)=0\)
\(\Leftrightarrow\left(x+3\right)\left(x-5\right)=0\)
\(\Leftrightarrow\left[{}\begin{matrix}x+3=0\\x-5=0\end{matrix}\right.\Leftrightarrow\left[{}\begin{matrix}x=-3\\x=5\end{matrix}\right.\)
Vậy: x∈{-3;5}
d) Ta có: \(\left(x^2-1\right)^2=4x+1\)
\(\Leftrightarrow x^4-2x^2+1-4x-1=0\)
\(\Leftrightarrow x^4-2x^2-4x=0\)
\(\Leftrightarrow x\left(x^3-2x-4\right)=0\)
\(\Leftrightarrow x\left(x^3+2x^2+2x-2x^2-4x-4\right)=0\)
\(\Leftrightarrow x\cdot\left[x\left(x^2+2x+2\right)-2\left(x^2+2x+2\right)\right]=0\)
\(\Leftrightarrow x\cdot\left(x^2+2x+2\right)\cdot\left(x-2\right)=0\)(3)
Ta có: \(x^2+2x+2\)
\(=x^2+2x+1+1=\left(x+1\right)^2+1\)
Ta có: \(\left(x+1\right)^2\ge0\forall x\)
\(\Rightarrow\left(x+1\right)^2+1\ge1>0\forall x\)
hay \(x^2+2x+2>0\forall x\)(4)
Từ (3) và (4) suy ra
\(\left[{}\begin{matrix}x=0\\x-2=0\end{matrix}\right.\Leftrightarrow\left[{}\begin{matrix}x=0\\x=2\end{matrix}\right.\)
Vậy: x∈{0;2}
a)x2+4x+3=0b)4x2+4x-3=0c)x2-x-12=0d)x2-25-(x-5)=0e)(2x-1)2-4x=-1g)x2(x2+1)-x2-1=0
Xem chi tiết
\(x^2+4x+3=0\)
\(x^2+x+3x+3=0\)
\(x\left(x+1\right)+3\left(x+1\right)=0\)
\(\left(x+1\right)\left(x+3\right)=0\)
\(\left[\begin{array}{nghiempt}x+1=0\\x+3=0\end{array}\right.\)
\(\left[\begin{array}{nghiempt}x=-1\\x=-3\end{array}\right.\)
\(4x^2+4x-3=0\)
\(4x^2-2x+6x-3=0\)
\(2x\left(2x-1\right)+3\left(2x-1\right)=0\)
\(\left(2x-1\right)\left(2x+3\right)=0\)
\(\left[\begin{array}{nghiempt}2x-1=0\\2x+3=0\end{array}\right.\)
\(\left[\begin{array}{nghiempt}2x=1\\2x=-3\end{array}\right.\)
\(\left[\begin{array}{nghiempt}x=\frac{1}{2}\\x=-\frac{3}{2}\end{array}\right.\)
\(x^2-x-12=0\)
\(x^2-4x+3x-12=0\)
\(x\left(x-4\right)+3\left(x-4\right)=0\)
\(\left(x-4\right)\left(x+3\right)=0\)
\(\left[\begin{array}{nghiempt}x-4=0\\x+3=0\end{array}\right.\)
\(\left[\begin{array}{nghiempt}x=4\\x=-3\end{array}\right.\)
\(x^2-25-\left(x-5\right)=0\)
\(\left(x-5\right)\left(x+5\right)-\left(x-5\right)=0\)
\(\left(x-5\right)\left(x+5-1\right)=0\)
\(\left(x-5\right)\left(x+4\right)=0\)
\(\left[\begin{array}{nghiempt}x-5=0\\x+4=0\end{array}\right.\)
\(\left[\begin{array}{nghiempt}x=5\\x=-4\end{array}\right.\)
\(x^2\left(x^2+1\right)-x^2-1=0\)
\(x^2\left(x^2+1\right)-\left(x^2+1\right)=0\)
\(\left(x^2+1\right)\left(x^2-1\right)=0\)
\(\left(x^2+1\right)\left(x-1\right)\left(x+1\right)=0\)
\(\left[\begin{array}{nghiempt}x-1=0\\x+1=0\end{array}\right.\) (vì \(x^2+1\ge1>0\))
\(\left[\begin{array}{nghiempt}x=1\\x=-1\end{array}\right.\)
Đúng 0
Bình luận (0)
Bài 1: Giải phương trình và bất phương trình sau: 1. 5.(2-3x). (x-2) = 3.( 1-3x) 2. 4x^2 + 4x + 1= 0 3. 4x^2 - 9= 0 4. 5x^2 - 10=0 5. x^2 - 3x= -2 6. |x-5| - 3= 0
Tìm x
a) 4x(x + 1) = 8(x + 1)
b) x(x – 1) – 2(1 – x) = 0
c) 5x(x – 2) – (2 – x) = 0
d) 5x(x – 200) – x + 200 = 0
e) x3 + 4x = 0
f) (x + 1) = (x + 1)2
a) 4x(x+1)=8(x+1)
<=>4x(x+1)-8(x+1)=0
<=>(4x-8)(x+1)=0
<=>\(\left[\begin{array}{} 4x-8=0\\ x+1=0 \end{array} \right.\)
<=>\(\left[\begin{array}{} x=2\\ x=-1 \end{array} \right.\)
Vậy...
b)x(x-1)-2(1-x)=0
<=>(x+2)(x-1)=0
<=>\(\left[\begin{array}{} x+2=0\\ x-1=0 \end{array} \right.\)
<=>\(\left[\begin{array}{} x=-2\\ x=1 \end{array} \right.\)
Vậy...
c)5x(x-2)-(2-x)=0
<=>(5x+1)(x-2)=0
<=>\(\left[\begin{array}{} 5x+1=0\\ x-2 \end{array} \right.\)
<=>\(\left[\begin{array}{} x=-1/5\\ x=2 \end{array} \right.\)
d)5x(x-200)-x+200=0
<=>(5x-1)(x-200)=0
<=>\(\left[\begin{array}{} 5x-1=0\\ x-200=0 \end{array} \right.\)
<=>\(\left[\begin{array}{} x=1/5\\ x=200 \end{array} \right.\)
e)\(x^3+4x=0 \)
\(\Leftrightarrow x(x^2+4)=0 \)
\(\Leftrightarrow \left[\begin{array}{} x=0\\ x^2+4=0 (loại vì x^2+4>=0 với mọi x) \end{array} \right.\)
Vậy x=0
f)\((x+1)=(x+1)^2\)
\(\Leftrightarrow (x+1)-(x+1)^2=0\)
\(\Leftrightarrow (x+1)(1-x-1)=0\)
\(\Leftrightarrow (x+1)(-x)=0\)
\(\Leftrightarrow \left[\begin{array}{} x=-1\\ x=0 \end{array} \right.\)
Vậy....
Đúng 1
Bình luận (0)
Giải phương trình:1. x^4-6x^2-12x-802. dfrac{x}{2x^2+4x+1}+dfrac{x}{2x^2-4x+1}dfrac{3}{5}3. x^4-x^3-8x^2+9x-9+left(x^2-x+1right)sqrt{x+9}04. 2x^2.sqrt{-4x^4+4x^2+3}4x^4+15. x^2+4x+3sqrt{dfrac{x}{8}+dfrac{1}{2}}6. left{{}begin{matrix}4x^3+xy^23x-y4xy+y^22end{matrix}right.7. left{{}begin{matrix}sqrt{x^2-3y}left(2x+y+1right)+2x+y-505x^2+y^2+4xy-3y-50end{matrix}right.8. left{{}begin{matrix}sqrt{2x^2+2}+left(x^2+1right)^2+2y-100left(x^2+1right)^2+x^2yleft(y-4right)0end{matrix}right.
Đọc tiếp
Giải phương trình:
1. \(x^4-6x^2-12x-8=0\)
2. \(\dfrac{x}{2x^2+4x+1}+\dfrac{x}{2x^2-4x+1}=\dfrac{3}{5}\)
3. \(x^4-x^3-8x^2+9x-9+\left(x^2-x+1\right)\sqrt{x+9}=0\)
4. \(2x^2.\sqrt{-4x^4+4x^2+3}=4x^4+1\)
5. \(x^2+4x+3=\sqrt{\dfrac{x}{8}+\dfrac{1}{2}}\)
6. \(\left\{{}\begin{matrix}4x^3+xy^2=3x-y\\4xy+y^2=2\end{matrix}\right.\)
7. \(\left\{{}\begin{matrix}\sqrt{x^2-3y}\left(2x+y+1\right)+2x+y-5=0\\5x^2+y^2+4xy-3y-5=0\end{matrix}\right.\)
8. \(\left\{{}\begin{matrix}\sqrt{2x^2+2}+\left(x^2+1\right)^2+2y-10=0\\\left(x^2+1\right)^2+x^2y\left(y-4\right)=0\end{matrix}\right.\)
1.
\(x^4-6x^2-12x-8=0\)
\(\Leftrightarrow x^4-2x^2+1-4x^2-12x-9=0\)
\(\Leftrightarrow\left(x^2-1\right)^2=\left(2x+3\right)^2\)
\(\Leftrightarrow\left[{}\begin{matrix}x^2-1=2x+3\\x^2-1=-2x-3\end{matrix}\right.\)
\(\Leftrightarrow\left[{}\begin{matrix}x^2-2x-4=0\\x^2+2x+2=0\end{matrix}\right.\)
\(\Leftrightarrow x=1\pm\sqrt{5}\)
Đúng 5
Bình luận (0)
3.
ĐK: \(x\ge-9\)
\(x^4-x^3-8x^2+9x-9+\left(x^2-x+1\right)\sqrt{x+9}=0\)
\(\Leftrightarrow\left(x^2-x+1\right)\left(\sqrt{x+9}+x^2-9\right)=0\)
\(\Leftrightarrow\sqrt{x+9}+x^2-9=0\left(1\right)\)
Đặt \(\sqrt{x+9}=t\left(t\ge0\right)\Rightarrow9=t^2-x\)
\(\left(1\right)\Leftrightarrow t+x^2+x-t^2=0\)
\(\Leftrightarrow\left(x+t\right)\left(x-t+1\right)=0\)
\(\Leftrightarrow\left[{}\begin{matrix}x=-t\\x=t-1\end{matrix}\right.\)
\(\Leftrightarrow\left[{}\begin{matrix}x=-\sqrt{x+9}\\x=\sqrt{x+9}-1\end{matrix}\right.\)
\(\Leftrightarrow...\)
Đúng 5
Bình luận (2)
2.
ĐK: \(x\ne\dfrac{2\pm\sqrt{2}}{2};x\ne\dfrac{-2\pm\sqrt{2}}{2}\)
\(\dfrac{x}{2x^2+4x+1}+\dfrac{x}{2x^2-4x+1}=\dfrac{3}{5}\)
\(\Leftrightarrow\dfrac{1}{2x+\dfrac{1}{x}+4}+\dfrac{1}{2x+\dfrac{1}{x}-4}=\dfrac{3}{5}\)
Đặt \(2x+\dfrac{1}{x}+4=a;2x+\dfrac{1}{x}-4=b\left(a,b\ne0\right)\)
\(pt\Leftrightarrow\dfrac{1}{a}+\dfrac{1}{b}=\dfrac{3}{5}\left(1\right)\)
Lại có \(a-b=8\Rightarrow a=b+8\), khi đó:
\(\left(1\right)\Leftrightarrow\dfrac{1}{b+8}+\dfrac{1}{b}=\dfrac{3}{5}\)
\(\Leftrightarrow\dfrac{2b+8}{\left(b+8\right)b}=\dfrac{3}{5}\)
\(\Leftrightarrow10b+40=3\left(b+8\right)b\)
\(\Leftrightarrow\left[{}\begin{matrix}b=2\\b=-\dfrac{20}{3}\end{matrix}\right.\)
TH1: \(b=2\Leftrightarrow...\)
TH2: \(b=-\dfrac{20}{3}\Leftrightarrow...\)
Đúng 3
Bình luận (0)
Xem thêm câu trả lời
Giải các bất phương trình sau:
a) \(2{x^2} + 3x + 1 \ge 0\)
b) \( - 3{x^2} + x + 1 > 0\)
c) \(4{x^2} + 4x + 1 \ge 0\)
d) \( - 16{x^2} + 8x - 1 < 0\)
e) \(2{x^2} + x + 3 < 0\)
g) \( - 3{x^2} + 4x - 5 < 0\)
a) \(2{x^2} + 3x + 1 \ge 0\)
Tam thức bậc hai \(f\left( x \right) = 2{x^2} + 3x + 1\) có 2 nghiệm phân biệt \(x = - 1,x = \frac{{ - 1}}{2}\)
hệ số \(a = 2 > 0\)
Ta có bảng xét dấu f(x) như sau:
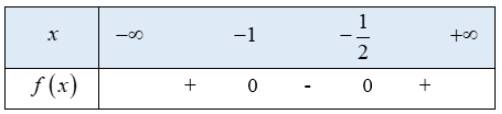
Từ bảng xét dấu ta thấy \(f\left( x \right) \ge 0 \Leftrightarrow \left[ \begin{array}{l}x \le - 1\\x \ge - \frac{1}{2}\end{array} \right.\)
Vậy tập nghiệm của bất phương trình là \(\left( { - \infty ; - 1} \right] \cup \left[ { - \frac{1}{2}; + \infty } \right)\)
b) \( - 3{x^2} + x + 1 > 0\)
Tam thức bậc hai \(f\left( x \right) = - 3{x^2} + x + 1\) có 2 nghiệm phân biệt \(x = \frac{{1 - \sqrt {13} }}{6},x = \frac{{1 + \sqrt {13} }}{6}\)
Hệ số \(a = - 3 < 0\)
Ta có bảng xét dấu f(x) như sau:
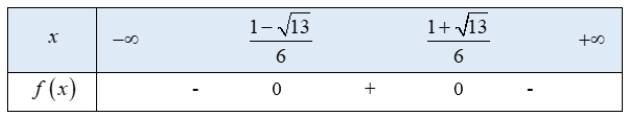
Từ bảng xét dấu ta thấy \(f\left( x \right) > 0\)\( \Leftrightarrow \frac{{1 - \sqrt {13} }}{6} < x < \frac{{1 + \sqrt {13} }}{6}\)
Vậy tập nghiệm của bất phương trình là \(\left( {\frac{{1 - \sqrt {13} }}{6};\frac{{1 + \sqrt {13} }}{6}} \right)\)
c) \(4{x^2} + 4x + 1 \ge 0\)
Tam thức bậc hai \(f\left( x \right) = 4{x^2} + 4x + 1\) có nghiệm duy nhất \(x = \frac{{ - 1}}{2}\)
hệ số \(a = 4 > 0\)
Ta có bảng xét dấu f(x) như sau:

Từ bảng xét dấu ta thấy \(f\left( x \right) \ge 0 \Leftrightarrow x \in \mathbb{R}\)
Vậy tập nghiệm của bất phương trình là \(\mathbb{R}\)
d) \( - 16{x^2} + 8x - 1 < 0\)
Tam thức bậc hai \(f\left( x \right) = - 16{x^2} + 8x - 1\) có nghiệm duy nhất \(x = \frac{1}{4}\)
hệ số \(a = - 16 < 0\)
Ta có bảng xét dấu f(x) như sau:
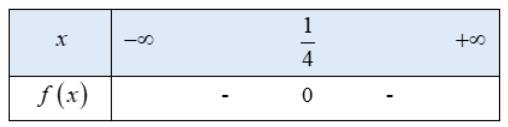
Từ bảng xét dấu ta thấy \(f\left( x \right) < 0 \Leftrightarrow x \ne \frac{1}{4}\)
Vậy tập nghiệm của bất phương trình là \(\mathbb{R}\backslash \left\{ {\frac{1}{4}} \right\}\)
e) \(2{x^2} + x + 3 < 0\)
Ta có \(\Delta = {1^2} - 4.2.3 = - 23 < 0\) và có \(a = 2 > 0\)
Sử dụng định lí về dấu của tam thức bậc hai, ta thấy tập hợp những giá trị của x sao cho \(2{x^2} + x + 3\) mang dấu “-” là \(\emptyset \)
Vậy tập nghiệm của bất phương trình \(2{x^2} + x + 3 < 0\) là \(\emptyset \)
g) \( - 3{x^2} + 4x - 5 < 0\)
Tam thức bậc hai \(f\left( x \right) = - 3{x^2} + 4x - 5\) có \(\Delta ' = {2^2} - \left( { - 3} \right).\left( { - 5} \right) = - 11 < 0\) và có \(a = - 3 < 0\)
Sử dụng định lí về dấu của tam thức bậc hai, ta thấy tập hợp những giá trị của x sao cho \( - 3{x^2} + 4x - 5\) mang dấu “-” là \(\mathbb{R}\)
Vậy tập nghiệm của bất phương trình \( - 3{x^2} + 4x - 5 < 0\) là \(\mathbb{R}\)
Đúng 0
Bình luận (0)


