a: \(A=\dfrac{2x^2+x^2-1-2x^2+2x+1}{x\left(x+1\right)}=\dfrac{x^2+2x}{x\left(x+1\right)}=\dfrac{x+2}{x+1}\)

\(\dfrac{7x-2}{3}-2x< 5-\dfrac{x-2}{4}\)

<=>\(\dfrac{4\left(7x-2\right)}{12}-\dfrac{24x}{12}< \dfrac{60}{12}-\dfrac{3\left(x-2\right)}{12}\)

<=>\(4\left(7x-2\right)-24x< 60-3\left(x-2\right)\)

<=>\(28x-8-24x< 60-3x+6\)

<=>\(28x+3x-24x< 60+8+6\)

<=>\(7x< 74\)

<=>x<\(\dfrac{74}{7}\)

Vậy...

Câu 1:

c. 

PT $(1)\Leftrightarrow x=1+2my$. Thay vô PT $(2)$:
$m(1+2my)+y=2$
$\Leftrightarrow y(2m^2+1)=2-m$

$\Leftrightarrow y=\frac{2-m}{2m^2+1}$

$x=1+2my=1+\frac{4m-2m^2}{2m^2+1}=\frac{4m+1}{2m^2+1}$
Vậy hpt có nghiệm duy nhất $(x,y)=(\frac{4m+1}{2m^2+1}, \frac{2-m}{2m^2+1})$
Để $x,y$ nguyên thì:
$4m+1\vdots 2m^2+1$ và $2-m\vdots 2m^2+1$

$\Rightarrow 4m+1+4(2-m)\vdots 2m^2+1$
$\Leftrightarrow 9\vdots 2m^2+1$

$\Rightarrow 2m^2+1\in\left\{1;3;9\right\}$

$\Rightarrow m\in\left\{0; 1; -1;2;-2\right\}$

Thử lại thì thấy $m=0; -1;2$ thỏa mãn.

\(\frac{a}{2}=\frac{b}{5}=\frac{c}{8}\)

\(=>\frac{a}{2}=\frac{b}{5}=\frac{2c}{16}\)

ÁP DỤNG T/C DÃY TỈ SỐ BẰNG NHAY, TA CÓ:

\(\frac{a}{2}=\frac{b}{5}=\frac{c}{8}=\frac{2c}{16}=\frac{a-b+2c}{2-5+16}=\frac{6}{13}\)

\(\frac{a}{2}=\frac{6}{13}=>a=\frac{12}{13}\)

\(\frac{b}{5}=\frac{6}{13}=>b=\frac{30}{13}\)

\(\frac{c}{8}=\frac{6}{13}=>c=\frac{48}{13}\)

Vậy a=...

b=...

c=...

b: B=418-3253+253+582

=1000-3000

=-2000

c: \(\Leftrightarrow x^2-5x-x^2-7< =0\)

=>-5x<=7

hay x>=-7/5

d: \(\Leftrightarrow x^2-x-2+3-x^2>=0\)

=>-x+1>=0

=>-x>=-1

hay x<=1

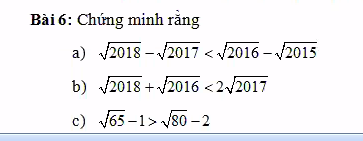
a) Ta có: \(\sqrt{2018}-\sqrt{2017}=\dfrac{1}{\sqrt{2018}+\sqrt{2017}}\)

\(\sqrt{2016}-\sqrt{2015}=\dfrac{1}{\sqrt{2016}+\sqrt{2015}}\)

mà \(\dfrac{1}{\sqrt{2018}+\sqrt{2017}}< \dfrac{1}{\sqrt{2016}+\sqrt{2015}}\)

nên \(\sqrt{2018}-\sqrt{2017}< \sqrt{2016}-\sqrt{2015}\)

b) Ta có: \(\left(\sqrt{2018}+\sqrt{2016}\right)^2=4034+24\sqrt{14126}\)

\(\left(2\sqrt{2017}\right)^2=8068=4034+4034\)

mà \(24\sqrt{14126}< 4034\)

nên \(\sqrt{2018}+\sqrt{2016}< 2\sqrt{2017}\)