\(\left\{{}\begin{matrix}\sqrt{5x^2+2xy+2y^2}+\sqrt{2x^2+2xy+5y^2}=3\left(x+y\right)\\\sqrt{2x+y+1}+2\sqrt[3]{7x+12y+8}=2xy+y+5\end{matrix}\right.\)

Xét \(pt\left(1\right)\) dễ dàng suy ra \(x+y\ge0\)

\(VT=\sqrt{\left(x-y\right)^2+\left(2x+y\right)^2}+\sqrt{\left(x-y\right)^2+\left(2y+x\right)^2}\)

\(\ge\left|2x+y\right|+\left|2y+x\right|\ge3\left(x+y\right)\)

Đẳng thức xảy ra khi \(\left\{{}\begin{matrix}x=y\\x,y\ge0\end{matrix}\right.\)

Thay vào \(pt\left(2\right)\) ta được:

\(\sqrt{3x+1}+2\sqrt[3]{19x+8}=2x^2+x+5\)

\(\Leftrightarrow\left[\sqrt{3x+1}-\left(x+1\right)\right]+2\left[\sqrt[3]{19x+8}-\left(x+2\right)\right]=2x^2-2x\)

\(\Leftrightarrow\left(x-x^2\right)\left[\dfrac{1}{\sqrt{3x+1}+x+1}+2\cdot\dfrac{x+7}{\sqrt[3]{\left(19x+8\right)^2}+\left(x+2\right)\sqrt[3]{19x+8}+\left(x+2\right)^2}+2\right]=0\)

Do \(x;y\ge0\) nên pt trong ngoặc luôn dương

\(\Rightarrow x-x^2=0\Rightarrow x\left(1-x\right)=0\Rightarrow\)\(\left[{}\begin{matrix}x=0\\x=1\end{matrix}\right.\)

Mà \(x=y\)\(\Rightarrow\left[{}\begin{matrix}x=y=0\\x=y=1\end{matrix}\right.\) là nghiệm của hpt

thanks b đã chỉ giúp mình.tại đánh máy nên mình ko để ý^^

pt(1): 5x²+2xy+2y²>=(2x+y)² nên \(\sqrt{5x^{2^{ }}+2xy+2y^2}\ge\:\)trị tuyệt đối 2x+y.

cmtt>\(\sqrt{2x^2+2xy+5y^2}\ge\)trị tuyệt đối x+ 2y.

>mà tt đối 2x+y cộng ttđ x+2y>= 3(x+y).

>(1)>=3(x+y).

đâu = xảy ra khi và chỉ khi x=y.

thay x=y >=0 vào (2):

\(\sqrt{3x+1}+2\sqrt[3]{19x+8}\) = 2x²+x+5.

<=>\(\left(\sqrt{3x+1}-\left(x+1\right)\right)\)+\(\left(2\sqrt[3]{19x+8}-\left(x+2\right)\right)\)= 2x²- 2x.

nhân liên hợp ta đc:

(x²-x)*(\(\dfrac{1}{\sqrt{3x+1}+x+1}+2\dfrac{x+7}{\sqrt[3]{19x+18}+\left(x+2\right)\left(\sqrt[3]{19x+18}\right)+\left(x+2\right)^2}=0\)

dễ thấy phần *>0 với mọi x,ytheo đk của (1)

>(x² -x)=0

>x=0 hoặc x=1

>(x,y)=(0,0); (1,1).

vậy....

a) \(\left\{{}\begin{matrix}2x+3y=5\\4x-5y=1\end{matrix}\right.\)

\(\Leftrightarrow\left\{{}\begin{matrix}4x+6y=10\\4x-5y=1\end{matrix}\right.\)

\(\Leftrightarrow\left\{{}\begin{matrix}2x+3y=5\\11y=9\end{matrix}\right.\)

\(\Leftrightarrow\left\{{}\begin{matrix}2x+3\cdot\dfrac{9}{11}=5\\y=\dfrac{9}{11}\end{matrix}\right.\)

\(\Leftrightarrow\left\{{}\begin{matrix}2x+\dfrac{27}{11}=5\\y=\dfrac{9}{11}\end{matrix}\right.\)

\(\Leftrightarrow\left\{{}\begin{matrix}2x=\dfrac{28}{11}\\y=\dfrac{9}{11}\end{matrix}\right.\)

\(\Leftrightarrow\left\{{}\begin{matrix}x=\dfrac{14}{11}\\y=\dfrac{9}{11}\end{matrix}\right.\)

Vậy: \(x=\dfrac{14}{11};y=\dfrac{9}{11}\)

Đề có vẻ sai sai. Bạn xem lại đề xem có đúng không?

2) 

a) ĐK: \(2x^2-8x-12\ge0\)(1)

Nhân 2 cả hai vế ta có:

\(2x^2-8x-12=2\sqrt{2x^2-8x-12}\)

Đặt: \(\sqrt{2x^2-8x-12}=t\left(t\ge0\right)\)

Ta có phương trình: \(t^2=2t\Leftrightarrow\orbr{\begin{cases}t=0\\t=2\end{cases}}\)(tm)

+) Với t=0  ta có:\(\sqrt{2x^2-8x-12}=0\Leftrightarrow2x^2-8x-12=0\Leftrightarrow x^2-4x-6=0\Leftrightarrow\orbr{\begin{cases}x=2+\sqrt{10}\\x=2-\sqrt{10}\end{cases}}\)( thỏa mãn đk (1))

+) Với t=2 ta có: \(\sqrt{2x^2-8x-12}=2\Leftrightarrow2x^2-8x-12=4\Leftrightarrow x^2-4x-8=\Leftrightarrow\orbr{\begin{cases}x=2+2\sqrt{3}\\x=2-2\sqrt{3}\end{cases}}\)( THỎA MÃN đk (1))

vậy ...

b) pt <=> \(\left(4x+1\right)\left(3x+2\right)\left(12x-1\right)\left(x+1\right)=4\)

<=> \(\left(12x^2+11x+2\right)\left(12x^2+11x-1\right)=4\)

Đặt :\(12x^2+11x+2=t\)

Ta có pt: \(t\left(t-3\right)=4\Leftrightarrow t^2-3t-4=0\Leftrightarrow\orbr{\begin{cases}t=4\\t=-1\end{cases}}\)

Với t=4 ta có: ....

Với t=-1 ta có:...

Em tự làm tiếp nhé

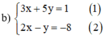
Từ (2) ta rút ra được y = 2x + 8 (*)

Thế (*) vào phương trình (1) ta được :

3x + 5(2x + 8) = 1 ⇔ 3x + 10x + 40 = 1 ⇔ 13x = -39 ⇔ x = -3.

Thay x = - 3 vào (*) ta được y = 2.(-3) + 8 = 2.

Vậy hệ phương trình có nghiệm duy nhất (-3 ; 2).

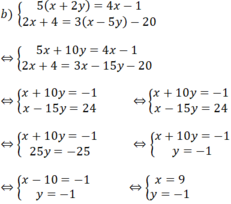
Vậy hệ phương trình đã cho có nghiệm (x; y) = (9; -1).