Họ nguyên hàm của hàm số f(x) = sin3x là
A. - 1 3 cos 3 x + C
B. 1 3 cos 3 x + C
C. 3 cos 3 x + C
D. - 3 cos 3 x + C
Tìm họ nguyên hàm của các hàm số lượng giác sau :
a) \(f\left(x\right)=\sin^3x.\sin3x\)
b) \(f\left(x\right)=\sin^3x.\cos3x+\cos^3x.\sin3x\)
a) \(f\left(x\right)=\sin^3x.\sin3x=\sin3x\left(\frac{3\sin x-\sin3x}{4}\right)=\frac{3}{4}\sin3x.\sin x-\frac{1}{4}\sin^23x\)
= \(\frac{3}{8}\left(\cos2x-\cos4x\right)-\frac{1}{8}\left(1-\cos6x\right)=\frac{3}{8}\cos2x+\frac{1}{8}\cos6x-\frac{3}{8}\cos4x-\frac{1}{8}\)
Do đó :
\(I=\int f\left(x\right)dx=\int\left(\frac{3}{8}\cos2x+\frac{1}{8}\cos6x-\frac{3}{8}\cos4x-\frac{1}{8}\right)dx=\frac{3}{16}\sin2x+\frac{1}{48}\sin6x-\frac{3}{32}\sin4x-\frac{1}{8}x+C\)
b) Ta biến đổi :
\(f\left(x\right)=\sin^3x.\cos3x+\cos^3x.\sin3x=\cos3x\left(\frac{3\sin x-\sin3x}{4}\right)+\sin3x\left(\frac{\cos3x+3\cos x}{4}\right)\)
\(=\frac{3}{4}\left(\cos3x\sin x+\sin3x\cos x\right)=\frac{3}{4}\sin4x\)
Do đó : \(I=\int f\left(x\right)dx=\frac{3}{4}\int\sin4xdx=-\frac{3}{16}\cos4x+C\)
Tìm họ nguyên hàm của hàm số: f(x)=cos(2x+3)
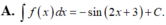
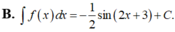
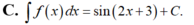
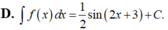
Tìm họ nguyên hàm của hàm số :
\(f\left(x\right)=\frac{4\sin^2x+1}{\sqrt{3}\sin x+\cos x}\)
Biến đổi :
\(4\sin^2x+1=5\sin^2x+\cos^2x=\left(a\sin x+b\cos x\right)\left(\sqrt{3}\sin x+\cos x\right)+c\left(\sin^2x+\cos^2x\right)\)
\(=\left(a\sqrt{3}+c\right)\sin^2x+\left(a+b\sqrt{3}\right)\sin x.\cos x+\left(b+c\right)\cos^2x\)
Đồng nhấtheej số hai tử số
\(\begin{cases}a\sqrt{3}+c=5\\a+b\sqrt{3}=0\\b+c=1\end{cases}\)
\(\Leftrightarrow\) \(\begin{cases}a=\sqrt{3}\\b=-1\\c=2\end{cases}\)
Tìm họ nguyên hàm của hàm số :
\(f\left(x\right)=\frac{\sin3x\sin4x}{\tan x+\cot2x}\)
Ta biến đổi :
\(f\left(x\right)=\frac{\sin3x\sin4x}{\tan x+\cot2x}=\frac{\sin3x\sin4x}{\frac{\sin x.\sin2x+\cos x.\cos2x}{\cos x.\sin2x}}=\frac{\sin3x\sin4x}{\frac{\cos x}{\cos x.\sin2x}}=\sin3x\sin4x\sin2x\)
\(=\frac{1}{2}\left(\cos x-\cos7x\right)\sin2x=\frac{1}{2}\left[\sin2x\cos x-\cos7x\sin2x\right]=\frac{1}{4}\left(\sin3x+\sin x-\sin9x+\sin5x\right)\)
Do đó :
\(I=\int\left(\frac{1}{4}\left(\sin3x+\sin x-\sin9x+\sin5x\right)\right)dx=-\frac{1}{2}\cos3x-\frac{1}{4}\cos x+\frac{1}{9}\cos9x-\frac{1}{5}\cos5x+C\)
Tìm họ nguyên hàm của hàm số lượng giác sau :
\(f\left(x\right)=\int\frac{4\sin x+3\cos x}{\sin x+2\cos x}dx\)
Biến đổi :
\(4\sin x+3\cos x=A\left(\sin x+2\cos x\right)+B\left(\cos x-2\sin x\right)=\left(A-2B\right)\sin x+\left(2A+B\right)\cos x\)
Đồng nhất hệ số hai tử số, ta có :
\(\begin{cases}A-2B=4\\2A+B=3\end{cases}\)\(\Leftrightarrow\begin{cases}A=2\\B=-1\end{cases}\)
Khi đó \(f\left(x\right)=\frac{2\left(\left(\sin x+2\cos x\right)\right)-\left(\left(\sin x-2\cos x\right)\right)}{\left(\sin x+2\cos x\right)}=2-\frac{\cos x-2\sin x}{\sin x+2\cos x}\)
Do đó,
\(F\left(x\right)=\int f\left(x\right)dx=\int\left(2-\frac{\cos x-2\sin x}{\sin x+2\cos x}\right)dx=2\int dx-\int\frac{\left(\cos x-2\sin x\right)dx}{\sin x+2\cos x}=2x-\ln\left|\sin x+2\cos x\right|+C\)
Tìm họ nguyên hàm của hàm số
\(f\left(x\right)=\frac{2}{\sqrt{3}\sin x+\cos x}\)
Ta có :
\(f\left(x\right)=\int\frac{dx}{\sqrt{3}\sin x+\cos x}=\frac{1}{2}\int\frac{dx}{\frac{\sqrt{3}}{2}\sin x+\frac{1}{2}\cos x}=\frac{1}{2}\int\frac{dx}{\sin\left(x+\frac{\pi}{6}\right)}\)
\(=\int\frac{dx}{2\tan\left(\frac{x}{2}+\frac{\pi}{12}\right)\cos^2\left(\frac{x}{2}+\frac{\pi}{12}\right)}=\int\frac{dx}{\sin\left(\frac{x}{2}+\frac{\pi}{12}\right)\cos\left(\frac{x}{2}+\frac{\pi}{12}\right)}=\int\frac{d\left(\tan\frac{x}{2}+\frac{\pi}{12}\right)}{\tan\left(\frac{x}{2}+\frac{\pi}{12}\right)}=\ln\left|\tan\left(\frac{x}{2}+\frac{\pi}{12}\right)\right|+C\)
Cho hàm số F(x) là một nguyên hàm của hàm số f ( x ) = sin 3 x . cos x . Tính I = F π 2 - F ( 0 )

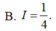
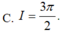
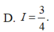
Họ nguyên hàm của hàm số f ( x ) = x 2 + 2 x - 3 ( x + 1 ) 2 là
![]()
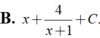
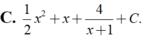
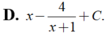
Nguyên hàm của hàm số f ( x ) = cos ( 2 x - 3 ) là
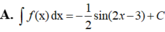
![]()
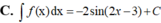
![]()