Tìm x, biết:12x3-4x(3x2+2)=24
Giúp mik với
Tìm P biết P + 4 x − 12 x 3 − 3 x 2 − 4 x + 12 = 3 x − 3 − x 2 4 − x 2
A. P = x x + 3
B. P = x x - 3
C. P = 2 x x − 3
D. P = x − 3 x
Tìm P biết: P + 4x − 12 x 3 − 3x 2 − 4x + 12 = 3 x − 3 − x 2 4 − x 2
A. P = x x + 3
B. P = x x − 3
C. P = 1 x − 3
D. P = x-3 x
Tính giá trị của biểu thức D = 3 x 2 + 6 x + 12 x 3 - 8 với x = 4001 2000
Điều kiện xác định của phân thức: x ≠ 2
Ta có 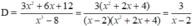
Với 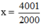 thỏa mãn điều kiện xác định của phân thức
thỏa mãn điều kiện xác định của phân thức
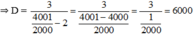
Tìm a, b biết:
a+b= 192 và ƯCLN( a,b) = 24
giúp mik với
TK:
Giải thích các bước giải:
ƯCLN(a;b)=24 nên a và b cùng chia hết cho 24
Đặt a=24m; b=24n, khi đó ƯCLN(m;n)=1
Ta có:
a+b=192
24m+24n=192
m+n=8
Do ƯCLN(m;n)=1 nên m=1;n=7 hoặc m=3; n=5 và các hoán vị
Vậy 2 số cần tìm là 24 và 168 hoặc 72 và 120
Phân Tích đa thức sau thành nhân tử
a)X2.(X2+4)-X2-4
b)(X2+X)2+4x2+4x-12
c)(x+2).(x+3).(x+4).(x+5)-24
Giúp e với ạ
a) \(x^2\left(x^2+4\right)-x^2-4=x^2\left(x^2+4\right)-\left(x^2+4\right)=\left(x^2+4\right)\left(x^2-1\right)=\left(x^2+4\right)\left(x-1\right)\left(x+1\right)\)
b) \(\left(x^2+x\right)^2+4x^2+4x-12=\left(x^2+x\right)^2+4\left(x^2+x\right)+4-16=\left(x^2+x+2\right)^2-4^2=\left(x^2+x+2-4\right)\left(x^2+x+2+4\right)=\left(x^2+x-2\right)\left(x^2+x+6\right)=\left(x-1\right)\left(x+2\right)\left(x^2+x+6\right)\)
c) \(\left(x+2\right)\left(x+3\right)\left(x+4\right)\left(x+5\right)-24=\left(x^2+7x+10\right)\left(x^2+7x+12\right)-24=\left(x^2+7x+10\right)^2+2\left(x^2+7x+10\right)+1-25=\left(x^2+7x+11\right)^2-5^2=\left(x^2+7x+11-5\right)\left(x^2+7x+11+5\right)=\left(x^2+7x+6\right)\left(x^2+7x+16\right)=\left(x+1\right)\left(x+6\right)\left(x^2+7x+16\right)\)
a. \(x^2\left(x^2+4\right)-x^2-4\)
\(=x^2\left(x^2+4\right)-\left(x^2+4\right)\)
\(=\left(x^2-1\right)\left(x^2+4\right)\)
\(=\left(x-1\right)\left(x+1\right)\left(x^2+4\right)\)
b. \(\left(x^2+x\right)^2+4x^2+4x-12\)
\(=x^4+2x^3+5x^2+4x-12\)
\(=\left(x-1\right)\left(x+2\right)\left(x^2+x+6\right)\)
c. \(\left(x+2\right)\left(x+3\right)\left(x+4\right)\left(x+5\right)-24\)
\(=\left(x+2\right)\left(x+5\right)\left(x+3\right)\left(x+4\right)-24\)
\(=\left(x^2+7x+10\right)\left(x^2+7x+12\right)-24\) (*)
Đặt \(t=x^2+7x+10\), ta được
(*) \(=t\left(t+2\right)-24\)
\(=t^2+2t-24\)
\(=\left(t-4\right)\left(t+6\right)\)
hay \(\left(x^2+7x+6\right)\left(x^2+7x+18\right)\)
a: Ta có: \(x^2\left(x^2+4\right)-x^2-4\)
\(=\left(x^2+4\right)\left(x^2-1\right)\)
\(=\left(x^2+4\right)\left(x-1\right)\left(x+1\right)\)
b: Ta có: \(\left(x^2+x\right)^2+4x^2+4x-12\)
\(=\left(x^2+x\right)^2+4\left(x^2+x\right)-12\)
\(=\left(x^2+x\right)^2+6\left(x^2+x\right)-2\left(x^2+x\right)-12\)
\(=\left(x^2+x-2\right)\left(x^2+x+6\right)\)
\(=\left(x^2+x+6\right)\left(x+2\right)\left(x-1\right)\)
c: Ta có: \(\left(x+2\right)\left(x+3\right)\left(x+4\right)\left(x+5\right)-24\)
\(=\left(x^2+7x+10\right)\left(x^2+7x+12\right)-24\)
\(=\left(x^2+7x\right)^2+22\left(x^2+7x\right)+96\)
\(=\left(x^2+7x+6\right)\left(x^2+7x+16\right)\)
\(=\left(x^2+7x+16\right)\left(x+1\right)\left(x+6\right)\)
Bài 1: Cho phân thức: 3x2+6x+12x3−83x2+6x+12x3−8
a,Tìm điều kiện của x để phân thức đã cho được xác định
b, Rút gọn phân thức
c, Tính giá trị của phân thức sau khi rút gọn với x = 4001200040012000
Bài 2: Cho phân thức: x2−10x+25x2−5xx2−10x+25x2−5x
a, Tìm giá trị của x để phân thức bằng 0
b, Tìm x để giá trị của phân thức bằng 5252
c, Tìm x nguyên để phân thức có giá trị nguyên
Bài 3: Cho biểu thức: (x+12x−2+3x2−1−x+32x+2)(4x2−45)(x+12x−2+3x2−1−x+32x+2)(4x2−45)
a, Tìm điều kiện của x để giá trị của biểu thức được xác định
b, CMR: Khi giá trị của biểu thức được xác định thì nó không phụ thuộc vào giá trị của biến x
x2−10x+25x2−5x=(x−5)2x(x−5)=x−5x" role="presentation" style="border:0px; box-sizing:border-box; direction:ltr; display:inline; float:none; line-height:normal; margin:0px; max-height:none; max-width:none; min-height:0px; min-width:0px; overflow-wrap:normal; padding:0px; position:relative; white-space:nowrap; word-spacing:normal" class="MathJax">x2−10x+25x2−5x=(x−5)2x(x−5)=x−5x
x−5x" role="presentation" style="border:0px; box-sizing:border-box; direction:ltr; display:inline; float:none; line-height:normal; margin:0px; max-height:none; max-width:none; min-height:0px; min-width:0px; overflow-wrap:normal; padding:0px; position:relative; white-space:nowrap; word-spacing:normal" class="MathJax">x−5x phải có giá trị nguyên.
x+12x−2+3x2−1−x+32x+2)⋅(4x2−45)" role="presentation" style="border:0px; box-sizing:border-box; direction:ltr; display:inline; float:none; line-height:normal; margin:0px; max-height:none; max-width:none; min-height:0px; min-width:0px; overflow-wrap:normal; padding:0px; position:relative; white-space:nowrap; word-spacing:normal" class="MathJax">x+12x−2+3x2−1−x+32x+2)⋅(4x2−45)
x+12(x−1)+3(x−1)(x+1)−x+32(x+1))⋅2(2x2−2)5" role="presentation" style="border:0px; box-sizing:border-box; direction:ltr; display:inline; float:none; line-height:normal; margin:0px; max-height:none; max-width:none; min-height:0px; min-width:0px; overflow-wrap:normal; padding:0px; position:relative; white-space:nowrap; word-spacing:normal" class="MathJax">x+12(x−1)+3(x−1)(x+1)−x+32(x+1))⋅2(2x2−2)5
(x+1)2+6−(x−1)(x+3)2(x−1)(x+1)⋅2⋅2(x2−1)5" role="presentation" style="border:0px; box-sizing:border-box; direction:ltr; display:inline; float:none; line-height:normal; margin:0px; max-height:none; max-width:none; min-height:0px; min-width:0px; overflow-wrap:normal; padding:0px; position:relative; white-space:nowrap; word-spacing:normal" class="MathJax">(x+1)2+6−(x−1)(x+3)2(x−1)(x+1)⋅2⋅2(x2−1)5
(x+1)2+6−(x2+3x−x−3)(x−1)(x+1)⋅2(x−1)(x+1)5" role="presentation" style="border:0px; box-sizing:border-box; direction:ltr; display:inline; float:none; line-height:normal; margin:0px; max-height:none; max-width:none; min-height:0px; min-width:0px; overflow-wrap:normal; padding:0px; position:relative; white-space:nowrap; word-spacing:normal" class="MathJax">(x+1)2+6−(x2+3x−x−3)(x−1)(x+1)⋅2(x−1)(x+1)5
25" role="presentation" style="border:0px; box-sizing:border-box; direction:ltr; display:inline; float:none; line-height:normal; margin:0px; max-height:none; max-width:none; min-height:0px; min-width:0px; overflow-wrap:normal; padding:0px; position:relative; white-space:nowrap; word-spacing:normal" class="MathJax">25
25" role="presentation" style="border:0px; box-sizing:border-box; direction:ltr; display:inline; float:none; line-height:normal; margin:0px; max-height:none; max-width:none; min-height:0px; min-width:0px; overflow-wrap:normal; padding:0px; position:relative; white-space:nowrap; word-spacing:normal" class="MathJax">25
25" role="presentation" style="border:0px; box-sizing:border-box; direction:ltr; display:inline; float:none; line-height:normal; margin:0px; max-height:none; max-width:none; min-height:0px; min-width:0px; overflow-wrap:normal; padding:0px; position:relative; white-space:nowrap; word-spacing:normal" class="MathJax">25
2(x+1)25+185−25x2−45x" role="presentation" style="border:0px; box-sizing:border-box; direction:ltr; display:inline-table; float:none; line-height:normal; margin:0px; max-height:none; max-width:none; min-height:0px; min-width:0px; overflow-wrap:normal; padding:0px; position:relative; white-space:nowrap; word-spacing:normal" class="MathJax">2(x+1)25+185−25x2−45x
2(x2+2x+1)5+185−25x2−45x" role="presentation" style="border:0px; box-sizing:border-box; direction:ltr; display:inline; float:none; line-height:normal; margin:0px; max-height:none; max-width:none; min-height:0px; min-width:0px; overflow-wrap:normal; padding:0px; position:relative; white-space:nowrap; word-spacing:normal" class="MathJax">2(x2+2x+1)5+185−25x2−45x
2x2+4x+25+185−25x2−45x" role="presentation" style="border:0px; box-sizing:border-box; direction:ltr; display:inline; float:none; line-height:normal; margin:0px; max-height:none; max-width:none; min-height:0px; min-width:0px; overflow-wrap:normal; padding:0px; position:relative; white-space:nowrap; word-spacing:normal" class="MathJax">2x2+4x+25+185−25x2−45x
2x2+4x+2+185−25x2−45x" role="presentation" style="border:0px; box-sizing:border-box; direction:ltr; display:inline; float:none; line-height:normal; margin:0px; max-height:none; max-width:none; min-height:0px; min-width:0px; overflow-wrap:normal; padding:0px; position:relative; white-space:nowrap; word-spacing:normal" class="MathJax">2x2+4x+2+185−25x2−45x
2x2+4x+205−25x2−45x" role="presentation" style="border:0px; box-sizing:border-box; direction:ltr; display:inline; float:none; line-height:normal; margin:0px; max-height:none; max-width:none; min-height:0px; min-width:0px; overflow-wrap:normal; padding:0px; position:relative; white-space:nowrap; word-spacing:normal" class="MathJax">2x2+4x+205−25x2−45x
c) tự làm, đkxđ: x≠1;x≠−1
A) X3-3x2+3x-1+2(x2-x)
B)12x3+4x2-27x-9
C)X4-25X2+20x-4
tìm a và b biết \(\dfrac{a}{2}=\dfrac{b}{3}\) và a.b = 24
giúp mk với
Đặt \(\dfrac{a}{2}=\dfrac{b}{3}=k\Leftrightarrow a=2k;b=3k\)
\(ab=24\Leftrightarrow6k^2=24\Leftrightarrow k^2=2\\ \Leftrightarrow\left[{}\begin{matrix}k=2\\k=-2\end{matrix}\right.\Leftrightarrow\left[{}\begin{matrix}a=4;b=6\\a=-4;b=-6\end{matrix}\right.\)
Ta có :
\(\dfrac{a}{2}=\dfrac{b}{3}=k\)
\(\Rightarrow\left\{{}\begin{matrix}a=2k\\b=3k\end{matrix}\right.\)
mà \(ab=24\)
\(\Rightarrow2k.3k=24\)
\(\Rightarrow6k^2=24\)
\(\Rightarrow k^2=2^2\)
\(\Rightarrow k=\left\{{}\begin{matrix}2\\-2\end{matrix}\right.\)
\(\Rightarrow\left\{{}\begin{matrix}\dfrac{a}{2}=\dfrac{b}{3}=2\\\dfrac{a}{2}=\dfrac{b}{3}=-2\end{matrix}\right.\)
\(\Rightarrow\left\{{}\begin{matrix}a=4;b=6\\a=-4;b=-6\end{matrix}\right.\)
Ta có:
(a.b)/(2.3)= 24/6=4(T/c dãy tỉ số = nhau )
=>a=4.2=8
b=4.3=12
tìm x y nguyên biết
3xy - 2x - 2y = 24
giúp
Lời giải:
$3xy-2x-2y=24$
$\Rightarrow (3xy-2x)-2y=24$
$\Rightarrow x(3y-2)-2y=24$
$\Rightarrow 3x(3y-2)-6y=72$
$\Rightarrow 3x(3y-2)-2(3y-2)=76$
$\Rightarrow (3x-2)(3y-2)=76$
Vì $x,y$ nguyên nên $3x-2, 3y-2$ cũng là số nguyên. Do đo $3x-2, 3y-2$ là ước của 76.
Đến đây thì đơn giản rồi. Bạn chỉ cần xét các TH khác nhau của ước của 76.