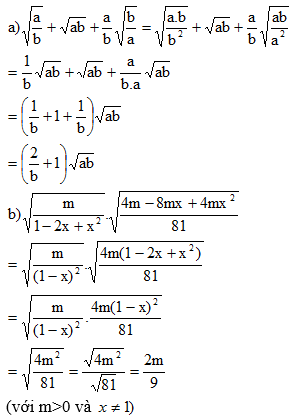Rút gọn biểu thức \(M=\sqrt{\frac{m}{1-2x+x^2}}.\sqrt{\frac{4m-8mx+4mx^2}{81}}\)
Tuyển Cộng tác viên Hoc24 nhiệm kì 26 tại đây: https://forms.gle/dK3zGK3LHFrgvTkJ6
SA
Những câu hỏi liên quan
\(\sqrt{\frac{m}{1-2x+x^2}}.\sqrt{\frac{4m-8mx+4mx^2}{81}}\) voi \(m>0;x\ne1\)
\(\sqrt{\frac{m}{1-2x+x^2}}.\sqrt{\frac{4m-8mx+4mx^2}{81}}=\sqrt{\frac{m}{\left(x-1\right)^2}}.\sqrt{\frac{4m\left(1-2x+x^2\right)}{81}}\)
\(=\sqrt{\frac{m}{\left(x-1\right)^2}}.\sqrt{\frac{4m\left(x-1\right)^2}{81}}=\frac{\sqrt{m}}{\left|x-1\right|}.\frac{2\sqrt{m}.\left|x-1\right|}{9}=\frac{2m}{9}\)
\(x\ne1\) chứ không phải x>1 nên không thể ghi |x-1|=x-1 nhé Despacito
Đúng 0
Bình luận (0)
A..mk vua nghi ra bai nay
\(\sqrt{\frac{m}{x^2-2x+1}}.\sqrt{\frac{4m-8mx+4mx^2}{81}}\)
\(=\sqrt{\frac{m}{\left(x-1\right)^2}}.\sqrt{\frac{4m\left(1-2x+x^2\right)}{81}}\)
\(=\frac{\sqrt{m}}{\left|x-1\right|}.\frac{\sqrt{4m\left(x-1\right)^2}}{9}\) ( Thoa man DKXD \(m>0;x\ne1\)
\(=\frac{\sqrt{m}}{x-1}.\frac{2\left(x-1\right).\sqrt{m}}{9}\)
\(=\frac{2m}{9}\)
ko biet co dung ko nua
Đúng 0
Bình luận (0)
Mình làm cách khác mà cũng ra giống kq zậy đó
Đúng 0
Bình luận (0)
Xem thêm câu trả lời
\(\sqrt{\dfrac{m}{1-2x+x^2}}+\sqrt{\dfrac{4m-8mx+4mx^2}{81}}\left(x>1,m\ge0\right)\)
\(\sqrt{\dfrac{m}{1-2x+x^2}}+\sqrt{\dfrac{4m-8mx+4mx^2}{81}}=\sqrt{\dfrac{m}{\left(1-x\right)^2}}+\sqrt{\dfrac{4m\left(1-2x+x^2\right)}{81}}\\ =\dfrac{\sqrt{m}}{\left|1-x\right|}+\dfrac{2\sqrt{m}\left|1-x\right|}{9}=\dfrac{9\sqrt{m}+2\sqrt{m}\left(1-x\right)^2}{\left|1-x\right|.9}\\ =\dfrac{\sqrt{m}\left(9+2-4x+2x^2\right)}{\left(x-1\right).9}\)
tới đây thì hết bt rồi
Đúng 0
Bình luận (0)
Toán 8 nâng cao
1/ \(\sqrt{\frac{m}{1-2x+x^2}}\cdot\sqrt{\frac{4m-8mx+4mx^2}{81}}\)
2/\(\left(\frac{1-a\sqrt{a}}{1-\sqrt{a}}+\sqrt{a}\right)\left(\frac{1-\sqrt{a}}{1-a}\right)^2\)
3/\(\frac{a+b}{b^2}\sqrt{\frac{a^2b^4}{a^2+2ab+b^2}}\)
1/ \(\sqrt{\frac{m}{1-2x+x^2}}\cdot\sqrt{\frac{4m-8mx+4mx^2}{81}}\)
\(=\sqrt{\frac{m}{\left(1-x\right)^2}}\cdot\sqrt{\frac{4m\left(1-2x+x^2\right)}{81}}\)
\(=\sqrt{\frac{m}{\left(1-x\right)^2}}\cdot\sqrt{\frac{4m\left(1-x\right)^2}{81}}\)
\(=\sqrt{\frac{m}{\left(1-x\right)^2}\cdot\frac{4m\left(1-x\right)^2}{81}}\)
\(=\sqrt{\frac{4m^2}{81}}=\sqrt{\frac{\left(2m\right)^2}{9^2}}=\frac{2\left|m\right|}{9}\)
3/\(\frac{a+b}{b^2}\sqrt{\frac{a^2b^4}{a^2+2ab+b^2}}\)
\(=\frac{a+b}{b^2}\sqrt{\frac{\left(ab^2\right)^2}{\left(a+b\right)^2}}\)
\(=\frac{a+b}{b^2}\cdot\frac{\left|a\right|b^2}{\left|a+b\right|}\)
TH1: \(\Rightarrow\frac{a+b}{b^2}\cdot\frac{\left|a\right|b^2}{-\left(a+b\right)}=-\left|a\right|\)
TH2: \(\Rightarrow\frac{a+b}{b^2}\cdot\frac{\left|a\right|b^2}{a+b}=\left|a\right|\)
Đúng 0
Bình luận (0)
2/\(\left(\frac{1-a\sqrt{a}}{1-\sqrt{a}}+\sqrt{a}\right)\left(\frac{1-\sqrt{a}}{1-a}\right)^2\)
\(=\left(\frac{1-a\sqrt{a}}{1-\sqrt{a}}+\sqrt{a}\right)\cdot\frac{\left(1-\sqrt{a}\right)^2}{\left(1-a\right)^2}\)
\(=\left(\frac{1-a\sqrt{a}}{1-\sqrt{a}}+\frac{\sqrt{a}\left(1-\sqrt{a}\right)}{1-\sqrt{a}}\right)\cdot\frac{\left(1-\sqrt{a}\right)^2}{\left(1-a\right)^2}\)
\(=\left(\frac{1-a\sqrt{a}}{1-\sqrt{a}}+\frac{\sqrt{a}-a}{1-\sqrt{a}}\right)\cdot\frac{\left(1-\sqrt{a}\right)^2}{\left(1-a\right)^2}\)
\(=\frac{1-a\sqrt{a}+\sqrt{a}-a}{1-\sqrt{a}}\cdot\frac{\left(1-\sqrt{a}\right)^2}{\left(1-a\right)^2}\)
\(=\frac{1-a\sqrt{a}+\sqrt{a}-a}{1}\cdot\frac{1-\sqrt{a}}{\left(1-a\right)^2}\)
\(=\frac{\left(1-a\sqrt{a}+\sqrt{a}-a\right)\cdot\left(1-\sqrt{a}\right)}{\left(1-a\right)^2}\)
\(=\frac{1-a\sqrt{a}+\sqrt{a}-a-\sqrt{a}+a^2-a+a\sqrt{a}}{\left(1-a\right)^2}\)
\(=\frac{a^2-2a+1}{\left(1-a\right)^2}\)
\(=\frac{\left(a-1\right)^2}{\left(1-a\right)^2}=\frac{-\left(1-a\right)^2}{\left(1-a\right)^2}=-1\)
Đúng 0
Bình luận (0)
1. Cho biểu thức:
B= ( \(\frac{\sqrt{x}}{\sqrt{x}-1}+\frac{2}{x-\sqrt{x}}\)) :\(\frac{1}{\sqrt{x}-1}\)
a) Rút gọn B
b) Tìm Min B
2. Rút gọn biểu thức:
\(\sqrt{\frac{1}{1-2x+x^2}}.\sqrt{\frac{4-4x+4x^2}{81}}\)
3. giải phương trình: 3+\(\sqrt{2x-3}\)= x
Rút gọn biểu thức: P=\(\frac{2\sqrt{x}}{\sqrt{x}+m}\)+\(\frac{\sqrt{x}}{\sqrt{x}-m}\)-\(\frac{m^2}{4x-4m^2}\)
Help me Cần gấp
P=\(\frac{2\sqrt{x}}{\sqrt{x}+m}+\frac{2\sqrt{x}}{\sqrt{x}-m}-\)\(\frac{m^2}{4x-4m^2}\)
= \(\frac{8\sqrt{x}\left(\sqrt{x}-m\right)+8\sqrt{x}\left(\sqrt{x}+m\right)-m^2}{4x-4m^2}\)
=\(\frac{8\sqrt{x}\left(\sqrt{x}-m+\sqrt{x}+m\right)-m^2}{4x-4m^2}\)
=\(\frac{8\sqrt{x}.2\sqrt{x}-m^2}{4x-4m^2}\)
=\(\frac{16x-m^2}{4x-4m^2}\)
Đúng 0
Bình luận (0)
Rút gọn biểu thức: \(M=\left(\frac{1}{2}-\frac{1}{2x}\right)\left(\frac{x-\sqrt{x}}{\sqrt{x}+1}-\frac{x+\sqrt{x}}{\sqrt{x}-1}\right)\)
ĐK:x>1
M=\(\frac{x-1}{2x}\) .\(\frac{\left(x-\sqrt{x}\right)\left(\sqrt{x}-1\right)-\left(x+\sqrt{x}\right)\left(\sqrt{x}+1\right)}{x-1}\)
=\(\frac{x-1}{2x}\).\(\frac{x\sqrt{x}-x-x+\sqrt{x}-x\sqrt{x}-x-x-\sqrt{x}}{x-1}\)=\(\frac{x-1}{2x}\).\(\frac{-4x}{x-1}\)=-2
Vậy M=-2
Đúng 0
Bình luận (0)
a) \(\sqrt{\dfrac{a}{b}}+\sqrt{ab}+\dfrac{a}{b}\sqrt{\dfrac{a}{b}}\) với \(a>0;b>0\)
b) \(\sqrt{\dfrac{m}{1-2x+x^2}}.\sqrt{\dfrac{4m-8mx+4mx^2}{81}}\) vớ \(m>0;x\ne1\)
a) \(\sqrt{\dfrac{a}{b}}+\sqrt{ab}+\dfrac{a}{b}\sqrt{\dfrac{a}{b}}\) với a>0 và b>0
b) \(\sqrt{\dfrac{m}{1-2x+x^2}}.\sqrt{\dfrac{4m-8mx+4mx^2}{81}}=\sqrt{\dfrac{m}{1-2x+x^2}}.\sqrt{\dfrac{4m\left(2-2x+x^2\right)}{81}}\)
\(=\sqrt{\dfrac{4m^2\left(1-2x+x^2\right)}{81\left(1-2x+x^2\right)}}=\sqrt{\dfrac{4m^2}{81}}=\sqrt{\dfrac{2m}{9}}\)
Đúng 0
Bình luận (0)
a)Rút gọn biểu thức
M=\(\frac{x^2+\sqrt{x}}{x-\sqrt{x}+1}+1-\frac{2x+\sqrt{x}}{\sqrt{x}}\) với x>0
b)Tìm GTNN của M
a) \(M=\frac{x^2+\sqrt{x}}{x-\sqrt{x}+1}+1\)\(-\frac{2x+\sqrt{x}}{\sqrt{x}}\)
\(=\frac{\sqrt{x}\left(\sqrt{x^3}+1\right)}{x-\sqrt{x}+1}\)\(+\frac{\sqrt{x}-2x-\sqrt{x}}{\sqrt{x}}\)
\(=\sqrt{x}\left(\sqrt{x}+1\right)-2\sqrt{x}\)
\(=x+\sqrt{x}-2\sqrt{x}=x-\sqrt{x}\)
Đúng 0
Bình luận (0)
Cho biểu thức : \(M=\frac{x+\sqrt{x^2-2x}}{x-\sqrt{x^2-2x}}-\frac{x-\sqrt{x^2-2x}}{x+\sqrt{x^2-2x}}\) \(\left(x< 0;x\ge2\right)\)
a, Rút gọn biểu thức M
b, Tìm giá trị của x để M < 2.
\(M=\frac{x+\sqrt{x^2-2x}}{x-\sqrt{x^2-2x}}-\frac{x-\sqrt{x^2-2x}}{x+\sqrt{x^2-2x}}\left(x< 0;x\ge2\right)\)
\(=\frac{\left(x+\sqrt{x^2-2x}\right)\left(x+\sqrt{x^2-2x}\right)}{x^2-\sqrt{x^2-2x}^2}-\frac{\left(x-\sqrt{x^2-2x}\right)\left(x-\sqrt{x^2-2x}\right)}{x^2-\sqrt{x^2-2x}^2}\)
\(=\frac{x^2+x\sqrt{x^2-2x}+x\sqrt{x^2-2x}+x^2-2x}{x^2-x^2-2x}-\frac{x^2-x\sqrt{x^2-2x}-x\sqrt{x^2-2x}+x^2-2x}{x^2-x^2-2x}\)
\(=\frac{2x^2+2x\sqrt{x^2-2x}-2x}{-2x}-\frac{2x^2-2\sqrt{x^2-2x}-2x}{-2x}\)
\(=\frac{2x^2+2x\sqrt{x^2-2x}-2x-2x^2+2x\sqrt{x^2-2x}+2x}{-2x}\)
\(=\frac{4x\sqrt{x^2-2x}}{-2x}=-2x\sqrt{x^2-2x}\)
Đúng 0
Bình luận (0)