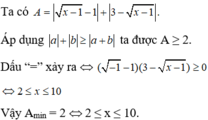\(A=\left(x^2-2x+1\right)+4=\left(x-1\right)^2+4\ge4\\ A_{min}=4\Leftrightarrow x=1\\ B=2\left(x^2-3x\right)=2\left(x^2-2\cdot\dfrac{3}{2}x+\dfrac{9}{4}\right)-\dfrac{9}{2}\\ B=2\left(x-\dfrac{3}{2}\right)^2-\dfrac{9}{2}\ge-\dfrac{9}{2}\\ B_{min}=-\dfrac{9}{2}\Leftrightarrow x=\dfrac{3}{2}\\ C=-\left(x^2-4x+4\right)+7=-\left(x-2\right)^2+7\le7\\ C_{max}=7\Leftrightarrow x=2\)

a,\(A=x^2-2x+5=\left(x^2-2x+1\right)+4=\left(x-1\right)^2+4\ge4\)

Dấu "=" \(\Leftrightarrow x=-1\)

b,\(B=2\left(x^2-3x\right)=2\left(x^2-3x+\dfrac{9}{4}\right)-\dfrac{9}{2}=2\left(x-\dfrac{3}{2}\right)^2-\dfrac{9}{2}\ge-\dfrac{9}{2}\)

Dấu "=" \(\Leftrightarrow x=\dfrac{3}{2}\)

c,\(=C=-\left(x^2-4x-3\right)=-\left[\left(x^2-4x+4\right)-7\right]=-\left(x-2\right)^2+7\le7\)

Dấu "=" \(\Leftrightarrow x=2\)

um

A = 2x² + 6x - 1

A = 2( x² + 3x - 1 / 2 )

A = 2[ x² + 2 . 3 / 2 . x + ( 3 / 2 )² - ( 3 / 2 )² - 1 / 2 ]

A = 2[ ( x + 3 / 2 )² - 11 / 4 ]

A = ( x + 3 / 2 )² - 11 / 2 \(\ge\)- 11 / 2

Dấu " = " xảy ra\(\Leftrightarrow\)x + 3 / 2 = 0

                            \(\Rightarrow\)x              = 3 / 2

Min A = - 11 / 2 \(\Leftrightarrow\)x = 3 / 2

1) \(A=-\left(x^2-6x-1\right)=-\left(x^2-2.3x+9-10\right)\)

         \(=-\left(x-3\right)^2+10\)

         \(=10-\left(x-3\right)^2\le10\)  ( vì  \(\left(x-3\right)^2\ge0\) với mọi x)

Dấu "=" xảy ra \(\Leftrightarrow x=3\)

Vậy Max A = 10 tại x=3.

\(A=\frac{2x^2-6x+5}{x^2-2x+1}=\frac{x^2-4x+4+x^2-2x+1}{x^2-2x+1}\)

\(=\frac{\left(x-2\right)^2+\left(x-1\right)^2}{\left(x-1\right)^2}=\frac{\left(x-2\right)^2}{\left(x-1\right)^2}+1\)

Vì \(\hept{\begin{cases}\left(x-2\right)^2\ge0\\\left(x-1\right)^2\ge0\end{cases}}\)\(\Rightarrow\frac{\left(x-2\right)^2}{\left(x-1\right)^2}\ge0\)\(\Rightarrow\frac{\left(x-2\right)^2}{\left(x-1\right)^2}+1\ge1\)

\(\Rightarrow A\ge1\).Nên GTNN của \(A=1\) đạt được khi \(x=2\)

dòng thứ 2 ko hiểu

1) \(M=9x^2-6x+6=\left(9x^2-6x+1\right)+5=\left(3x-1\right)^2+5\ge5\)

\(minM=5\Leftrightarrow x=\dfrac{1}{3}\)

2) \(M=5-2x-x^2=-\left(x^2+2x+1\right)+6=-\left(x+1\right)^2+6\le6\)

\(maxM=6\Leftrightarrow x=-1\)

3) \(N=5+6x-9x^2=-\left(9x^2-6x+1\right)+6=-\left(3x-1\right)^2+6\le6\)

\(maxN=6\Leftrightarrow x=\dfrac{1}{3}\)

lập bảng xét dấu đi