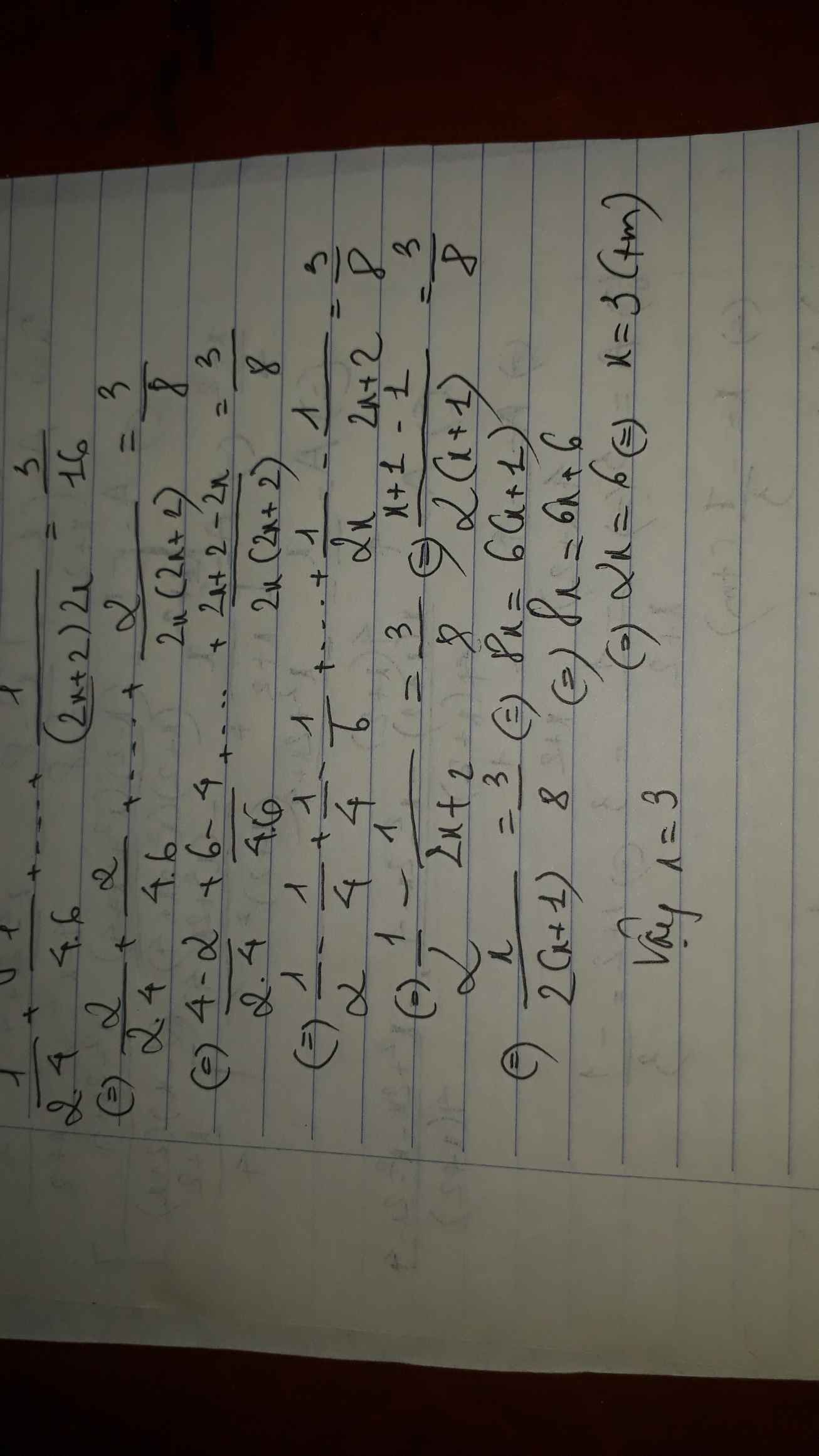1/(2.4) + 1/(4.6) + … + 1/[(2x – 2).2x] = 1/8 (x ∈ N, x ≥ 2)
Tuyển Cộng tác viên Hoc24 nhiệm kì 26 tại đây: https://forms.gle/dK3zGK3LHFrgvTkJ6
H24
Những câu hỏi liên quan
1/(2.4) + 1/(4.6) + … + 1/[(2x – 2).2x] = 1/8 (x ∈ n, x ≥ 2)
2 . 1/8 = 2/2.4 + 2/4.6 + ...+ 2/((2x -2).2x)
1/4 = 4-2/2.4 + 6-4/4.6 + ... + 2x-(2x-2)/(2x-2)+2x
1/4 = 1/2 - 1/4 + 1/4 - 1/6 + 1/6 - 1/8 + ... + 1/2x -2 - 1/2x
1/4 = 1/2 - 1/2x
1/4 = 2x-2/2.2x
Tự làm tiếp nhé
Mong Bạn Bấm Cho mik
Tìm x, biết
1/(2.4) + 1/(4.6) + … + 1/[(2x – 2).2x] = 1/8 (x ∈ N, x ≥ 2)
\(\frac{1}{2\cdot4}+\frac{1}{4\cdot6}+...+\frac{1}{\left(2x-2\right)\cdot2x}=\frac{1}{8}\)
\(\Rightarrow\frac{2}{2\cdot4}+\frac{2}{4\cdot6}+...+\frac{2}{\left(2x-2\right)\cdot2x}=\frac{2}{8}\)
\(\Rightarrow\frac{1}{2}-\frac{1}{4}+\frac{1}{4}-\frac{1}{6}+...+\frac{1}{2x-2}-\frac{1}{2x}=\frac{1}{4}\)
\(\Rightarrow\frac{1}{2}-\frac{1}{2x}=\frac{1}{4}\)
\(\Rightarrow\frac{1}{2x}=\frac{1}{2}-\frac{1}{4}\)
\(\Rightarrow\frac{1}{2x}=\frac{1}{4}\)
\(\Rightarrow2x=4\)
\(\Rightarrow x=2\)
Đúng 0
Bình luận (0)
tìm x
1/2.4+1/4.6+.......+1/(2x-2).2x =1/8(x thuộc N ,x> bằng 2)
\(\frac{1}{2.4}+\frac{1}{4.6}+...+\frac{1}{\left(2x-2\right).2x}\)= \(\frac{1}{8}\)
\(\frac{1}{2}.\left(\frac{2}{2.4}+\frac{2}{4.6}+...+\frac{2}{\left(2x-2\right).2x}\right)\)= \(\frac{1}{8}\)
\(\frac{1}{2}.\left(\frac{1}{2}-\frac{1}{2x}\right)\)= \(\frac{1}{8}\)
=> \(\frac{1}{2}-\frac{1}{2x}\)= \(\frac{1}{4}\)
=> 1/2x = 1/4
=> 2x = 4
x = 4 : 2
x = 2
Đúng 0
Bình luận (0)
\(\frac{1}{2.4}+\frac{1}{4.6}+...+\frac{1}{\left(2x-2\right)2x}=\frac{1}{8}\)
\(\Rightarrow\frac{1}{2}\left(\frac{1}{2}-\frac{1}{4}+\frac{1}{4}-\frac{1}{6}+...+\frac{1}{2x-2}-\frac{1}{2x}\right)=\frac{1}{8}\)
\(\Rightarrow\frac{1}{2}-\frac{1}{2x}=\frac{1}{8}.2=\frac{1}{4}\)
\(\Rightarrow\frac{1}{2x}=\frac{1}{2}-\frac{1}{4}=\frac{1}{4}\)
\(\Rightarrow2x=4\Leftrightarrow x=2\)
Đúng 0
Bình luận (0)
Tìm x
\(\dfrac{1}{2.4}\) + \(\dfrac{1}{4.6}\) +...+ \(\dfrac{1}{\left(2x-2\right).2x}\) = \(\dfrac{1}{8}\) ( x ∈ N , x ≥ 2 )
có lời giải chi tiết
\(\dfrac{1}{2.4}+\dfrac{1}{4.6}+...+\dfrac{1}{\left(2x-2\right).2x}=\dfrac{1}{8}\)
\(\Leftrightarrow\dfrac{2}{2.4}+\dfrac{2}{4.6}+...+\dfrac{2}{\left(2x-2\right).2x}=\dfrac{1}{4}\)
\(\Leftrightarrow\dfrac{1}{2}-\dfrac{1}{4}+\dfrac{1}{4}-\dfrac{1}{4}+...+\dfrac{1}{2x-2}-\dfrac{1}{2x}=\dfrac{1}{4}\)
\(\Leftrightarrow\dfrac{1}{2}-\dfrac{1}{2x}=\dfrac{1}{4}\)
\(\Leftrightarrow\dfrac{1}{2x}=\dfrac{1}{4}\)
\(\Leftrightarrow2x=4\\ \Leftrightarrow x=2\left(tm\right)\)
Đúng 3
Bình luận (1)
\(\Leftrightarrow\dfrac{1}{4}\left(\dfrac{1}{1.2}+\dfrac{1}{2.3}+.......+\dfrac{1}{\left(x-1\right)x}\right)=\dfrac{1}{8}\) ( đk x khác 0 , x khác 1)
\(\Leftrightarrow\dfrac{1}{4}\left(1-\dfrac{1}{2}+\dfrac{1}{2}-\dfrac{1}{3}+....+\dfrac{1}{x-1}-\dfrac{1}{x}\right)=\dfrac{1}{8}\)
\(\Leftrightarrow1-\dfrac{1}{x}=\dfrac{1}{2}\)
=> x =2 ( tm)
Đúng 1
Bình luận (0)
ai giúp mik vs.....
Tìm x, biết
1/(2.4) + 1/(4.6) + … + 1/[(2x – 2).2x] = 1/8 (x ∈ N, x ≥ 2)
1/(2.4) + 1/(4.6) + … + 1/[(2x – 2).2x] = 1/8
suy ra 2/(2.4) + 2/(4.6) + ...+ 2/[(2x - 2).2x] = 2/8
suy ra 1-1/4+1/4-1/6+...+1/(2x-2) - 1/2x = 2/8
suy ra 1 - 1/2x = 2/8
suy ra 1/2x = 1 - 2/8
suy ra 1/2x = 6/8 = 3/4
suy ra 1.4 = 2.x.3
suy ra 4 = 6x
suy ra x thuộc rỗng
Vậy x thuộc rỗng
k cho mình nha. Chúc bạn học tốt!
Đúng 0
Bình luận (0)
Thùy Anh tính sai rùi, dòng 2 phải nhân cả tử và mẫu cùng zới 2 mò, ko nhận ra ak mng ưi
Đúng 0
Bình luận (0)
Xem thêm câu trả lời
1/(2.4) + 1/(4.6) + … + 1/[(2x – 2).2x] = 1/8 (x ∈ N, x ≥ 2)
giup vs
\(A=\dfrac{1}{2.4}+\dfrac{1}{4.6}+...+\dfrac{1}{\left(2x-2\right).2x}=\dfrac{1}{8}\)
\(2A=\dfrac{2}{2.4}+\dfrac{2}{4.6}+...+\dfrac{2}{\left(2x-2\right).2x}=\dfrac{1}{8}.2=\dfrac{2}{8}=\dfrac{1}{4}\)
\(2A=\dfrac{1}{2}-\dfrac{1}{4}+\dfrac{1}{4}-\dfrac{1}{6}+...+\dfrac{1}{2x-2}-\dfrac{1}{2x}=\dfrac{1}{4}\)
\(2A=\dfrac{1}{2}-\dfrac{1}{2x}=\dfrac{1}{4}\)
\(A=\dfrac{1}{2}.\left(\dfrac{1}{2}-\dfrac{1}{2x}\right)=\dfrac{1}{8}\)
\(A=\dfrac{1}{2}.\dfrac{1}{2}-\dfrac{1}{2}.\dfrac{1}{2x}=\dfrac{1}{8}\)
\(A=\dfrac{1}{4}-\dfrac{1}{4x}=\dfrac{1}{8}\)
\(\Rightarrow\dfrac{1}{4x}=\dfrac{1}{4}-\dfrac{1}{8}=\dfrac{2}{8}-\dfrac{1}{8}=\dfrac{1}{8}\)
\(\Rightarrow4x=8\)
\(\Rightarrow x=8:4=2\)
Đúng 0
Bình luận (5)
1/(2.4) 1/(4.6) … 1/[(2x – 2).2x] = 1/8 (x ∈ N, x ≥ 2)
Tìm x, biết:
\(\dfrac{1}{2.4}+\dfrac{1}{4.6}+...+\dfrac{1}{\left(2x-2\right).2x}=\dfrac{11}{48}\) (x ϵ N , x ≥ 2)
\(\Leftrightarrow\dfrac{2}{2.4}+\dfrac{2}{4.6}+...+\dfrac{2}{\left(2x-2\right).2x}=\dfrac{11}{24}\)
\(\Leftrightarrow\dfrac{4-2}{2.4}+\dfrac{6-4}{4.6}+...+\dfrac{2x-\left(2x-2\right)}{\left(2x-2\right).2x}=\dfrac{11}{24}\)
\(\Leftrightarrow\dfrac{1}{2}-\dfrac{1}{4}+\dfrac{1}{4}-\dfrac{1}{6}+...+\dfrac{1}{2x-2}-\dfrac{1}{2x}=\dfrac{11}{24}\)
\(\Leftrightarrow\dfrac{1}{2}-\dfrac{1}{2x}=\dfrac{11}{24}\)
\(\Leftrightarrow\dfrac{1}{2x}=\dfrac{1}{2}-\dfrac{11}{24}\)
\(\Leftrightarrow\dfrac{1}{2x}=\dfrac{1}{24}\)
\(\Rightarrow2x=24\)
\(\Rightarrow x=12\)
Đúng 2
Bình luận (0)
1/2.4+1/4.6+...+1/(2x.2).2x=3/16(x thuộc n, x lớn hơn hoặc bằng 2) giải hộ mình nhó