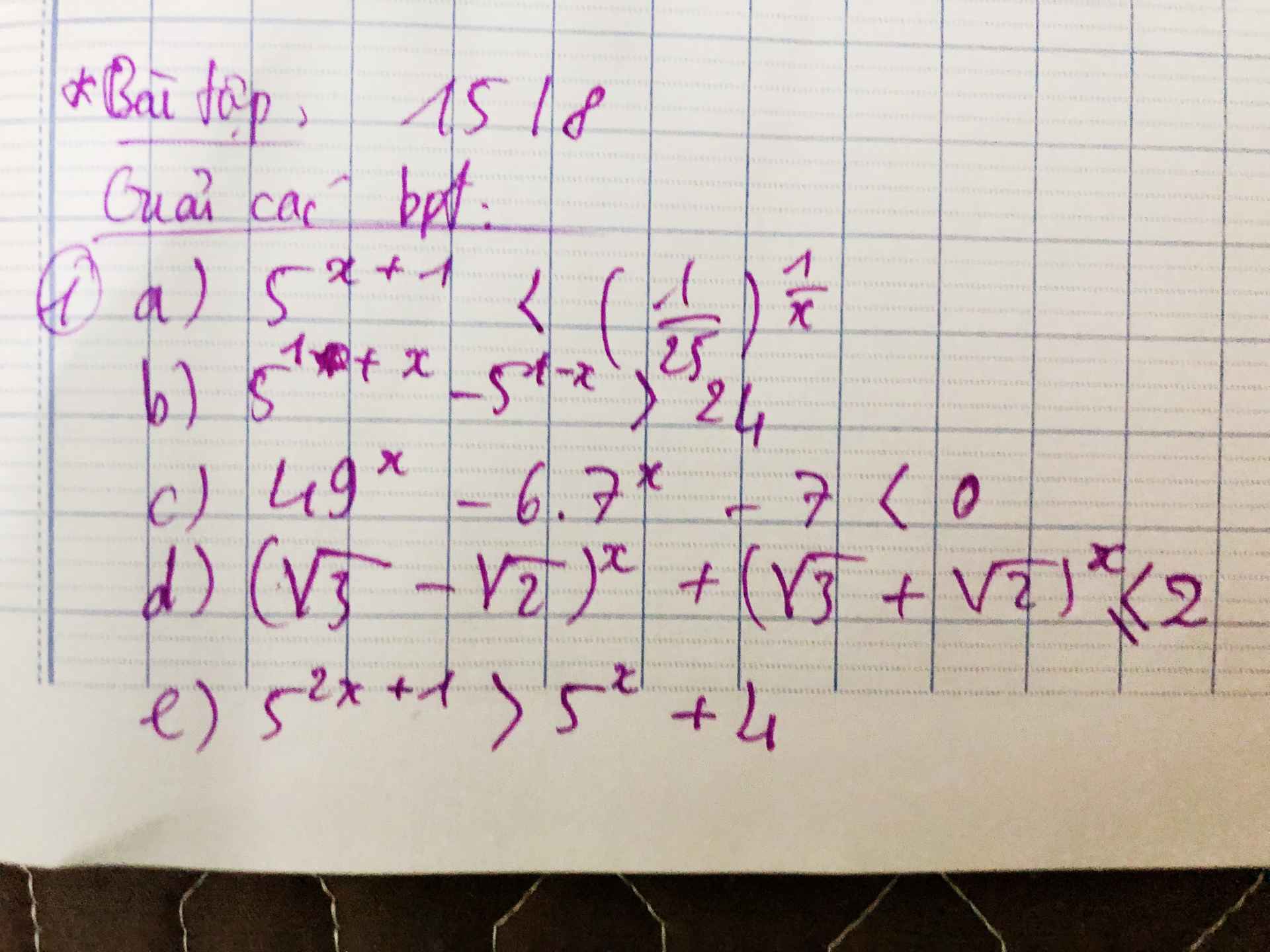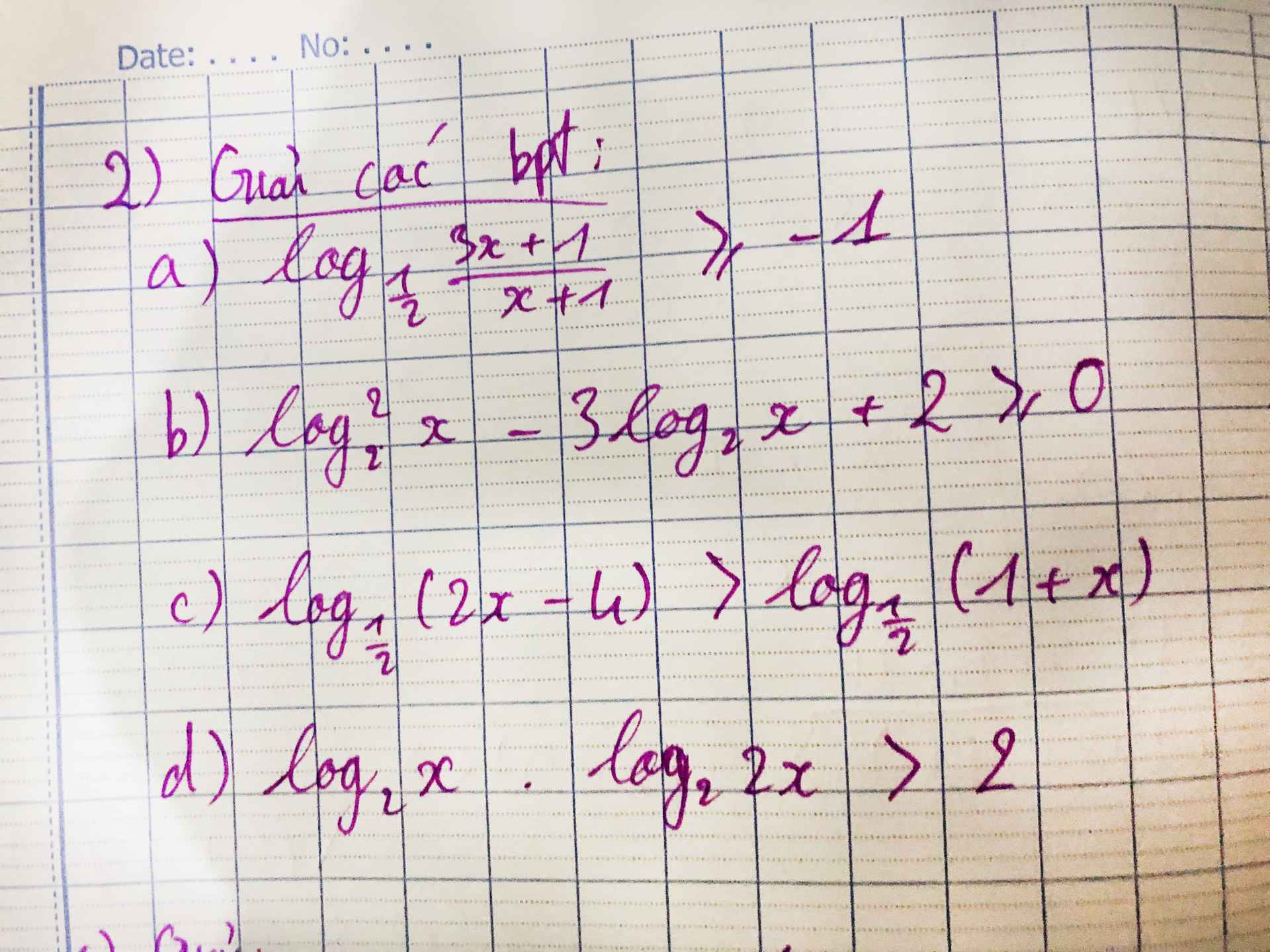Bài 6: Bất phương trình mũ và logarit

ĐKXĐ: \(x>0\)

\(log_3x< 2\)

\(\Leftrightarrow log_3x< log_39\)

\(\Rightarrow x< 9\)

Kết hợp ĐKXĐ ta được: \(0< x< 9\)

ĐKXĐ: \(x>0\)

\(\left(log_3x^5\right)^2-25.2.log_3x-750\le0\)

\(\Leftrightarrow\left(5log_3x\right)^2-50log_3x-750\le0\)

\(\Leftrightarrow25log_3^2x-50log_3x-750\le0\)

Đặt \(log_3x=t\)

\(\Rightarrow25t^2-50t-750\le0\)

\(\Rightarrow1-\sqrt{31}\le t\le1+\sqrt{31}\)

\(\Rightarrow1-\sqrt{31}\le log_3x\le1+\sqrt{31}\)

\(\Rightarrow3^{1-\sqrt{31}}\le x\le3^{1+\sqrt{31}}\)

1. ĐKXĐ: \(x>\dfrac{4}{3}\)

\(log_2\left(3x-4\right)< 2\)

\(\Leftrightarrow log_2\left(3x-4\right)< log_24\)

\(\Leftrightarrow3x-4< 4\)

\(\Rightarrow x< \dfrac{8}{3}\)

\(\Rightarrow\dfrac{4}{3}< x< \dfrac{8}{3}\)

b.

ĐKXĐ: \(x>\dfrac{1}{2}\)

\(log_{0,3}\left(x+1\right)>log_{0,3}\left(4x-2\right)\)

\(\Leftrightarrow x+1< 4x-2\) (do \(0,3< 1\) )

\(\Leftrightarrow x>1\)

Vậy \(x>1\)

3.

ĐKXĐ: \(\left[{}\begin{matrix}-3< x< 1\\x>3\end{matrix}\right.\)

\(log\left(x^2-4x+3\right)>log\left(x+3\right)\)

\(\Leftrightarrow x^2-4x+3>x+3\)

\(\Leftrightarrow x^2-5x>0\)

\(\Rightarrow\left[{}\begin{matrix}x>5\\x< 0\end{matrix}\right.\)

Kết hợp ĐKXĐ: \(\left[{}\begin{matrix}-3< x< 0\\x>5\end{matrix}\right.\)

4.

ĐKXĐ: \(\dfrac{3}{2}< x< 2\)

\(ln\left(4-x^2\right)\le ln\left(2x-3\right)\)

\(\Leftrightarrow4-x^2\le2x-3\)

\(\Leftrightarrow x^2+2x-7\ge0\Rightarrow\left[{}\begin{matrix}x\ge-1+2\sqrt{2}\\x\le-1-2\sqrt{2}\end{matrix}\right.\)

Kết hợp ĐKXĐ: \(\Rightarrow-1+2\sqrt{2}\le x< 2\)

Lời giải:

$\ln (x+2)< 0$

$\Leftrightarrow 0< x+2< 1$

$\Leftrightarrow -2< x< -1$

Ta có:\(5^{x-1}\ge5^{x^2-x-9}\)

   \(\Leftrightarrow x-1\ge x^2-x-9\)

   \(\Leftrightarrow x^2-2x-8\le0\)

   \(\Leftrightarrow\left(x-4\right)\left(x+2\right)\le0\)

   \(\Leftrightarrow\left[{}\begin{matrix}\left\{{}\begin{matrix}x-4\le0\\x+2\ge0\end{matrix}\right.\\\left\{{}\begin{matrix}x-4\ge0\\x+2\le0\end{matrix}\right.\end{matrix}\right.\Leftrightarrow\left[{}\begin{matrix}\left\{{}\begin{matrix}x\le4\\x\ge-2\end{matrix}\right.\\\left\{{}\begin{matrix}x\ge4\\x\le-2\end{matrix}\right.\end{matrix}\right.\Leftrightarrow-2\le x\le4\)

ĐKXĐ: \(x>-3\)

Xét hàm \(f\left(x\right)=2^{x+1}+log_3\left(x+3\right)-12\)

\(f'\left(x\right)=2^{x+1}.ln2+\dfrac{1}{\left(x+3\right)ln3}>0\) ; \(\forall x>-3\)

\(\Rightarrow f\left(x\right)\) đồng biến

\(f\left(2\right)=8+log_35-12=log_35-4< 0\)

\(f\left(3\right)=4+log_36>0\)

\(\Rightarrow f\left(x\right)< 0\) có các nghiệm nguyên: \(x=\left\{-2;-1;0;1;2\right\}\)