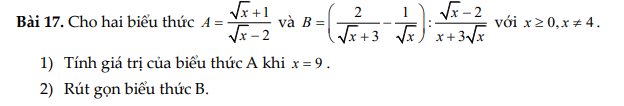a.
\(x=9\Rightarrow A=\dfrac{\sqrt{9}+1}{\sqrt{9}-2}=\dfrac{3+1}{3-2}=4\)
b.
\(B=\left(\dfrac{2\sqrt{x}}{\sqrt{x}\left(\sqrt{x}+3\right)}-\dfrac{\sqrt{x}+3}{\sqrt{x}\left(\sqrt{x}+3\right)}\right):\dfrac{\sqrt{x}-2}{\sqrt{x}\left(\sqrt{x}+3\right)}\)
\(=\left(\dfrac{2\sqrt{x}-\sqrt{x}-3}{\sqrt{x}\left(\sqrt{x}+3\right)}\right).\dfrac{\sqrt{x}\left(\sqrt{x}+3\right)}{\sqrt{x}-2}\)
\(=\dfrac{\left(\sqrt{x}-3\right)}{\sqrt{x}\left(\sqrt{x}+3\right)}.\dfrac{\sqrt{x}\left(\sqrt{x}+3\right)}{\left(\sqrt{x}-2\right)}\)
\(=\dfrac{\sqrt{x}-3}{\sqrt{x}-2}\)
c.
\(M=\dfrac{A}{B}=\dfrac{\sqrt{x}+1}{\sqrt{x}-2}.\dfrac{\sqrt{x}-2}{\sqrt{x}-3}=\dfrac{\sqrt{x}+1}{\sqrt{x}-3}=\dfrac{\sqrt{x}-3+4}{\sqrt{x}-3}=1+\dfrac{4}{\sqrt{x}-3}\)
Do M nguyên \(\Rightarrow\dfrac{4}{\sqrt{x}-3}\) nguyên
\(\Rightarrow\sqrt{x}-3\inƯ\left(4\right)\)
Mà \(\sqrt{x}-3\ge-3;\forall x\ge0\)
\(\Rightarrow\sqrt{x}-3\in\left\{-2;-1;1;2;4\right\}\)
\(\Rightarrow\sqrt{x}\in\left\{1;2;4;5;7\right\}\)
\(\Rightarrow x\in\left\{1;4;16;25;49\right\}\)