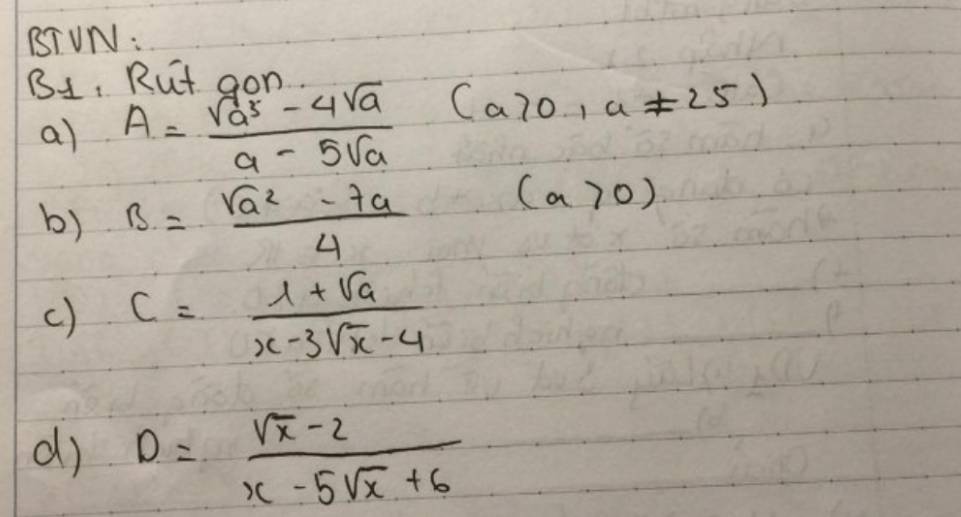a: \(A=\dfrac{a\cdot\sqrt{a}-4\sqrt{a}}{\sqrt{a}\left(\sqrt{a}-5\right)}=\dfrac{\sqrt{a}\left(a-4\right)}{\sqrt{a}\left(\sqrt{a}-5\right)}=\dfrac{a-4}{\sqrt{a}-5}\)
b: \(B=\dfrac{\left|a\right|-7a}{4}\)
mà a>0
nên \(B=\dfrac{a-7a}{4}=-\dfrac{6a}{4}=-\dfrac{3}{2}a\)
c: \(=\dfrac{1+\sqrt{x}}{x-4\sqrt{x}+\sqrt{x}-4}\)
\(=\dfrac{\left(\sqrt{x}+1\right)}{\left(\sqrt{x}-4\right)\left(\sqrt{x}+1\right)}=\dfrac{1}{\sqrt{x}-4}\)
d: \(=\dfrac{\sqrt{x}-2}{x-2\sqrt{x}-3\sqrt{x}+6}\)
\(=\dfrac{\sqrt{x}-2}{\left(\sqrt{x}-2\right)\left(\sqrt{x}-3\right)}\)
\(=\dfrac{1}{\sqrt{x}-3}\)
a) \(A=\dfrac{a\sqrt{a}-4\sqrt{a}}{a-5\sqrt{a}}\)
\(A=\dfrac{a\cdot\sqrt{a}-4\cdot\sqrt{a}}{\left(\sqrt{a}\right)^2-5\cdot\sqrt{a}}\)
\(A=\dfrac{\sqrt{a}\cdot\left(a-4\right)}{\sqrt{a}\cdot\left(\sqrt{a}-5\right)}\)
\(A=\dfrac{a-4}{\sqrt{a}-5}\)
b) \(B=\dfrac{\sqrt{a^2}-7a}{4}\)
\(B=\dfrac{\left|a\right|-7a}{4}\) (Do a>0 nên |a| = a)
\(B=\dfrac{a-7a}{4}\)
\(B=-\dfrac{6a}{4}\)
\(B=-\dfrac{3a}{2}\)
c) \(C=\dfrac{1+\sqrt{x}}{x-3\sqrt{x}-4}\)
\(C=\dfrac{\sqrt{x}+1}{x-4\sqrt{x}+\sqrt{x}-4}\)
\(C=\dfrac{\sqrt{x}+1}{\sqrt{x}\left(\sqrt{x}-4\right)+\left(\sqrt{x}-4\right)}\)
\(C=\dfrac{\sqrt{x}+1}{\left(\sqrt{x}+1\right)\left(\sqrt{x}-4\right)}\)
\(C=\dfrac{1}{\sqrt{x}-4}\)
d) \(D=\dfrac{\sqrt{x}-2}{x-5\sqrt{x}+6}\)
\(D=\dfrac{\sqrt{x}-2}{x-2\sqrt{x}-3\sqrt{x}+6}\)
\(D=\dfrac{\sqrt{x}-2}{\sqrt{x}\left(\sqrt{x}-2\right)-3\left(\sqrt{x}-2\right)}\)
\(D=\dfrac{\sqrt{x}-2}{\left(\sqrt{x}-2\right)\left(\sqrt{x}-3\right)}\)
\(D=\dfrac{1}{\sqrt{x}-3}\)