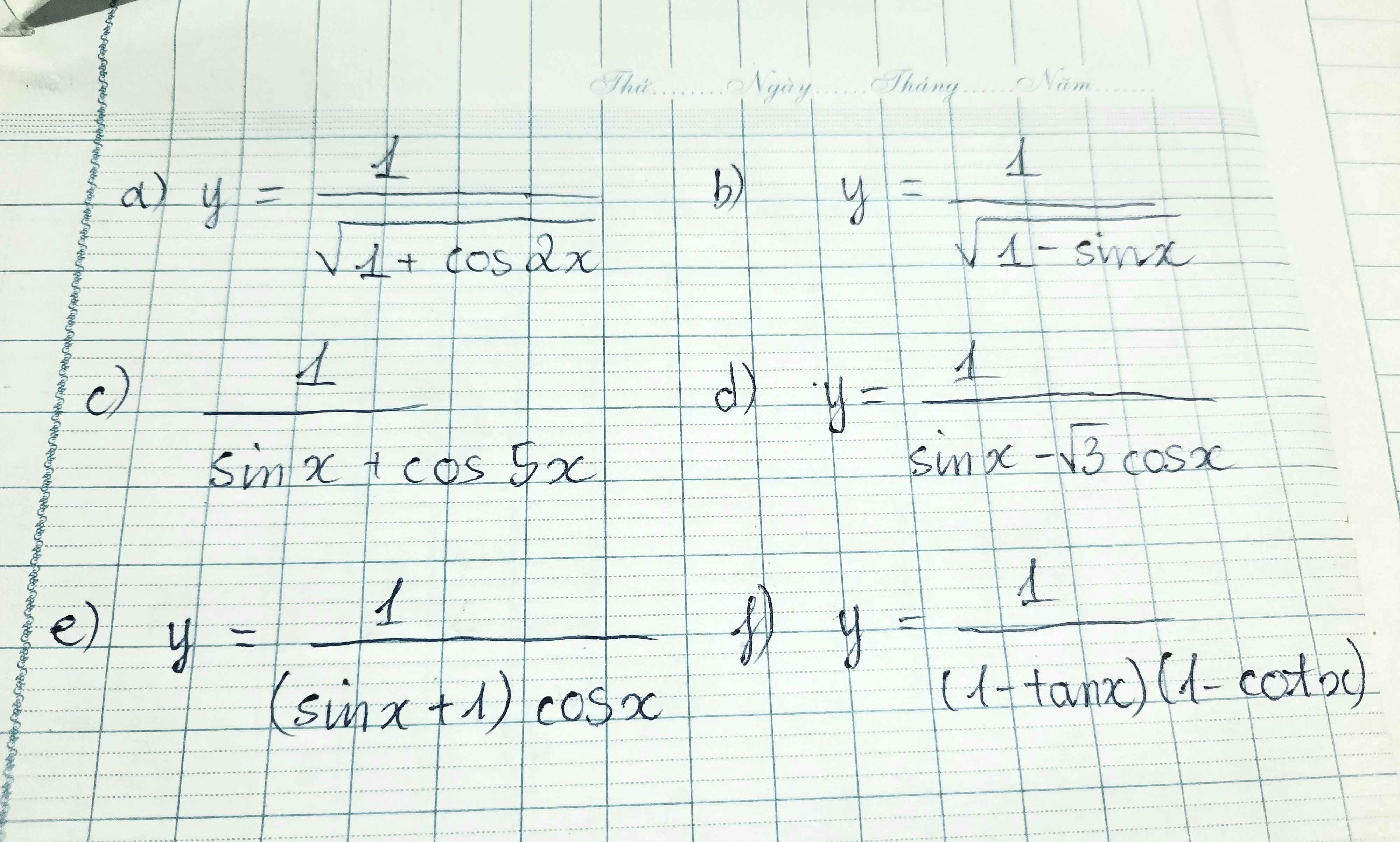Giải giúp mình với, mình cần gấp. Thank you!!!
a) Xác định hàm số y= ax+b ( a# 0) biết nó cắt đường thẳng y=-x+2 tại một điểm trên trục tung và đi qua điểm A (1;3)
b) Tìm điểm trên đường thẳng y=-x+2 có hoành độ gấp 3 lần tung độ
c) Xác định hàm số y= ax+b biết nó cắt Oy tại điểm có tung độ -2 và đi qua A