Làm bài 7 chi tiết ạ cả 2 càng tốt ạ
Phép nhân và phép chia các đa thức
Bài 8:
a) \(x^2-25x=0\)
\(\Leftrightarrow x\left(x-25\right)=0\)
\(\Leftrightarrow\left[{}\begin{matrix}x=0\\x-25=0\end{matrix}\right.\)
\(\Leftrightarrow\left[{}\begin{matrix}x=0\\x=25\end{matrix}\right.\)
b) \(7x\left(x-3\right)-5\left(3-x\right)=0\)
\(\Leftrightarrow7x\left(x-3\right)+5\left(x-3\right)=0\)
\(\Leftrightarrow\left(x-3\right)\left(7x+5\right)=0\)
\(\Leftrightarrow\left[{}\begin{matrix}x-3=0\\7x+5=0\end{matrix}\right.\)
\(\Leftrightarrow\left[{}\begin{matrix}x=3\\x=-\dfrac{5}{7}\end{matrix}\right.\)
c) \(7x\left(x+4\right)-7x-28=0\)
\(\Leftrightarrow7x\left(x+4\right)-7\left(x+4\right)=0\)
\(\Leftrightarrow7\left(x+4\right)\left(x-1\right)=0\)
\(\Leftrightarrow\left[{}\begin{matrix}x+4=0\\x-1=0\end{matrix}\right.\)
\(\Leftrightarrow\left[{}\begin{matrix}x=-4\\x=1\end{matrix}\right.\)
d) \(\left(2x^2+5x\right)\left(x^2-x\right)+\left(2x^2+10\right)\left(x-x^2\right)=0\)
\(\Leftrightarrow\left(2x^2+5x\right)\left(x^2-x\right)-\left(2x^2+10\right)\left(x^2-x\right)=0\)
\(\Leftrightarrow\left(x^2-x\right)\left(2x^2+5x-2x^2-10\right)=0\)
\(\Leftrightarrow x\left(x-1\right)\left(5x-10\right)=0\)
\(\Leftrightarrow\left[{}\begin{matrix}x=0\\x-1=0\\5x-10=0\end{matrix}\right.\)
\(\Leftrightarrow\left[{}\begin{matrix}x=0\\x=1\\x=2\end{matrix}\right.\)
Đúng 2
Bình luận (2)
7:
a: \(4a^3b-12a^2b^2+8ab^3\)
\(=4ab\cdot a^2-4ab\cdot3ab+4ab\cdot2b^2\)
\(=4ab\left(a^2-3ab+2b^2\right)\)
\(=4ab\left(a^2-ab-2ab+2b^2\right)\)
\(=4ab\left[a\left(a-b\right)-2b\left(a-b\right)\right]\)
\(=4ab\left(a-b\right)\left(a-2b\right)\)
b: \(\dfrac{5}{2}x^4+\dfrac{3}{4}x^3-\dfrac{1}{5}x^2\)
\(=x^2\cdot\dfrac{5}{2}x^2+x^2\cdot\dfrac{3}{4}x-x^2\cdot\dfrac{1}{5}\)
\(=x^2\left(\dfrac{5}{2}x^2+\dfrac{3}{4}x-\dfrac{1}{5}\right)\)
c: \(3x^2\left(x+9\right)-2\left(x+9\right)\)
\(=\left(x+9\right)\cdot3x^2-\left(x+9\right)\cdot2\)
\(=\left(x+9\right)\left(3x^2-2\right)\)
d: \(3x^2\left(7x+y\right)-5x\left(7x+y\right)+14x+2y\)
\(=\left(7x+y\right)\left(3x^2-5x\right)+2\left(7x+y\right)\)
\(=\left(7x+y\right)\left(3x^2-5x+2\right)\)
\(=\left(7x+y\right)\left(3x^2-3x-2x+2\right)\)
\(=\left(7x+y\right)\left[3x\left(x-1\right)-2\left(x-1\right)\right]\)
\(=\left(7x+y\right)\left(x-1\right)\left(3x-2\right)\)
Đúng 1
Bình luận (0)
Làm bài 12 giúp ạ chi tiết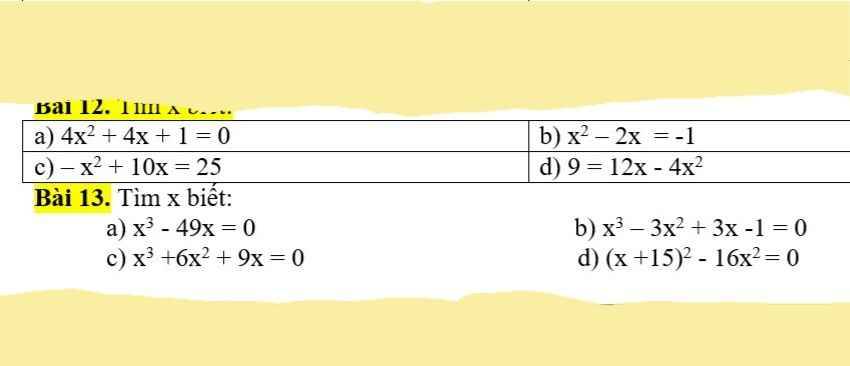
Bài 12:
a) \(4x^2+4x+1=0\)
\(\Leftrightarrow\left(2x\right)^2+2\cdot2x\cdot1+1^2=0\)
\(\Leftrightarrow\left(2x+1\right)^2=0\)
\(\Leftrightarrow2x+1=0\)
\(\Leftrightarrow x=-\dfrac{1}{2}\)
b) \(x^2-2x=-1\)
\(\Leftrightarrow x^2-2x+1=0\)
\(\Leftrightarrow\left(x-1\right)^2=0\)
\(\Leftrightarrow x-1=0\)
\(\Leftrightarrow x=1\)
c) \(-x^2+10x=25\)
\(\Leftrightarrow-x^2+10x-25=0\)
\(\Leftrightarrow-\left(x^2-10x+25\right)=0\)
\(\Leftrightarrow-\left(x-5\right)^2=0\)
\(\Leftrightarrow x-5=0\)
\(\Leftrightarrow x=5\)
d) \(9=12x-4x^2\)
\(\Leftrightarrow4x^2-12x+9=0\)
\(\Leftrightarrow\left(2x-3\right)^2=0\)
\(\Leftrightarrow2x-3=0\)
\(\Leftrightarrow2x=3\)
\(\Leftrightarrow x=\dfrac{3}{2}\)
Đúng 1
Bình luận (0)
Bài 7:
a) \(4a^3b-12a^2b^2+8ab^3\)
\(=4ab\left(a^2-3ab+2b^2\right)\)
\(=4ab\left(a^2-ab-2ab+2b^2\right)\)
\(=4ab\left(a-b\right)\left(a-2b\right)\)
b) \(\dfrac{5}{4}x^4+\dfrac{3}{4}x^3-\dfrac{1}{5}x^2\)
\(=x^2\cdot\left(\dfrac{5}{4}x^2+\dfrac{3}{4}x-\dfrac{1}{5}\right)\)
\(=x^2\cdot\dfrac{1}{4}\cdot\left(5x^2+3x-\dfrac{4}{5}\right)\)
\(=\dfrac{x^2}{4}\cdot\left(5x^2+3x-\dfrac{4}{5}\right)\)
c) \(3x^2\left(x+9\right)-2\left(x+9\right)\)
\(=\left(x+9\right)\left(3x^2-2\right)\)
d) \(3x^2\left(7x+y\right)-5x\left(7x+y\right)+14x+2y\)
\(=3x^2\left(7x+y\right)-5x\left(7x+y\right)+2\left(7x+y\right)\)
\(=\left(7x+y\right)\left(3x^2-5x+2\right)\)
\(=\left(7x+y\right)\left(3x^2-3x-2x-2\right)\)
\(=\left(7x+y\right)\left(3x-2\right)\left(x-1\right)\)
Đúng 2
Bình luận (0)
Bài 8:
a: \(x^2-25x=0\)
=>\(x\left(x-25\right)=0\)
=>\(\left[{}\begin{matrix}x=0\\x-25=0\end{matrix}\right.\Leftrightarrow\left[{}\begin{matrix}x=0\\x=25\end{matrix}\right.\)
b: \(7x\left(x-3\right)-5\left(3-x\right)=0\)
=>\(7x\left(x-3\right)+5\left(x-3\right)=0\)
=>\(\left(x-3\right)\left(7x+5\right)=0\)
=>\(\left[{}\begin{matrix}x-3=0\\7x+5=0\end{matrix}\right.\Leftrightarrow\left[{}\begin{matrix}x=3\\x=-\dfrac{5}{7}\end{matrix}\right.\)
c: \(7x\left(x+4\right)-7x-28=0\)
=>\(7x\left(x+4\right)-7\left(x+4\right)=0\)
=>\(x\left(x+4\right)-\left(x+4\right)=0\)
=>\(\left(x+4\right)\left(x-1\right)=0\)
=>\(\left[{}\begin{matrix}x+4=0\\x-1=0\end{matrix}\right.\Leftrightarrow\left[{}\begin{matrix}x=-4\\x=1\end{matrix}\right.\)
d: \(\left(2x^2+5x\right)\left(x^2-x\right)+\left(2x^2+10\right)\left(x-x^2\right)=0\)
=>\(\left(2x^2+5x\right)\left(x^2-x\right)-\left(2x^2+10\right)\left(x^2-x\right)=0\)
=>\(\left(x^2-x\right)\left(2x^2+5x-2x^2-10\right)=0\)
=>\(5\left(x-2\right)\cdot x\left(x-1\right)=0\)
=>\(x\left(x-1\right)\left(x-2\right)=0\)
=>\(\left[{}\begin{matrix}x=0\\x-1=0\\x-2=0\end{matrix}\right.\Leftrightarrow\left[{}\begin{matrix}x=0\\x=1\\x=2\end{matrix}\right.\)
Đúng 1
Bình luận (0)
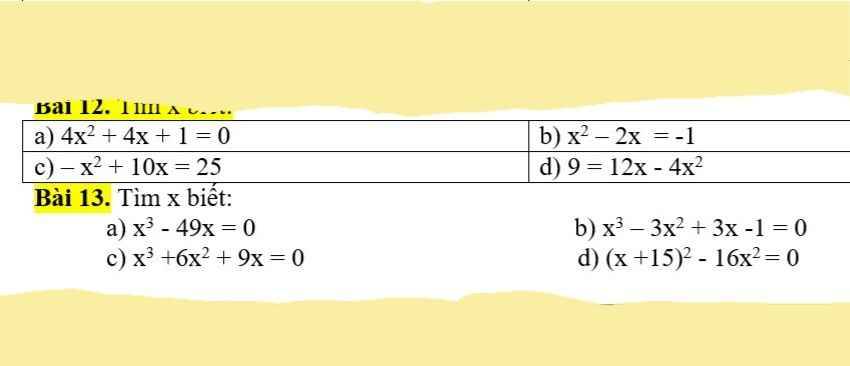 chi tiết nhất ạ
chi tiết nhất ạ
Bài 13:
a) \(x^3-49x=0\)
\(\Leftrightarrow x\left(x^2-49\right)=0\)
\(\Leftrightarrow x\left(x+7\right)\left(x-7\right)=0\)
\(\Leftrightarrow\left[{}\begin{matrix}x=0\\x+7=0\\x-7=0\end{matrix}\right.\)
\(\Leftrightarrow\left[{}\begin{matrix}x=0\\x=-7\\x=7\end{matrix}\right.\)
b) \(x^3-3x^2+3x-1=0\)
\(\Leftrightarrow x^3-3\cdot x^2\cdot1+3\cdot x\cdot1^2-1^3=0\)
\(\Leftrightarrow\left(x-1\right)^3=0\)
\(\Leftrightarrow x-1=0\)
\(\Leftrightarrow x=1\)
c) \(x^3+6x^2+9x=0\)
\(\Leftrightarrow x\left(x^2+6x+9\right)=0\)
\(\Leftrightarrow x\left(x^2+2\cdot3\cdot x+3^2\right)=0\)
\(\Leftrightarrow x\left(x+3\right)^2=0\)
\(\Leftrightarrow\left[{}\begin{matrix}x=0\\x+3=0\end{matrix}\right.\)
\(\Leftrightarrow\left[{}\begin{matrix}x=0\\x=-3\end{matrix}\right.\)
d) \(\left(x+5\right)^2-16x^2=0\)
\(\Leftrightarrow\left(x+5\right)^2-\left(4x\right)^2=0\)
\(\Leftrightarrow\left(x+5-4x\right)\left(x+5+4x\right)=0\)
\(\Leftrightarrow\left(5-3x\right)\left(5x+5\right)=0\)
\(\Leftrightarrow\left[{}\begin{matrix}5-3x=0\\5x+5=0\end{matrix}\right.\)
\(\Leftrightarrow\left[{}\begin{matrix}3x=5\\5x=-5\end{matrix}\right.\)
\(\Leftrightarrow\left[{}\begin{matrix}x=\dfrac{5}{3}\\x=-1\end{matrix}\right.\)
Đúng 1
Bình luận (0)
12:
a: \(4x^2+4x+1=0\)
=>\(\left(2x\right)^2+2\cdot2x\cdot1+1^2=0\)
=>\(\left(2x+1\right)^2=0\)
=>2x+1=0
=>2x=-1
=>\(x=-\dfrac{1}{2}\)
b: \(x^2-2x=-1\)
=>\(x^2-2x+1=0\)
=>\(x^2-2\cdot x\cdot1+1^2=0\)
=>\(\left(x-1\right)^2=0\)
=>x-1=0
=>x=1
c: \(-x^2+10x=25\)
=>\(-x^2+10x-25=0\)
=>\(x^2-10x+25=0\)
=>\(x^2-2\cdot x\cdot5+5^2=0\)
=>\(\left(x-5\right)^2=0\)
=>x-5=0
=>x=5
d: \(12x-4x^2=9\)
=>\(-4x^2+12x-9=0\)
=>\(4x^2-12x+9=0\)
=>\(\left(2x\right)^2-2\cdot2x\cdot3+3^2=0\)
=>\(\left(2x-3\right)^2=0\)
=>2x-3=0
=>2x=3
=>x=3/2
Đúng 1
Bình luận (0)
22x22=
(8x3)2=
\(2^2\cdot2^2=2^{2+2}=2^4=16\)
\(\left(8\cdot3\right)^2=8^2\cdot3^2=64\cdot9=576\)
Đúng 0
Bình luận (0)
Tìm thương của phép chia: [9x^3 (x^2 - 1) − 6x^2 (x^2 - 1)^2 + 12x(x^2 -1)]: 3x(x^2 - 1). Giúp mik vs, mik cần gấp!!
[9x³(x² - 1) - 6x²(x² - 1) + 12x(x² - 1)] : 3x(x² - 1)
= [9x³(x² - 1) : 3x(x² - 1)] - [6x²(x² - 1) : 3x(x² - 1) + [12x(x² - 1) : 3x(x² - 1)]
= 3x² - 2x + 4
Đúng 2
Bình luận (0)
hạp mi với ạ
2:
a: \(\left(3x-1\right)\left(3x-1\right)+9\left(1-x\right)\left(x+1\right)=12\)
=>\(\left(3x-1\right)^2-9\left(x-1\right)\left(x+1\right)=12\)
=>\(9x^2-6x+1-9\left(x^2-1\right)=12\)
=>\(9x^2-6x+1-9x^2+9=12\)
=>-6x+10=12
=>-6x=2
=>x=-1/3
b: \(4\left(x+1\right)^2-\left(2x-1\right)^2=0\)
=>\(4\left(x^2+2x+1\right)-4x^2+4x-1=0\)
=>\(4x^2+8x+4-4x^2+4x-1=0\)
=>12x+3=0
=>x=-1/4
3:
a: Xét ΔODC có AB//CD
nên \(\dfrac{OA}{AD}=\dfrac{OB}{BC}\)
mà AD=BC
nên OA=OB
=>ΔOAB cân tại O
b: Xét ΔABD và ΔBAC có
BA chung
BD=AC
AD=BC
Do đó: ΔABD=ΔBAC
c: ΔABD=ΔBAC
=>\(\widehat{EAB}=\widehat{EBA}\)
=>EA=EB
EA+EC=AC
EB+ED=BD
mà AC=BD và EA=EB
nên EC=ED
=>ΔECD cân tại E
d: OA+AD=OD
OB+BC=OC
mà OA=OB và AD=BC
nên OD=OC
OD=OC
ED=EC
Do đó: OE là đường trung trực của CD
OA=OB
EA=EB
Do đó: OE là đường trung trực của AB
Đúng 2
Bình luận (1)
câu 1. (1,5đ) cho hai đa thức sau: P=x^2y+2x^3-xy^2+5 Q=x^3+xy^2-2x^2y-6 a) tính tổng của đa thức p và q. b) tìm đa thức n sao cho q = p + n.
a) P + Q = (x² + 2x³ - xy² + 5) + (x³ + xy² - 2x²y - 6)
= x² + 2x³ - xy² + 5 + x³ + xy² - 2x²y - 6
= (2x³ + x³) + x² + (-xy² + xy²) - 2x²y + (5 - 6)
= 3x³ + x² - 2x²y - 1
b) Q = P + N
N = Q - P
= (x³ + xy² - 2x²y - 6) - (x² + 2x³ - xy² + 5)
= x³ + xy² - 2x²y - 6 - x² - 2x³ + xy² - 5
= (x³ - 2x³) + (xy² + xy²) - 2x²y - x² + (-6 - 5)
= -x³ + 2xy² - 2x²y - x² - 11
Vậy N = -x³ + 2xy² - 2x²y - x² - 11
Đúng 0
Bình luận (0)
Tính tổng hai đa thức P và Q rồi tìm bậc của đa thức tổng
Đúng 0
Bình luận (0)
Bài 5.5: Tìm x: (2x-3)(x+1)+(4x^3-6x^2-6x):(-2x)18Bài 6.1: Tìm số tự nhiên n để: 5x^{n-2}:3x^2Bài 6.2: Tìm số tự nhiên n để đa thức x^{n-1}-3x^2:2x^2Bài 6.3: Tìm n ∈ N để phép tính chia sau là phép chia hết:3x^7y^7-4x^6y^6-5x^3y^3:(2x^ny^n)Trả lời nhanh giúp mìn nhóe!
Đọc tiếp
Bài 5.5: Tìm x: (2x-3)(x+1)+(4x\(^3\)-6x\(^2\)-6x):(-2x)=18
Bài 6.1: Tìm số tự nhiên n để: 5x\(^{n-2}\):3x\(^2\)
Bài 6.2: Tìm số tự nhiên n để đa thức x\(^{n-1}\)-3x\(^2\):2x\(^2\)
Bài 6.3: Tìm n ∈ N để phép tính chia sau là phép chia hết:
3x\(^7\)y\(^7\)-4x\(^6\)y\(^6\)-5x\(^3\)y\(^3\):(2x\(^n\)y\(^n\))
Trả lời nhanh giúp mìn nhóe!![]()
Bài 5.5:
\(\left(2x-3\right)\left(x+1\right)+\left(4x^3-6x^2-6x\right):\left(-2x\right)=18\)
\(\Leftrightarrow\left(2x^2+2x-3x-3\right)+2x\cdot\left(2x^2-3x-3\right):\left(-2x\right)=18\)
\(\Leftrightarrow2x^2-x-3-2x^2+3x+3=18\)
\(\Leftrightarrow2x=18\)
\(\Leftrightarrow x=\dfrac{18}{2}\)
\(\Leftrightarrow x=9\)
Đúng 1
Bình luận (0)
Bài 7:
a) A = (x - 2)(x - 2) - (x - 1)(x + 1)
= x² - 2x - 2x + 4 - x² - x + x + 1
= (x² - x²) + (-2x - 2x - x + x) + (4 + 1)
= -4x + 5
Tại x = 21 thì
A = -4.21 + 5
= -77
b) B = (x - 1)(x - 7) - (2x - 6)(x - 1)
= x² - 7x - x + 7 - 2x² + 2x + 6x - 6
= (x² - 2x²) + (-7x - x + 2x + 6x) + (7 - 6)
= -x² + 1
Tại x = 0 thì
B = -0² + 1
= 1
c) C = (2x + y)(2 + y) + (3x + y)(y - 2)
= 4x + 2xy + 2y + y² + 3xy - 6x + y² - 2y
= (4x - 6x) + (2xy + 3xy) + (2y - 2y) + (y² + y²)
= -2x + 5xy + 2y²
Tại x = 1; y = -1 thì
C = -2.1 + 5.1.(-1) + 2.(-1)²
= -5
d) D = (x - 1)(x + 2) - x(x - 2)
= x² + 2x - x - 2 - x² + 2x
= (x² - x²) + (2x - x + 2x) - 2
= 3x - 2
Tại x = 100 thì
D = 3.100 - 2
= 298
Đúng 1
Bình luận (0)
Bài 8
1) A = x³ - 30x² - 31x + 1
= x³ + x² - 31x² - 31x + 1
= (x³ + x²) - (31x² + 31x) + 1
= x²(x + 1) - 31x(x + 1) + 1
Tại x = 31 thì
A = 31².32 - 31².32 + 1
= 1
2) B = x³ - 17x² - 18x + 20
= x³ + x² - 18x² - 18x + 20
= (x³ + x²) - (18x² + 18x) + 20
= x²(x + 1) - 18x(x + 1) + 20
Tại x = 20 thì
B = 18².19 - 18².19 + 20
= 20
3) C = x⁴ - 17x³ + 17x² - 17x + 20
= x⁴ - x³ - 16x³ + x² + 16x² - x + 16 - 16x
= (x⁴ - x³) - (16x³ - 16x²) + (x² - x) - (16x - 16)
= x³(x - 1) - 16x²(x - 1) + x(x - 1) - 16(x - 1)
Tại x = 16 thì
C = 16³.15 - 16³.15 + 16.15 - 16.15
= 0
4) D = x⁴ + 10x³ + 10x² + 10x + 10
= x⁴ + x³ + 9x³ + 9x² + x² + x + 9x + 9 + 1
= (x⁴ + x³) + (9x³ + 9x²) + (x² + x) + (9x + 9) + 1
= x³(x + 1) + 9x²(x + 1) + x(x + 1) + 9(x + 1) + 1
Tại x = -9 thì
D = (-9)³.(-8) + 9³.(-8) - 9.(-8) + 9.(-8) + 1
= 1
Đúng 0
Bình luận (0)





