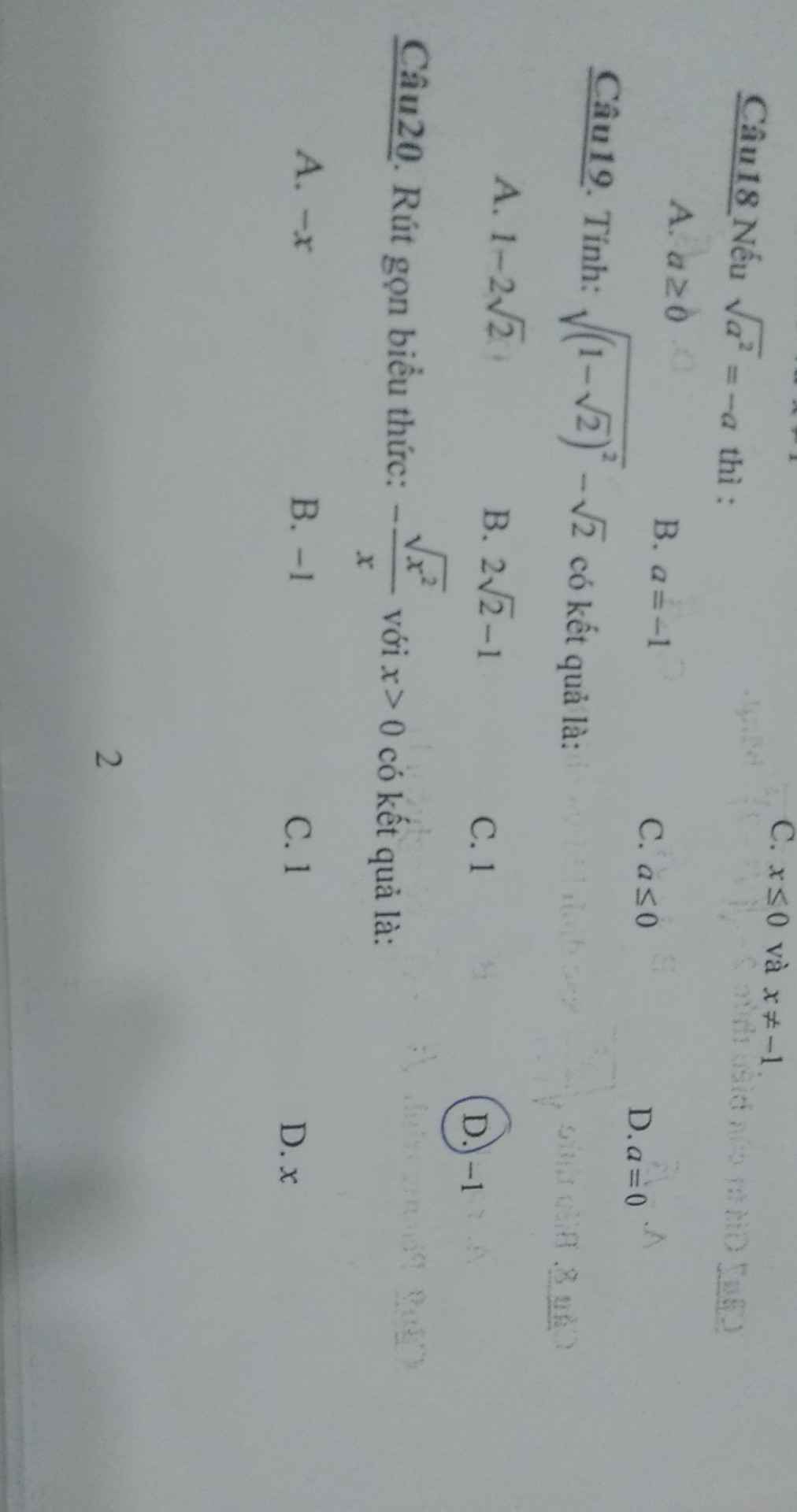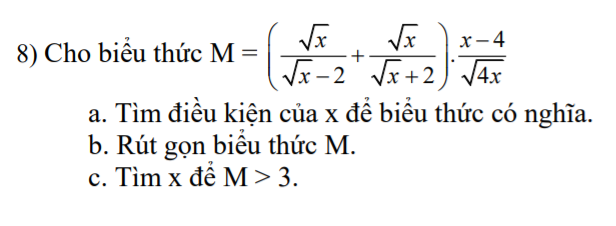a: \(A=\dfrac{x\sqrt{x}+1-\left(x-1\right)\left(\sqrt{x}-1\right)}{x-1}\)
\(=\dfrac{x\sqrt{x}+1-x\sqrt{x}+x+\sqrt{x}-1}{x-1}=\dfrac{x+\sqrt{x}}{x-1}=\dfrac{\sqrt{x}}{\sqrt{x}-1}\)
b: Thay x=1/4 vào A, ta được:
\(A=\dfrac{\dfrac{1}{2}}{\dfrac{1}{2}-1}=\dfrac{1}{2}:\dfrac{-1}{2}=-1\)
c: Để A<0 thì \(\sqrt{x}-1< 0\)
hay 0<=x<1
d: Để |A|=A thì A>=0
=>\(\sqrt{x}-1>=0\)
hay x>1
Bài 4:
a) ĐKXĐ: \(x\ne1;x\ge0\)
\(A=\dfrac{x\sqrt{x}+1}{x-1}-\dfrac{x-1}{\sqrt{x}+1}\)
\(=\dfrac{x\sqrt{x}+1-x\sqrt{x}+x+\sqrt{x}-1}{\left(\sqrt{x}-1\right)\left(\sqrt{x}+1\right)}\)
\(=\dfrac{\sqrt{x}\left(\sqrt{x}+1\right)}{\left(\sqrt{x}-1\right)\left(\sqrt{x}+1\right)}\)
\(=\dfrac{\sqrt{x}}{\sqrt{x}-1}\)
b) \(\left|x\right|=\dfrac{1}{4}\Leftrightarrow\left[{}\begin{matrix}x=\dfrac{1}{4}\\x=-\dfrac{1}{4}\end{matrix}\right.\)
Thay \(x=\dfrac{1}{4}\) vào \(A=\dfrac{\sqrt{x}}{\sqrt{x}-1}\) ta có:
\(A=\dfrac{\sqrt{\dfrac{1}{4}}}{\sqrt{\dfrac{1}{4}}-1}=-1\)
Thay \(x=-\dfrac{1}{4}\) vào \(A=\dfrac{\sqrt{x}}{\sqrt{x}-1}\) ta có:
\(A=\dfrac{\sqrt{-\dfrac{1}{3}}}{\sqrt{-\dfrac{1}{4}}-1}=-1\) (vô lí, vì \(\sqrt{x}\ge0\forall x\))
Vậy \(A=-1\) khi \(\left|x\right|=\dfrac{1}{4}\)
c) Để \(A< 0\) thì \(\dfrac{\sqrt{x}}{\sqrt{x}-1}< 0\)
Mà \(\sqrt{x}>0\) (luôn dương \(\forall x\))
\(\Rightarrow\left(\sqrt{x}-1\right)< 0\)
\(\Leftrightarrow0\le x< 1\)
Vậy \(0\le x< 1\) thì \(A< 0\)
d) Ta có: \(\left|A\right|=A\) (ĐK: \(A>0\))
\(\Rightarrow\dfrac{\sqrt{x}}{\sqrt{x}-1}>0\)
\(\Rightarrow\sqrt{x}-1>0\)
\(\Leftrightarrow x>1\)
Vậy \(x>1\) thì \(\left|A\right|=A\)






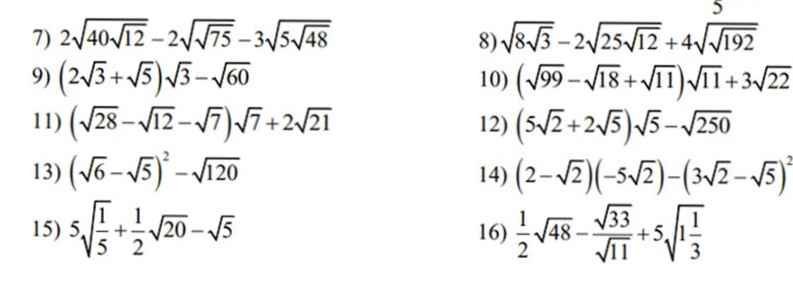

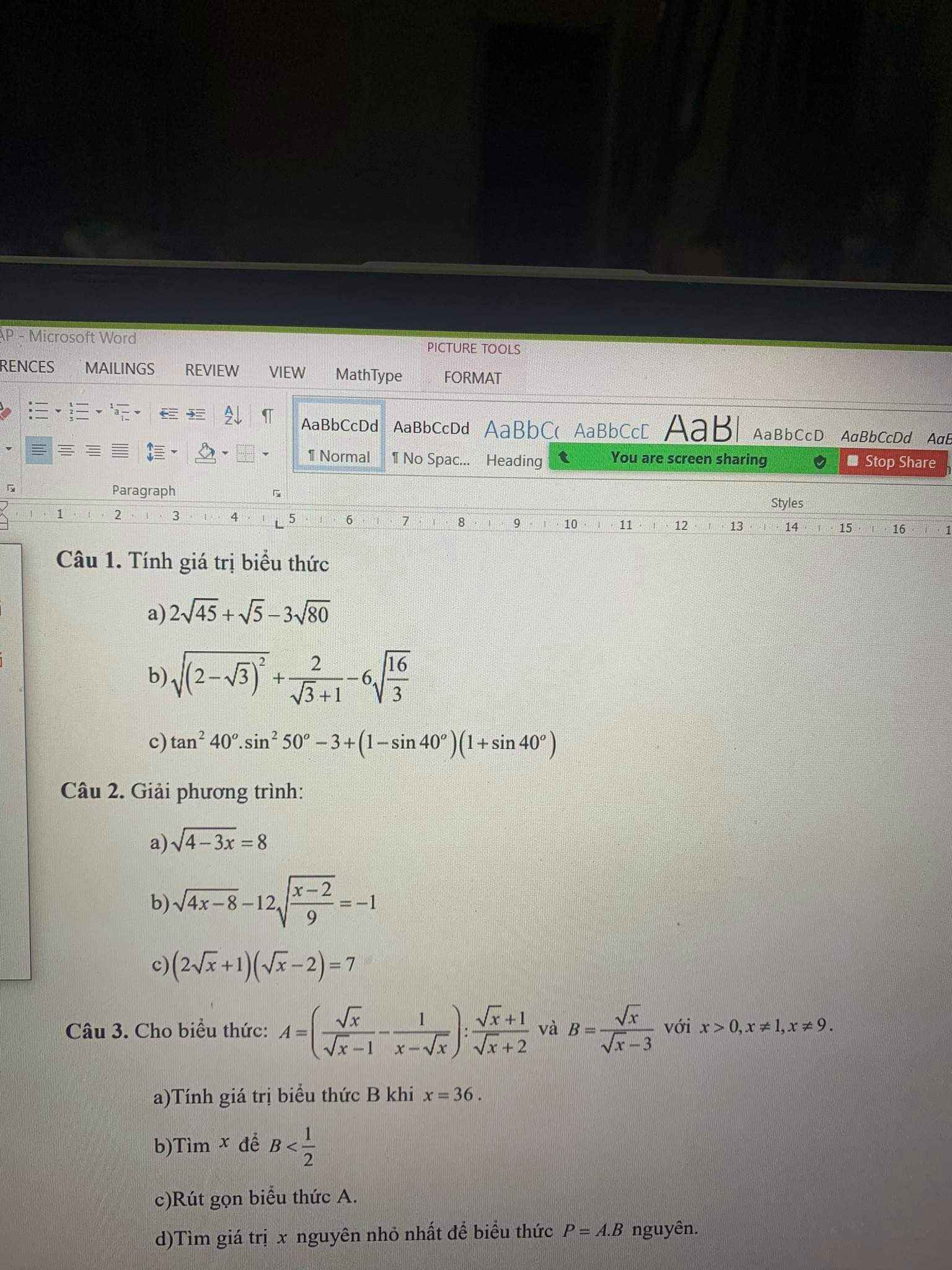
 Mn giúp mình bài 3 với ạ. Mình đang cần gấp lắm. Mình cảm ơn nhiều !
Mn giúp mình bài 3 với ạ. Mình đang cần gấp lắm. Mình cảm ơn nhiều !