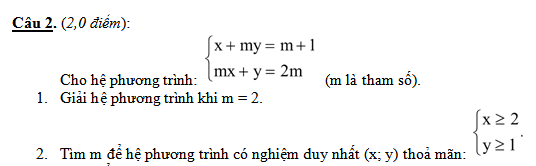\(\Rightarrow\left\{{}\begin{matrix}x+my=m+1\\m^2x+my=2m^2\end{matrix}\right.\)
\(\Rightarrow\left\{{}\begin{matrix}x+my=m+1\\\left(m^2-1\right)x=2m^2-m-1\end{matrix}\right.\)
Phương trình có nghiệm duy nhất khi \(m^2-1\ne0\Rightarrow m\ne\pm1\)
Khi đó ta có: \(x=\dfrac{2m^2-m-1}{m^2-1}=\dfrac{\left(m-1\right)\left(2m+1\right)}{\left(m-1\right)\left(m+1\right)}=\dfrac{2m+1}{m+1}\)
\(\Rightarrow y=2m-mx=\dfrac{m}{m+1}\)
Để \(\left\{{}\begin{matrix}x\ge2\\y\ge1\end{matrix}\right.\) \(\Rightarrow\left\{{}\begin{matrix}\dfrac{2m+1}{m+1}\ge2\\\dfrac{m}{m+1}\ge1\end{matrix}\right.\) \(\Rightarrow\left\{{}\begin{matrix}\dfrac{-1}{m+1}\ge0\\\dfrac{-1}{m+1}\ge0\end{matrix}\right.\)
\(\Rightarrow m+1< 0\Rightarrow m< -1\)