Bài `2`
\(a,4xy+4xz\\ =4x\left(y+z\right)\\ b,x^2-y^2+9-6x\\ =\left(x^2-6x+9\right)-y^2\\ =\left(x-3\right)^2-y^2\\ =\left(x-3-y\right)\left(x-3+y\right)\)
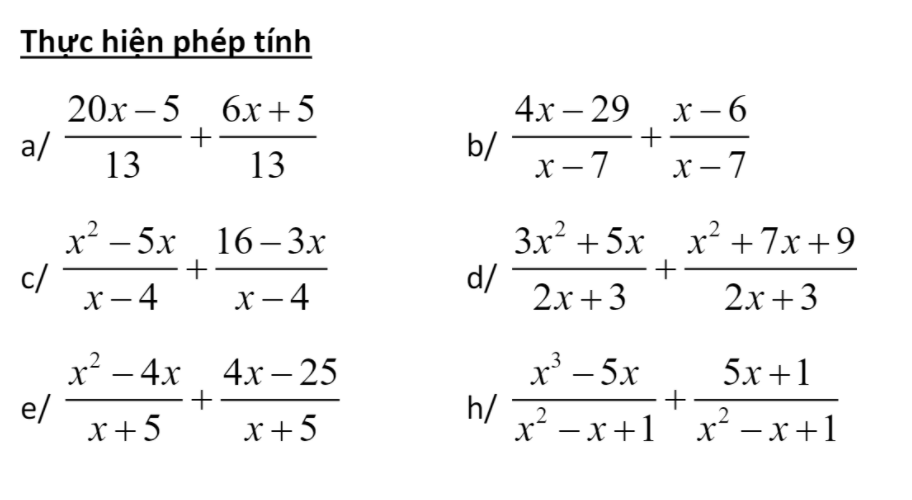
Bài `3`
\(a,\dfrac{3xy}{y+z}+\dfrac{3xz}{y+z}\\ =\dfrac{3xy+3xz}{y+z}\\ =\dfrac{3x\left(y+z\right)}{y+z}\\ =3x\\ b,\dfrac{x}{x+2}-\dfrac{x}{x-2}\\ =\dfrac{x\left(x-2\right)}{\left(x-2\right)\left(x+2\right)}-\dfrac{x\left(x+2\right)}{\left(x-2\right)\left(x+2\right)}\\ =\dfrac{x^2-2x-\left(x^2+2x\right)}{\left(x-2\right)\left(x+2\right)}\\ =\dfrac{x^2-2x-x^2-2x}{\left(x-2\right)\left(x+2\right)}\\ =\dfrac{-4x}{x-4}\)
Bài 5
Xét △ vuông CAB có:
\(CB^2=CA^2+AB^2\) ( Đ/Lí Pythagore )
\(\Rightarrow AB^2=CB^2-AC^2\)
\(\Rightarrow AB^2=13^2-5^2=144\)
\(\Rightarrow AB=\sqrt{144}=12m\)
Vậy chiều cao mà thang có thể vương tới là 12 m
Bài 6
Ta có: \(x^2-2x+y^2-4y+5=0\)
\(\Leftrightarrow x^2-2x+y^2-4y+4+1=0\)
\(\Leftrightarrow\left(x^2-2x+1\right)+\left(y^2-4y+4\right)=0\)\(\Leftrightarrow\left(x-1\right)^2+\left(y-2\right)^2=0\)
\(Mà\left\{{}\begin{matrix}\left(x-1\right)^2\ge0\\\left(y-2\right)^2\ge0\end{matrix}\right.\)
\(\Leftrightarrow\text{}\text{}\left(\text{}x-1\right)^2+\left(y-2\right)^2\ge0\)
\(\Leftrightarrow\left\{{}\begin{matrix}x-1=0\\y-2=0\end{matrix}\right.\)
\(\Leftrightarrow\left\{{}\begin{matrix}x=1\\y=2\end{matrix}\right.\)
Thay x=1; y=2 vào biểu thức A ta được:
\(A=-2x+y\)
\(A=-2.1+2\)
\(A=0\)
Bài 1:
a: \(2x^2y\left(2x^2y^2-xy^2\right)\)
\(=2x^2y\cdot2x^2y^2-2x^2y\cdot xy^2\)
\(=4x^4y^3-2x^3y^3\)
b: \(\left(x-1\right)\left(2x+3\right)\)
\(=2x^2+3x-2x-3\)
\(=2x^2+x-3\)
c: \(\dfrac{20x^3y^4+10x^2y^3-5xy}{5xy}\)
\(=\dfrac{5xy\cdot4x^2y^3+5xy\cdot2xy^2-5xy\cdot1}{5xy}\)
\(=4x^2y^3+2xy^2-1\)
d: \(\left(y-3x\right)^2-\left(y^2-6xy\right)\)
\(=y^2-6xy+9x^2-y^2+6xy\)
\(=9x^2\)