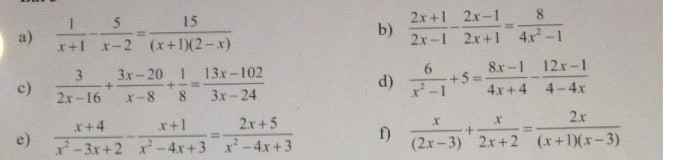a)
\(\dfrac{A}{B}=\dfrac{-13x^{17}y^{2n-3}+22x^{16}y^7}{-7x^{3n+1}y^6}=\dfrac{13}{7}x^{17-\left(3n+1\right)}y^{2n-3-6}-\dfrac{22}{7}x^{16-\left(3n+1\right)}y^{7-6}\\ =\dfrac{13}{7}x^{16-3n}y^{2n-9}-\dfrac{22}{7}x^{15-3n}y\)
Để A chia hết cho b thì: \(\left\{{}\begin{matrix}16-3n\ge0\\2n-9\ge0\\15-3n\ge0\end{matrix}\right.\Leftrightarrow\dfrac{9}{2}\le n\le5\Rightarrow n=5\) (vì n là STN)
b)
\(\dfrac{A}{B}=\dfrac{20x^8y^{2n}-10x^4y^{3n}+15x^5y^6}{3x^{2n}y^{n+1}}\\ =\dfrac{20}{3}x^{8-2n}y^{2n-\left(n+1\right)}-\dfrac{10}{3}x^{4-2n}y^{3n-\left(n+1\right)}+5x^{5-2n}y^{6-\left(n+1\right)}\\ =\dfrac{20}{3}x^{8-2n}y^{n-1}-\dfrac{10}{3}x^{4-2n}y^{2n-1}+5^{5-2n}y^{5-n}\)
Để A chia hết cho B thì:
\(8-2n\ge0;n-1\ge0;4-2n\ge0;2n-1\ge0;5-2n\ge0;5-n\ge0\)
\(\Rightarrow1\le n\le2\Rightarrow n\in\left\{1;2\right\}\)







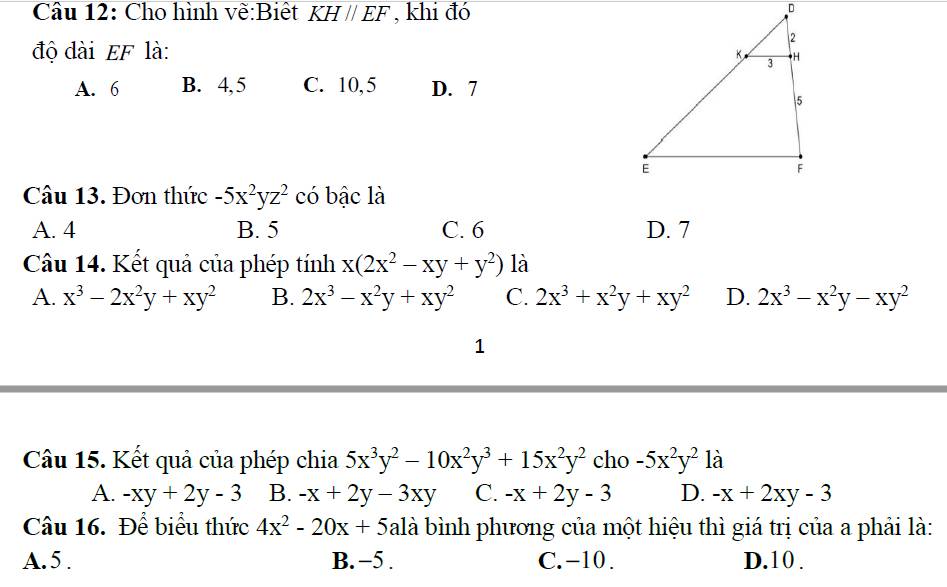
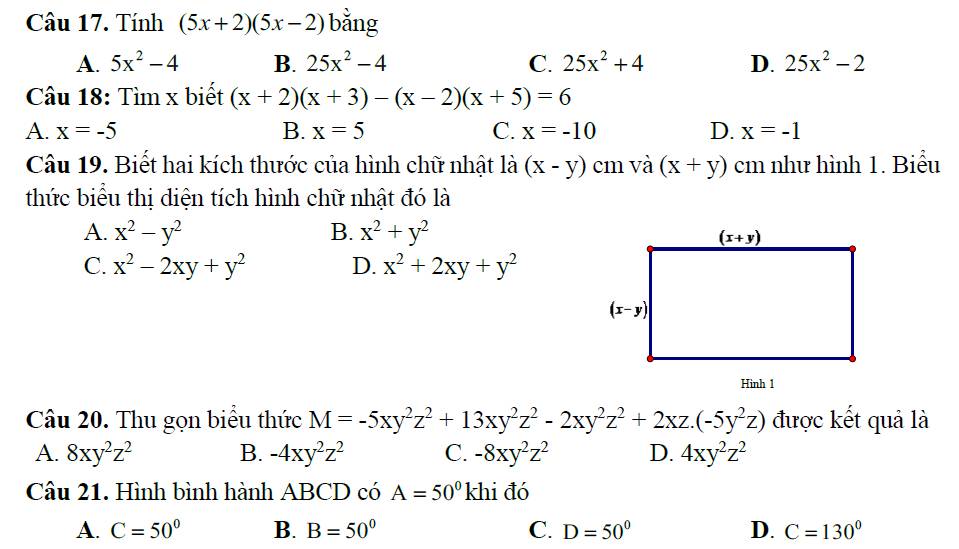
 Giải gấp giúp em với. Giải từng câu và giải thích nhé ạ. Em cảm ơn
Giải gấp giúp em với. Giải từng câu và giải thích nhé ạ. Em cảm ơn