bây gio mk phai đi hoc k kip giai r chiêu nhe
\(\left\{\begin{matrix}\frac{1}{\sqrt{x}}+\sqrt{2-\frac{1}{y}}=2\\\frac{1}{\sqrt{y}}+\sqrt{2-\frac{1}{x}}=2\end{matrix}\right.\)
ĐK: \(\left\{\begin{matrix}x\ge\frac{1}{2}\\y\ge\frac{1}{2}\end{matrix}\right.\)
\(\Leftrightarrow\left\{\begin{matrix}\sqrt{2-\frac{1}{y}}=2-\frac{1}{\sqrt{x}}\\\sqrt{2-\frac{1}{x}}=2-\frac{1}{\sqrt{y}}\end{matrix}\right.\)
\(\Leftrightarrow\left\{\begin{matrix}2-\frac{1}{y}=4-\frac{4}{\sqrt{x}}+\frac{1}{x}\left(1\right)\\2-\frac{1}{x}=4-\frac{4}{\sqrt{y}}+\frac{1}{y}\left(2\right)\end{matrix}\right.\)
Lấy (1) - (2) vế theo vế rồi rút gọn được
\(\frac{4}{\sqrt{x}}=\frac{4}{\sqrt{y}}\)
\(\Leftrightarrow\) x = y
Thế vào (1) ta được
\(\frac{2}{x}-\frac{4}{\sqrt{x}}+2=0\)
\(\Leftrightarrow2\left(\frac{1}{\sqrt{x}}-1\right)^2=0\)
\(\Leftrightarrow x=1\)
\(\Rightarrow y=1\)
 Giải hpt
Giải hpt

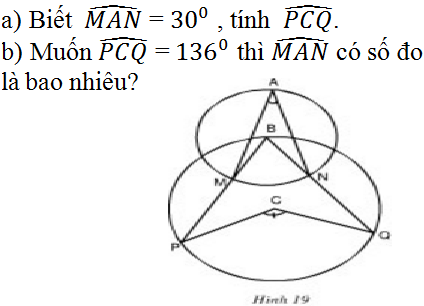
 Giải giúp mình bài 4
Giải giúp mình bài 4 


 Mọi người làm ơn giải giúp mình nhé ! Mình học hơi kém môn Hình học nên có cả hưỡng dẫn cũng chẳng an thua j!
Mọi người làm ơn giải giúp mình nhé ! Mình học hơi kém môn Hình học nên có cả hưỡng dẫn cũng chẳng an thua j!