16:
a: ĐKXĐ: x>0
\(x+\dfrac{1}{x}+\sqrt{x}+\dfrac{1}{\sqrt{x}}=4\)
\(x+\dfrac{1}{x}>=2\cdot\sqrt{x\cdot\dfrac{1}{x}}=2\)
\(\sqrt{x}+\dfrac{1}{\sqrt{x}}>=2\cdot\sqrt{\sqrt{x}\cdot\dfrac{1}{\sqrt{x}}}=2\)
Do đó: \(x+\dfrac{1}{x}+\sqrt{x}+\dfrac{1}{\sqrt{x}}>=2+2=4\)
Dấu '=' xảy ra khi \(\left\{{}\begin{matrix}x^2=1\\x=1\end{matrix}\right.\)
=>x=1
Đúng 1
Bình luận (0)




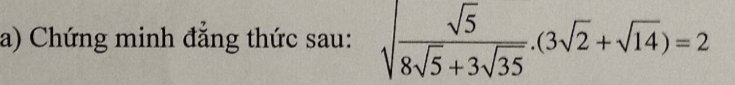


 giúp e bài 3b và bài 6 vs ạ
giúp e bài 3b và bài 6 vs ạ