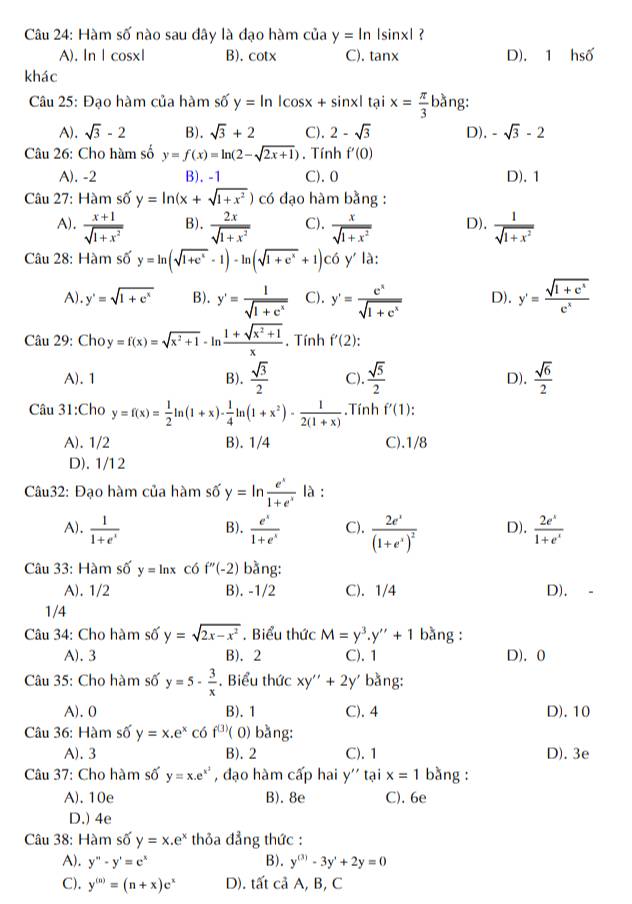
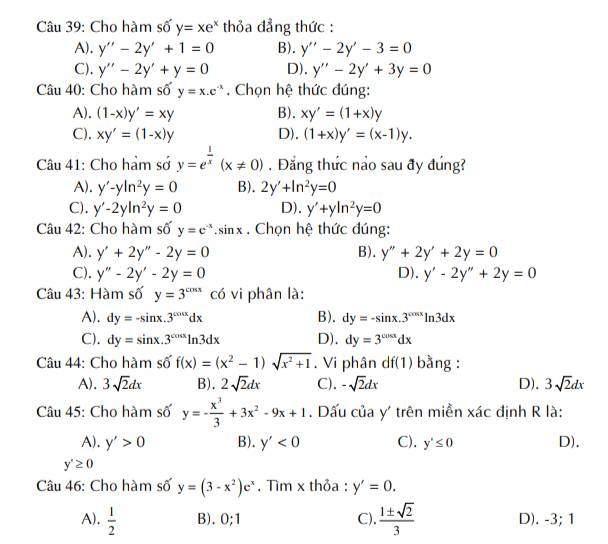24.
\(y'=\dfrac{\left(sinx\right)'}{sinx}=\dfrac{cosx}{sinx}=cotx\)
25.
\(y'=\dfrac{\left(cosx+sinx\right)'}{cosx+sinx}=\dfrac{cosx-sinx}{cosx+sinx}\)
\(y'\left(\dfrac{\pi}{3}\right)=\dfrac{cos\left(\dfrac{\pi}{3}\right)-sin\left(\dfrac{\pi}{3}\right)}{cos\left(\dfrac{\pi}{3}\right)+sin\left(\dfrac{\pi}{3}\right)}=\sqrt{3}-2\)
26.
\(f'\left(x\right)=\dfrac{\left(2-\sqrt{2x+1}\right)'}{2-\sqrt{2x+1}}=\dfrac{-\dfrac{1}{\sqrt{2x+1}}}{2-\sqrt{2x+1}}\)
\(f'\left(0\right)=\dfrac{-\dfrac{1}{\sqrt{2.0+1}}}{2-\sqrt{2.0+1}}=-1\)
27.
\(y'=\dfrac{\left(x+\sqrt{1+x^2}\right)'}{x+\sqrt{1+x^2}}=\dfrac{1+\dfrac{x}{\sqrt{1+x^2}}}{x+\sqrt{1+x^2}}=\dfrac{1}{\sqrt{1+x^2}}\)
28.
\(y'=\dfrac{\left(\sqrt{1+e^x}-1\right)'}{\sqrt{1+e^x-1}}-\dfrac{\left(\sqrt{1+e^x}+1\right)'}{\sqrt{1+e^x}+1}\)
\(=\dfrac{\dfrac{e^x}{2\sqrt{1+e^x}}}{\sqrt{1+e^x}-1}-\dfrac{\dfrac{e^x}{2\sqrt{1+e^x}}}{\sqrt{1+e^x}+1}=\dfrac{e^x}{2\sqrt{1+e^x}}\left(\dfrac{1}{\sqrt{1+e^x}-1}-\dfrac{1}{\sqrt{1+e^x}+1}\right)\)
\(=\dfrac{e^x}{2\sqrt{1+e^x}}.\dfrac{2}{e^x}=\dfrac{1}{\sqrt{1+e^x}}\)
29.
\(f\left(x\right)=\sqrt{x^2+1}-ln\left(1+\sqrt{x^2+1}\right)+lnx\)
\(f'\left(x\right)=\dfrac{x}{\sqrt{x^2+1}}-\dfrac{\left(1+\sqrt{x^2+1}\right)'}{1+\sqrt{x^2+1}}+\dfrac{1}{x}\)
\(=\dfrac{x}{\sqrt{x^2+1}}-\dfrac{\dfrac{x}{\sqrt{x^2+1}}}{1+\sqrt{x^2+1}}+\dfrac{1}{x}\)
\(=\dfrac{x}{\sqrt{x^2+1}}\left(1-\dfrac{1}{1+\sqrt{x^2+1}}\right)+\dfrac{1}{x}\)
\(=\dfrac{x}{1+\sqrt{x^2+1}}+\dfrac{1}{x}\)
\(f'\left(2\right)=\dfrac{2}{1+\sqrt{5}}+\dfrac{1}{2}=\dfrac{\sqrt{5}}{2}\)
31.
\(f'\left(x\right)=\dfrac{1}{2\left(x+1\right)}-\dfrac{1}{4}.\dfrac{2x}{\left(1+x^2\right)}+\dfrac{1}{2\left(x+1\right)^2}\)
\(f'\left(1\right)=\dfrac{1}{4}-\dfrac{1}{4}.\dfrac{2}{2}+\dfrac{1}{8}=\dfrac{1}{8}\)
32.
\(y=ln\left(e^x\right)-ln\left(1+e^x\right)=1-ln\left(1+e^x\right)\)
\(y'=1-\dfrac{e^x}{1+e^x}=\dfrac{1}{1+e^x}\)
33.
\(y'=\dfrac{1}{x}\)
\(y''=-\dfrac{1}{x^2}\)
\(y''\left(-2\right)=-\dfrac{1}{4}\)
34.
\(y'=\dfrac{2-2x}{2\sqrt{2x-x^2}}=\dfrac{1-x}{\sqrt{2x-x^2}}\)
\(y''=\dfrac{-\sqrt{2x-x^2}-\left(1-x\right).\dfrac{1-x}{\sqrt{2x-x^2}}}{2x-x^2}=\dfrac{-1}{\left(\sqrt{2x-x^2}\right)^3}\)
\(\Rightarrow y^3.y''+1=\left(\sqrt{2x-x^2}\right)^3.\dfrac{-1}{\left(\sqrt{2x-x^2}\right)^3}+1=0\)
35.
\(y'=\dfrac{3}{x^2}\)
\(y''=-\dfrac{6}{x^3}\)
\(x.y''+2y'=-\dfrac{6}{x^2}+\dfrac{6}{x^2}=0\)
36.
\(y'=e^x+x.e^x\)
\(y''=e^x+e^x+x.e^x=2e^x+x.e^x\)
\(y^{\left(3\right)}=3e^x+x.e^x\)
\(\Rightarrow f^{\left(3\right)}\left(0\right)=3.e^0+0.e^0=3\)
37.
\(y'=e^{x^2}+2x^2.e^{x^2}\)
\(y''=2x.e^{x^2}+4x.e^{x^2}+4x^3.e^{x^2}\)
\(\Rightarrow y''\left(1\right)=2e+4e+4e=10e\)
38.
\(y'=e^x+x.e^x\)
\(y''=e^x+e^x+x.e^x=2e^x+x.e^x\)
Từ quy luật \(\Rightarrow y^{\left(n\right)}=n.e^x+x.e^x=\left(n+x\right)e^x\)
\(y''-y'=\left(2e^x+x.e^x\right)-\left(e^x+x.e^x\right)=e^x\)
D đúng
39.
Từ câu 38 \(\Rightarrow y''-2y'=-x.e^x\)
\(\Rightarrow y''-2y'+x.e^x=0\)
\(\Rightarrow y''-2y'+y=0\)
40.
\(y'=e^x+x.e^x=\left(1+x\right)e^x\)
\(\Rightarrow xy'=\left(1+x\right)x.e^x=\left(1+x\right).y\)
41.
\(y'=-\dfrac{1}{x^2}.e^{\dfrac{1}{x}}\)
\(ln^2y=\left(\dfrac{1}{x}\right)^2=\dfrac{1}{x^2}\)
\(\Rightarrow y.ln^2y=\dfrac{1}{x^2}.e^{\dfrac{1}{x}}\)
\(\Rightarrow y'+y.ln^2y=0\)
42.
\(y'=-e^{-x}.sinx+e^{-x}.cosx=e^{-x}\left(cosx-sinx\right)\)
\(y''=-e^{-x}\left(cosx-sinx\right)-e^{-x}.\left(cosx+sinx\right)=-2cosx.e^{-x}\)
\(\Rightarrow y''+2y'=-2e^{-x}.sinx\)
\(\Rightarrow y''+2y'+2y=0\)
43.
\(dy=y'dx=-sinx.3^{cosx}.ln3dx\)
44.
\(y'=2x\sqrt{x^2+1}+\dfrac{\left(x^2-1\right)x}{\sqrt{x^2+1}}\)
\(\Rightarrow y'\left(1\right)=2\sqrt{2}\)
\(\Rightarrow df\left(1\right)=2\sqrt{2}dx\)
45.
\(y'=-x^2+6x-9=-\left(x-3\right)^2\le0;\forall x\)
46.
\(y'=-2x.e^x+\left(3-x^2\right).e^x=e^x\left(3-2x-x^2\right)\)
\(y'=0\Rightarrow e^x\left(3-2x-x^2\right)=0\)
\(\Rightarrow3-2x-x^2=0\)
\(\Rightarrow x=\left\{-3;1\right\}\)