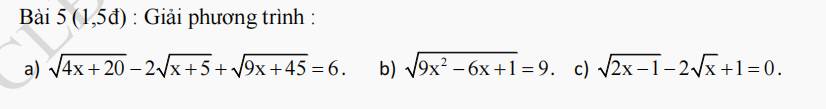a) \(\sqrt{4x+20}-2\sqrt{x+5}+\sqrt{9x+45}=6\) (ĐK: \(x\ge-5\))
\(\Leftrightarrow2\sqrt{x+5}-2\sqrt{x+5}+3\sqrt{x+5}=6\)
\(\Leftrightarrow3\sqrt{x+5}=6\)
\(\Leftrightarrow\sqrt{x+5}=\dfrac{6}{3}\)
\(\Leftrightarrow\sqrt{x+5}=2\)
\(\Leftrightarrow x+5=2^2\)
\(\Leftrightarrow x+5=4\)
\(\Leftrightarrow x=4-5\)
\(\Leftrightarrow x=-1\)
b) \(\sqrt{9x^2-6x+1}=9\)
\(\Leftrightarrow\sqrt{\left(3x-1\right)^2}=9\)
\(\Leftrightarrow\left(3x-1\right)^2=9^2\)
\(\Leftrightarrow\left[{}\begin{matrix}3x-1=9\\3x-1=-9\end{matrix}\right.\)
\(\Leftrightarrow\left[{}\begin{matrix}3x=10\\3x=-8\end{matrix}\right.\)
\(\Leftrightarrow\left[{}\begin{matrix}x=\dfrac{10}{3}\\x=-\dfrac{8}{3}\end{matrix}\right.\)
c) \(\sqrt{2x-1}-2\sqrt{x}+1=0\) (ĐK: \(x\ge\dfrac{1}{2}\))
\(\Leftrightarrow\sqrt{2x-1}=2\sqrt{x}-1\)
\(\Leftrightarrow\left(\sqrt{2x-1}\right)^2=\left(2\sqrt{x}-1\right)^2\)
\(\Leftrightarrow2x-1=4x-4\sqrt{x}+1\)
\(\Leftrightarrow4x-2x-4\sqrt{x}=-1-1\)
\(\Leftrightarrow2x-4\sqrt{x}=-2\)
\(\Leftrightarrow2x-4\sqrt{x}+2=0\)
\(\Leftrightarrow2\left(x-2\sqrt{x}+1\right)=0\)
\(\Leftrightarrow\left(\sqrt{x}-1\right)^2=0\)
\(\Leftrightarrow\sqrt{x}-1=0\)
\(\Leftrightarrow\sqrt{x}=1\)
\(\Leftrightarrow x=1\)
a) ĐK: x>=-5
pt <=> \(\sqrt{4\left(x+5\right)}-2\sqrt{x+5}+\sqrt{9\left(x+5\right)}=6\)
\(\Leftrightarrow2\sqrt{x+5}-2\sqrt{x+5}+3\sqrt{x+5}=6\)
\(\Leftrightarrow3\sqrt{x+5}=6\)
\(\Leftrightarrow\sqrt{x+5}=2\)
\(\Leftrightarrow x+5=4\)
\(\Leftrightarrow x=-1\left(tm\right)\)
Vậy, pt có nghiệm duy nhất là x=-1
b) ĐK: x thuộc R
pt<=> \(\sqrt{\left(3x-1\right)^2}=9\)
\(\Leftrightarrow\left|3x-1\right|=9\Leftrightarrow\left[{}\begin{matrix}3x-1=9\\3x-1=-9\end{matrix}\right.\Leftrightarrow\left[{}\begin{matrix}x=\dfrac{10}{3}\\x=-\dfrac{8}{3}\end{matrix}\right.\)
Vậy, pt có tập nghiệm là \(S=\left\{\dfrac{10}{3};-\dfrac{8}{3}\right\}\)
c) ĐK: \(x\ge\dfrac{1}{2}\)
pt<=> \(\sqrt{2x-1}=2\sqrt{x}-1\)
\(\Leftrightarrow2x-1=4x+1-4\sqrt{x}\)
\(\Leftrightarrow2x+2=4\sqrt{x}\)
\(\Leftrightarrow x+1=2\sqrt{x}\)
\(\Leftrightarrow x-2\sqrt{x}+1=0\Leftrightarrow\left(\sqrt{x}-1\right)^2=0\)
\(\Leftrightarrow\sqrt{x}-1=0\Leftrightarrow x=1\left(tm\right)\)
Vậy, pt có nghiệm duy nhất là x=1