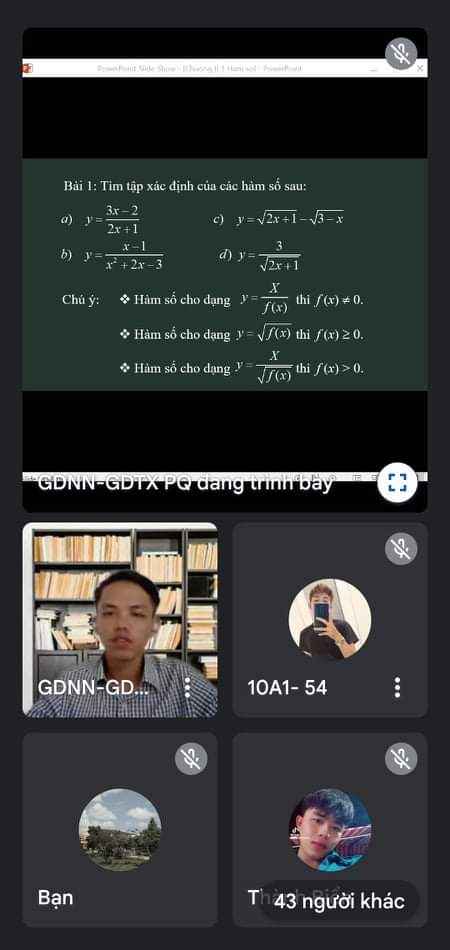
a.TXĐ: \(2x^2-x-3\ne0\Leftrightarrow\left\{{}\begin{matrix}x\ne\frac{3}{2}\\x\ne-1\end{matrix}\right.\)
b. TXĐ: \(\left\{{}\begin{matrix}4x+1\ge0\\-2x+1\ge0\end{matrix}\right.\)\(\Leftrightarrow\frac{1}{4}\le x\le\frac{1}{2}\)
c.\(TXĐ:\left\{{}\begin{matrix}x\ge-2\\\left|x+1\right|-2\ne0\end{matrix}\right.\)\(\Leftrightarrow\left\{{}\begin{matrix}x\ge-2\\x\ne1\end{matrix}\right.\)
sửa lại bài của bạn kia 1 tí :)
a) \(y=\frac{x^2-4}{2x^2-x-3}\)
ĐK: \(2x^2-x-3\ne0\)
\(\Rightarrow x\ne\frac{3}{2};x\ne-1\)
Vậy TXĐ của D là: \(R\backslash\left\{\frac{3}{2}\right\};\left\{-1\right\}\)
b) \(y=\sqrt{4x+1}-\sqrt{-2x+1}\)
ĐK: \(\left\{{}\begin{matrix}4x+1\ge0\\-2x+1\ge0\end{matrix}\right.\Leftrightarrow\left\{{}\begin{matrix}x\ge-\frac{1}{4}\\x\le\frac{1}{2}\end{matrix}\right.\)
Vậy TXĐ của D là: \(\left[-\frac{1}{4};\frac{1}{2}\right]\)
c) \(y=\frac{\sqrt{x+2}}{\left|x+1\right|-2}\)
ĐK: \(\left\{{}\begin{matrix}x+2\ge0\\\left|x+1\right|-2\ne0\left(1\right)\end{matrix}\right.\)
Đặt |x+1| = t khi đó (1) \(\Leftrightarrow\left|t\right|-2\ne0\Leftrightarrow t\ne\pm2\)
\(\Rightarrow x+1\ne2\Rightarrow x\ne1\)
\(\Rightarrow x+1\ne-2\Rightarrow x\ne-3\)
\(\Leftrightarrow\left\{{}\begin{matrix}x\ge-2\\x\ne1\\x\ne-3\end{matrix}\right.\)
Vậy TXĐ của D là: \([-2;+\infty)\backslash\left\{1\right\}\)
Bổ sung câu c:
=> \(\left\{{}\begin{matrix}x\ge2\\x\ne1\\x\ne-3\end{matrix}\right.\)