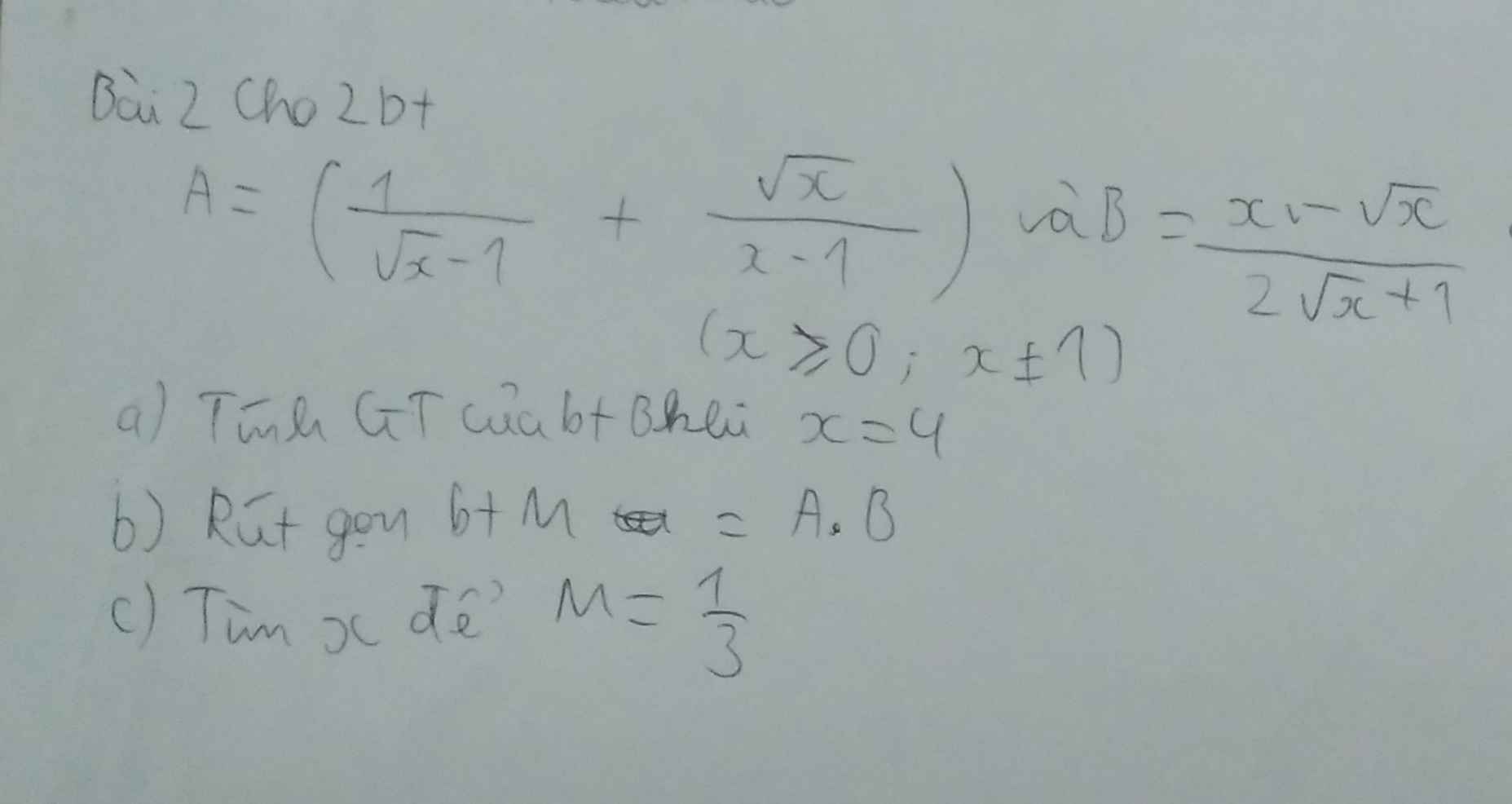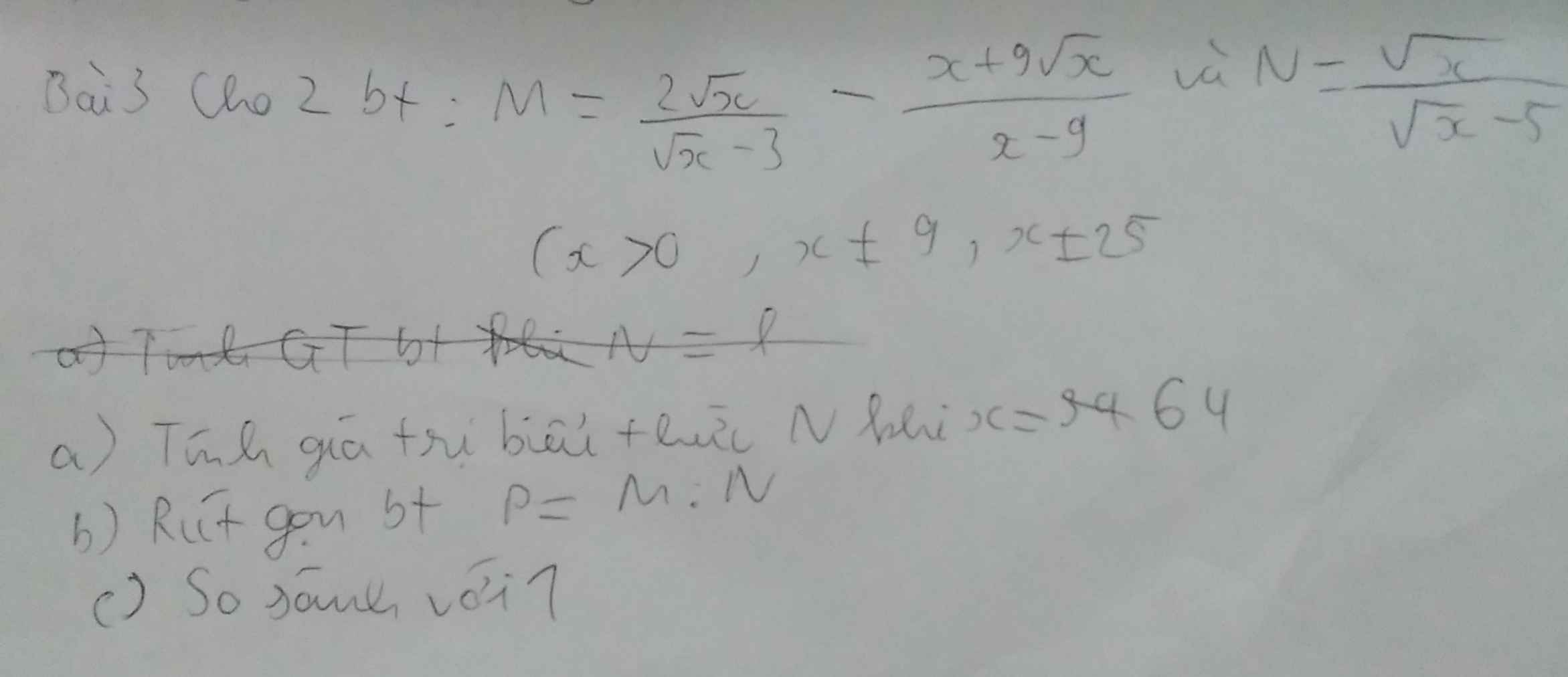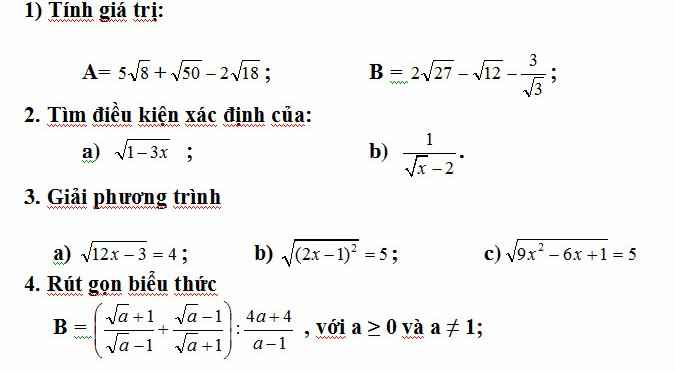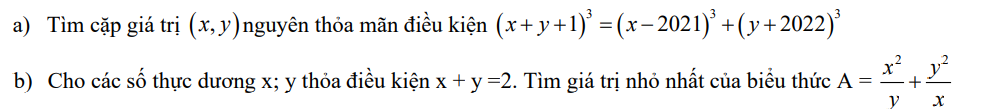Bài 2:
a: \(P=\sqrt{a}+2+\sqrt{a}+2=2\sqrt{a}+4\)
b: Để P=a+1 thì \(a-2\sqrt{a}-3=0\)
hay a=9
\(2,\\ a,P=\dfrac{\left(\sqrt{a}+2\right)^2}{\sqrt{a}+2}+\dfrac{\left(2-\sqrt{a}\right)\left(2+\sqrt{a}\right)}{2-\sqrt{a}}=\sqrt{a}+2+2+\sqrt{a}=2\sqrt{a}+4\\ b,P=a+1\Leftrightarrow a-2\sqrt{a}-3=0\\ \Leftrightarrow\left(\sqrt{a}+1\right)\left(\sqrt{a}-3\right)=0\\ \Leftrightarrow\sqrt{a}=3\left(\sqrt{a}+1>0\right)\\ \Leftrightarrow a=9\left(tm\right)\\ 3,\\ a,ĐK:x\ge0;x\ne4\\ b,P=\dfrac{x+3\sqrt{x}+2+2x-4\sqrt{x}-2-5\sqrt{x}}{\left(\sqrt{x}-2\right)\left(\sqrt{x}+2\right)}\\ P=\dfrac{3x-6\sqrt{x}}{\left(\sqrt{x}-2\right)\left(\sqrt{x}+2\right)}=\dfrac{3\sqrt{x}\left(\sqrt{x}-2\right)}{\left(\sqrt{x}-2\right)\left(\sqrt{x}+2\right)}=\dfrac{2\sqrt{x}}{\sqrt{x}+2}\\ c,P=2\Leftrightarrow2\sqrt{x}+4=2\sqrt{x}\\ \Leftrightarrow0\sqrt{x}=-4\Leftrightarrow x\in\varnothing\)




 mọi người giúp em với ạ 🥺
mọi người giúp em với ạ 🥺
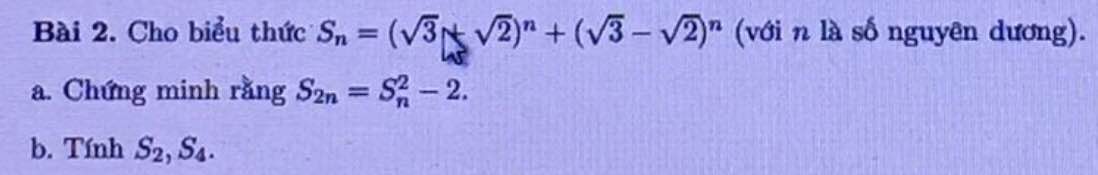 mọi người giải giúp em bài này với ạ 🥺
mọi người giải giúp em bài này với ạ 🥺