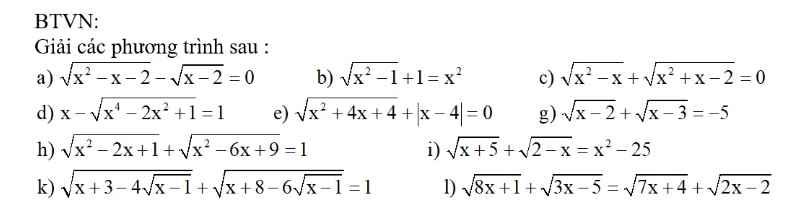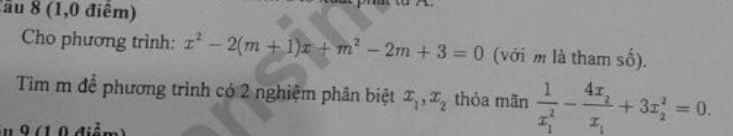Question

mọi người giúp em với ạ, em xin cảm ơn

Answer 1

a: \(\Delta=\left(2m+1\right)^2-4\left(2m+10\right)\)

\(=4m^2+4m+1-8m-40\)

\(=4m^2-4m-39\)

\(=\left(2m-1\right)^2-40\)

Để phương trình có hai nghiệm phân biệt thì:

\(\left[{}\begin{matrix}2m-1>2\sqrt{10}\\2m-1< -2\sqrt{10}\end{matrix}\right.\Leftrightarrow\left[{}\begin{matrix}m>\dfrac{2\sqrt{10}+1}{2}\\m< \dfrac{-2\sqrt{10}+1}{2}\end{matrix}\right.\)

b: \(A=10x_1x_2+\left(x_1+x_2\right)^2-2x_1x_2\)

\(=\left(x_1+x_2\right)^2+8x_1x_2\)

\(=\left(2m+1\right)^2+8\left(2m+10\right)\)

\(=4m^2+4m+1+16m+80\)

\(=4m^2+20m+81=4m^2+20m+25+56\)

\(=\left(2m+5\right)^2+56\)>=56

Dấu '=' xảy ra khi m=-5/2