\(1,\\ a,=5\sqrt{2}-12\sqrt{2}+10\sqrt{2}=3\sqrt{2}\\ b,=\dfrac{\sqrt{5}-2}{5-4}-\dfrac{\sqrt{5}\left(\sqrt{3}-2\right)}{\sqrt{3}-2}=\sqrt{5}-2-\sqrt{5}=-2\\ c,=3\sqrt{3}-\left|1-\sqrt{3}\right|-\dfrac{6\sqrt{3}}{3}=3\sqrt{3}-\left(\sqrt{3}-1\right)-2\sqrt{3}=1\)
\(2,\\ a,P=\left(\dfrac{1}{\sqrt{a}-2}+\dfrac{1}{\sqrt{a}+2}\right)\left(1+\dfrac{2\left(\sqrt{a}-2\right)}{a-2\sqrt{a}}\right)\left(a>0;a\ne4\right)\\ P=\dfrac{\sqrt{a}+2+\sqrt{a}-2}{\left(\sqrt{a}-2\right)\left(\sqrt{a}+2\right)}\cdot\left[1+\dfrac{2\left(\sqrt{a}-2\right)}{\sqrt{a}\left(\sqrt{a}-2\right)}\right]\\ P=\dfrac{2\sqrt{a}}{\left(\sqrt{a}-2\right)\left(\sqrt{a}+2\right)}\cdot\dfrac{\sqrt{a}+2}{\sqrt{a}}=\dfrac{2}{\sqrt{a}-2}\)
\(b,\sqrt{4x+12}-3\sqrt{x+3}+\sqrt{16x+48}=9\left(x\ge-3\right)\\ \Leftrightarrow2\sqrt{x+3}-3\sqrt{x+3}+4\sqrt{x+3}=9\\ \Leftrightarrow3\sqrt{x+3}=9\Leftrightarrow\sqrt{x+3}=3\\ \Leftrightarrow x+3=9\Leftrightarrow x=6\left(tm\right)\)









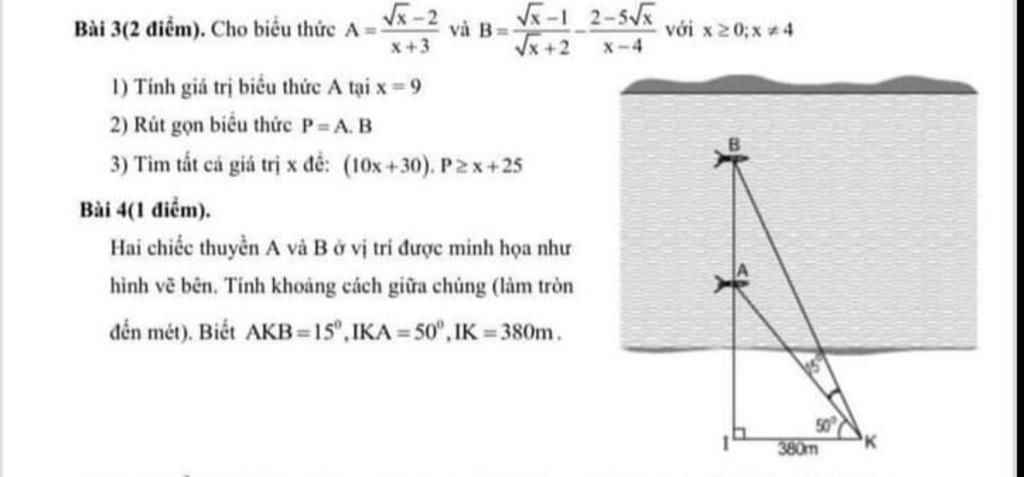
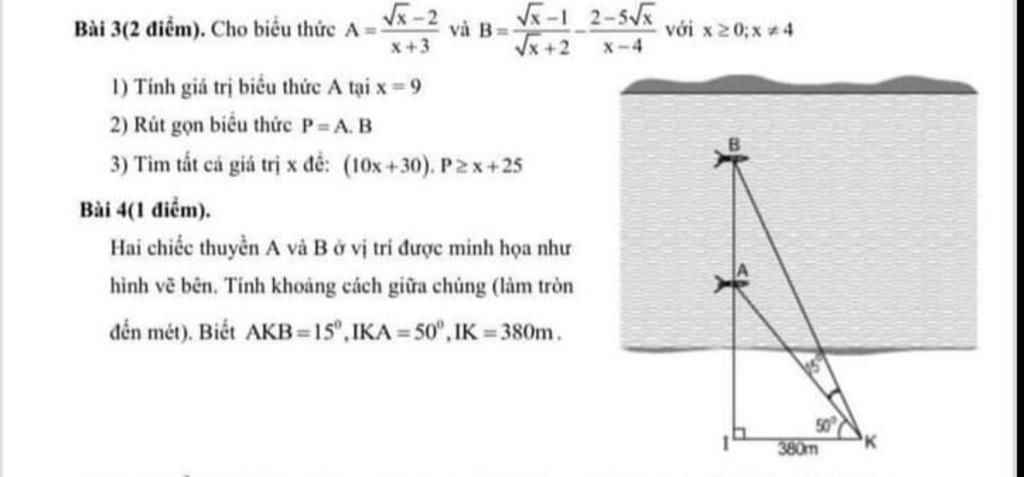
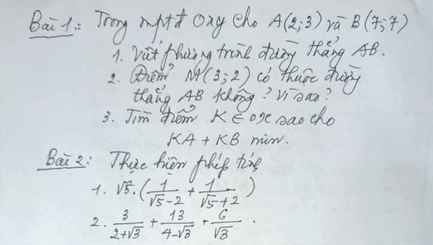 làm hộ mik bài 1 thôi nha
làm hộ mik bài 1 thôi nha