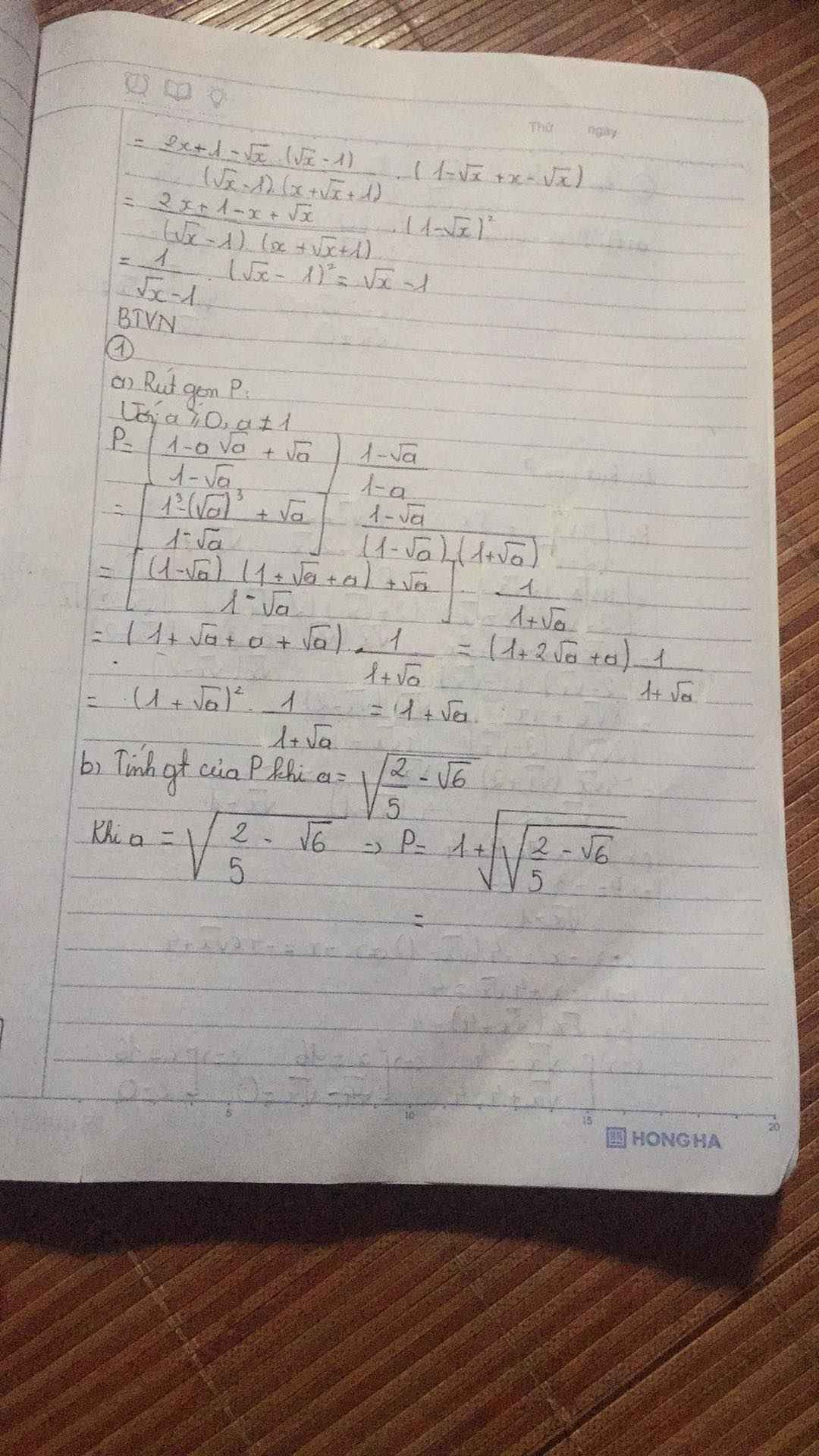bạn đăng ít thôi cho mn cùng giúp nhé
a, \(x^2-2\sqrt{5}x+5=0\Leftrightarrow\left(x-\sqrt{5}\right)^2=0\Leftrightarrow x=\sqrt{5}\)
b, \(x^2-9x+10=0\)
\(\Delta=81-4.10=41>0\)
\(x_1=\dfrac{9-\sqrt{41}}{2};x_2=\dfrac{9+\sqrt{41}}{2}\)
c, \(2x^2-3x+5=0\)
\(\Delta=9-4.5.2=9-40< 0\)
Vậy pt vô nghiệm
d: \(\text{Δ}=\left(-6\right)^2-4\cdot1\cdot14=36-56=-20< 0\)
Do đó: Phương trình vô nghiệm
e: =>x+9=16
hay x=7
f: =>(x-3)(x-5)=0
=>x=3 hoặc x=5
j: \(\Leftrightarrow\left(4x-5\right)^2=0\)
=>4x-5=0
hay x=5/4
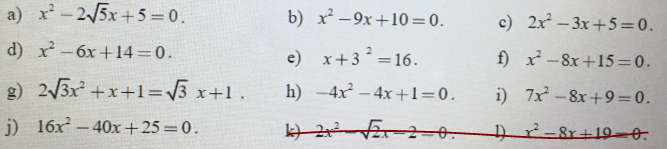 GIÚP MÌNH CÂU KHÔNG GẠCH ĐỎ VỚI NHÉ!
GIÚP MÌNH CÂU KHÔNG GẠCH ĐỎ VỚI NHÉ!


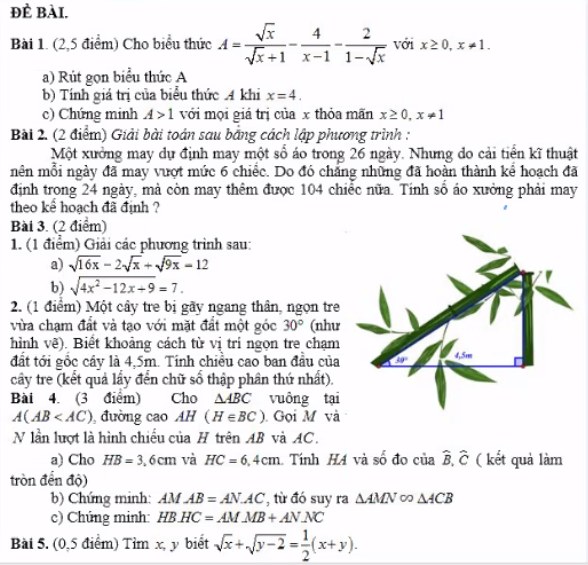



 Giúp mình câu Viest với, mình phân tích không ra, mình đang cần gấp.Cảm ơn rất nhiều!
Giúp mình câu Viest với, mình phân tích không ra, mình đang cần gấp.Cảm ơn rất nhiều!