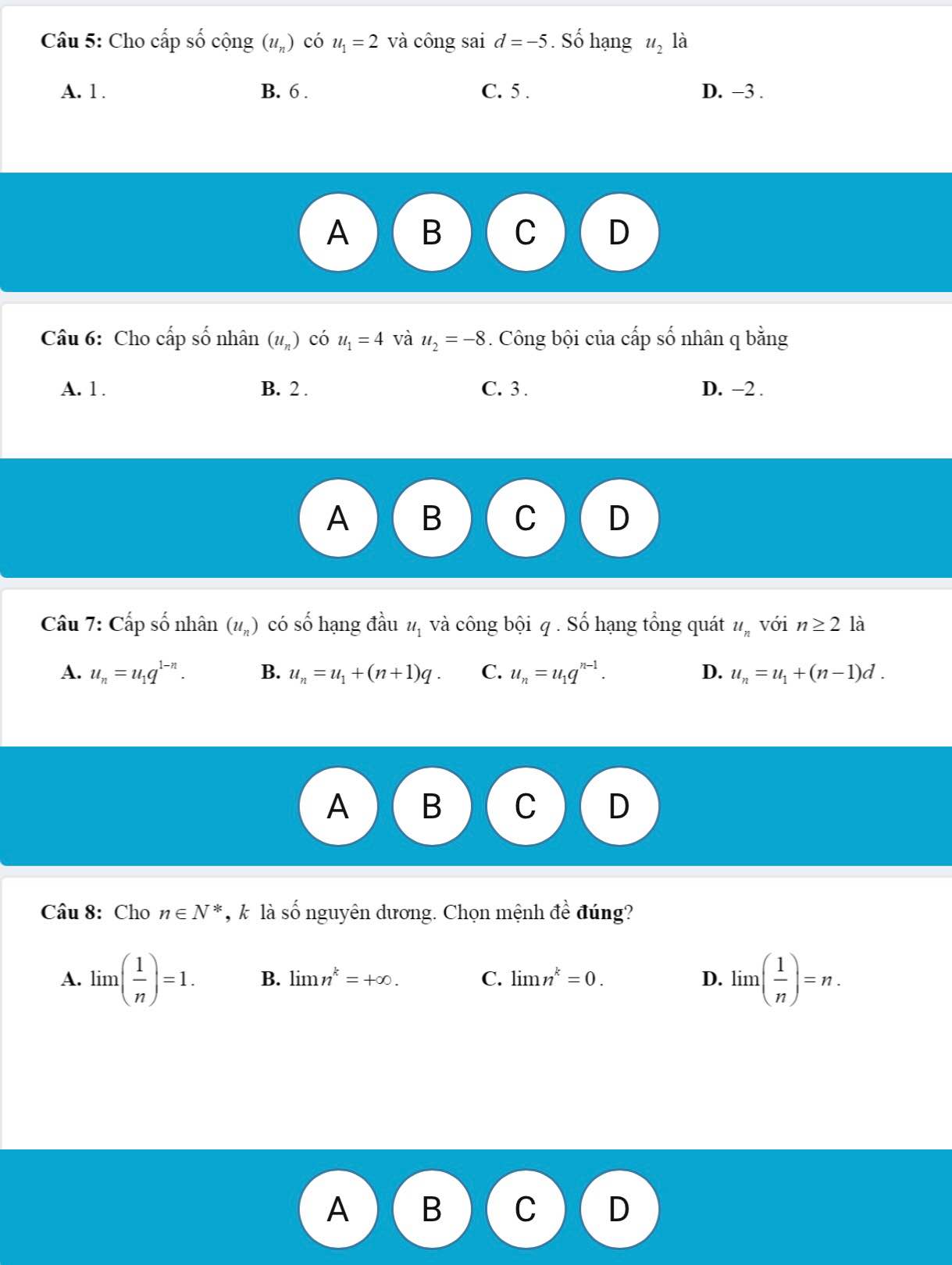
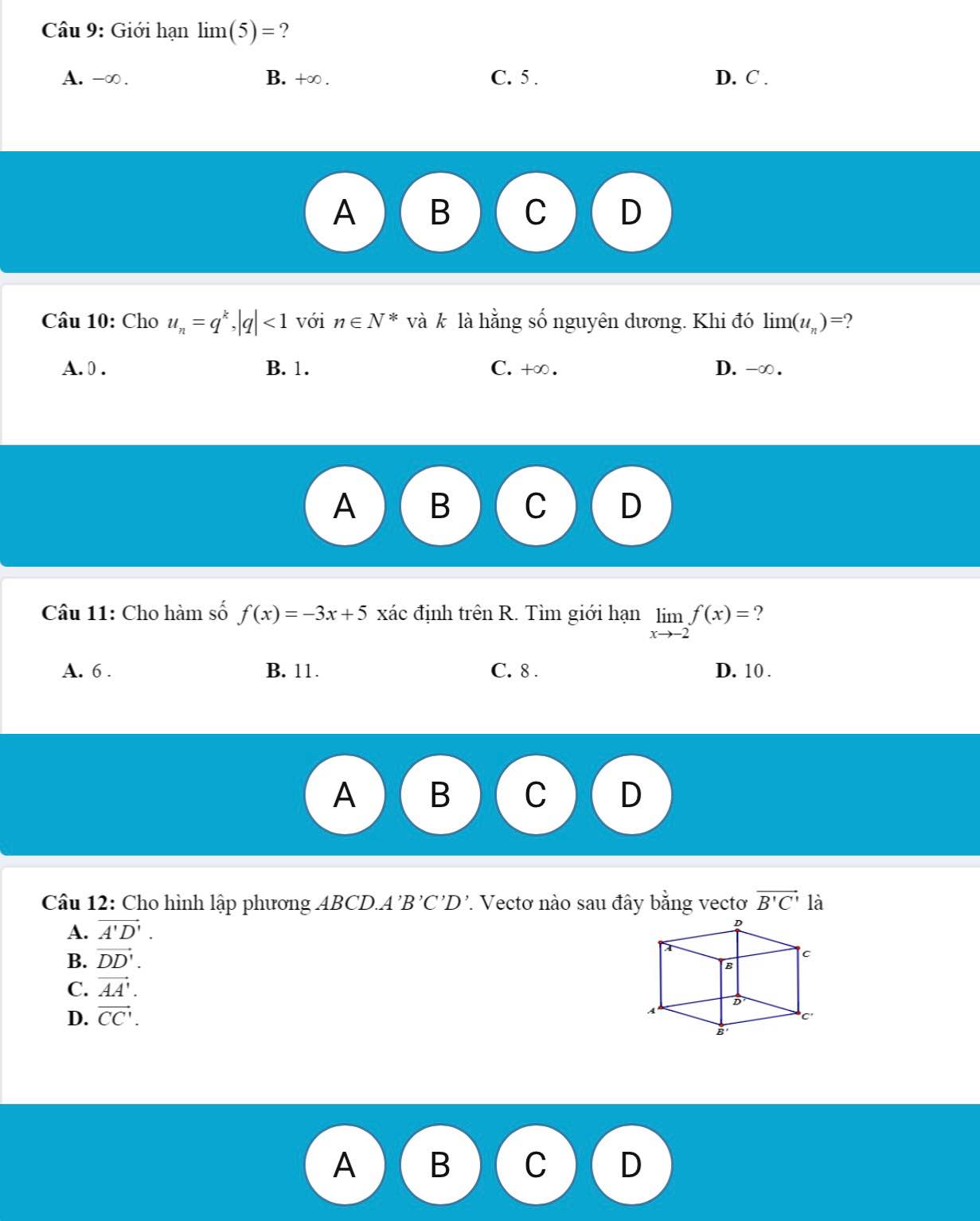
114.
ĐKXĐ: \(\left\{{}\begin{matrix}x+2>0\\8-log_2\left(x+2\right)\ge0\end{matrix}\right.\) \(\Rightarrow-2< x\le254\)
\(\left(x^2-6x+5\right)\sqrt{8-log_2\left(x+2\right)}\ge0\)
\(\Rightarrow\left[{}\begin{matrix}8-log_2\left(x+2\right)=0\\x^2-6x+5\ge0\end{matrix}\right.\)
\(\Rightarrow\left[{}\begin{matrix}x=254\\x\ge5\\x\le1\end{matrix}\right.\) \(\Rightarrow\left[{}\begin{matrix}-2< x\le1\\5\le x\le254\end{matrix}\right.\)
\(\Rightarrow\) Có 253 số nguyên
115.
\(\left(log_4y-1\right)\left(x.log_2y-8\right)< 0\)
\(\Leftrightarrow\dfrac{x}{2}\left(log_2y-2\right)\left(log_2y-\dfrac{8}{x}\right)< 0\)
\(\Leftrightarrow\left(log_2y-2\right)\left(log_2y-\dfrac{8}{x}\right)< 0\)
Th1: \(\dfrac{8}{x}>2\Rightarrow x< 4\)
\(\Rightarrow2< log_2y< \dfrac{8}{x}\Rightarrow4< y< 2^{\dfrac{8}{x}}\)
Do có đúng 2 số nguyên y thỏa mãn \(\Rightarrow6< 2^{\dfrac{8}{x}}< 7\Rightarrow log_26< \dfrac{8}{x}< log_27\Rightarrow x=3\)
TH2: \(\dfrac{8}{x}< 2\Rightarrow x>4\)
\(\Rightarrow\dfrac{8}{x}< log_2y< 2\Rightarrow2^{\dfrac{8}{x}}< y< 4\)
Có đúng 2 giá trị y nguyên \(\Rightarrow1< 2^{\dfrac{8}{x}}< 2\)
\(\Rightarrow0< \dfrac{8}{x}< 1\)
\(\Rightarrow x>8\Rightarrow2022-9+1=2014\) giá trị nguyên x
Tổng 2 trường hợp \(\Rightarrow2015\) x nguyên dương
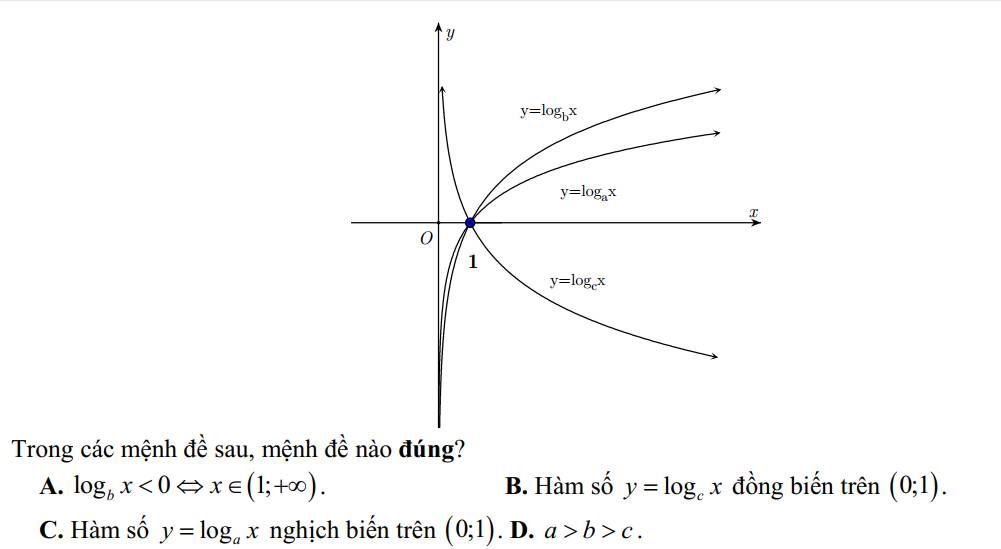
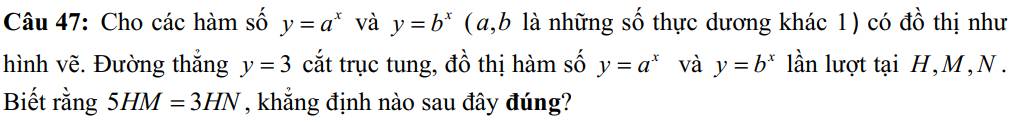
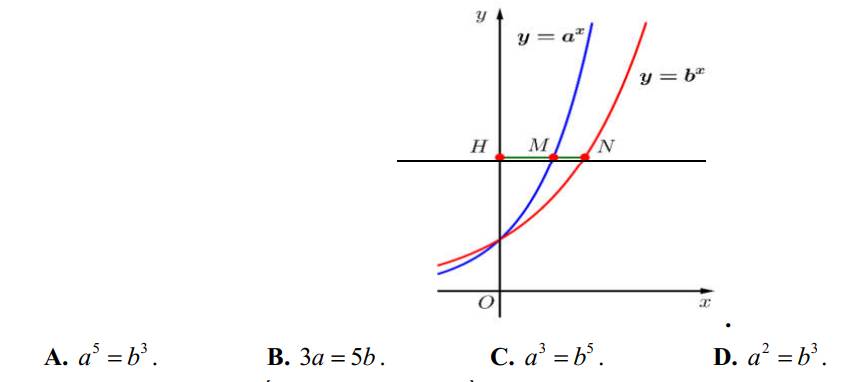
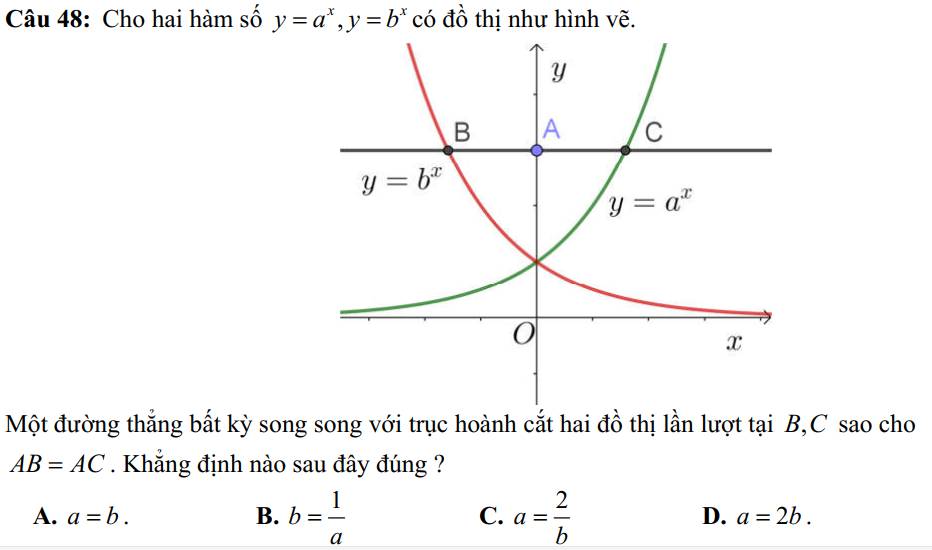
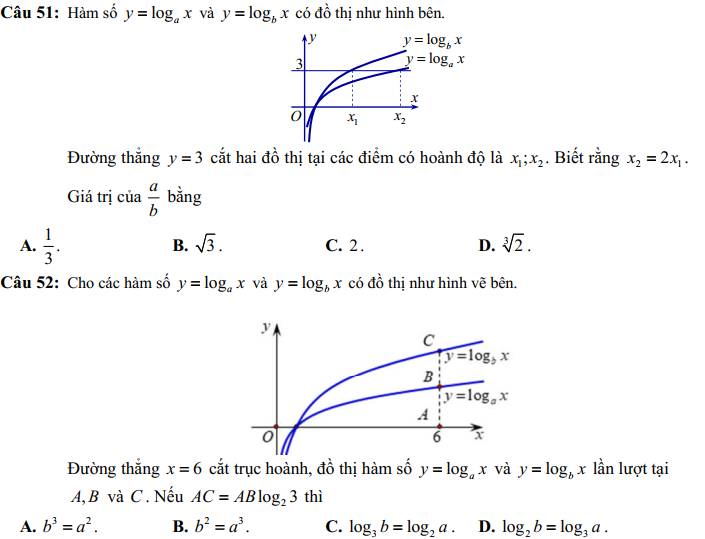
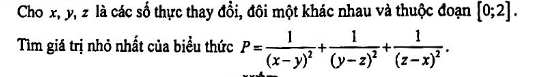
Giúp em với ạ. Cần rất gấp ạ. Em cảm ơn ạ
