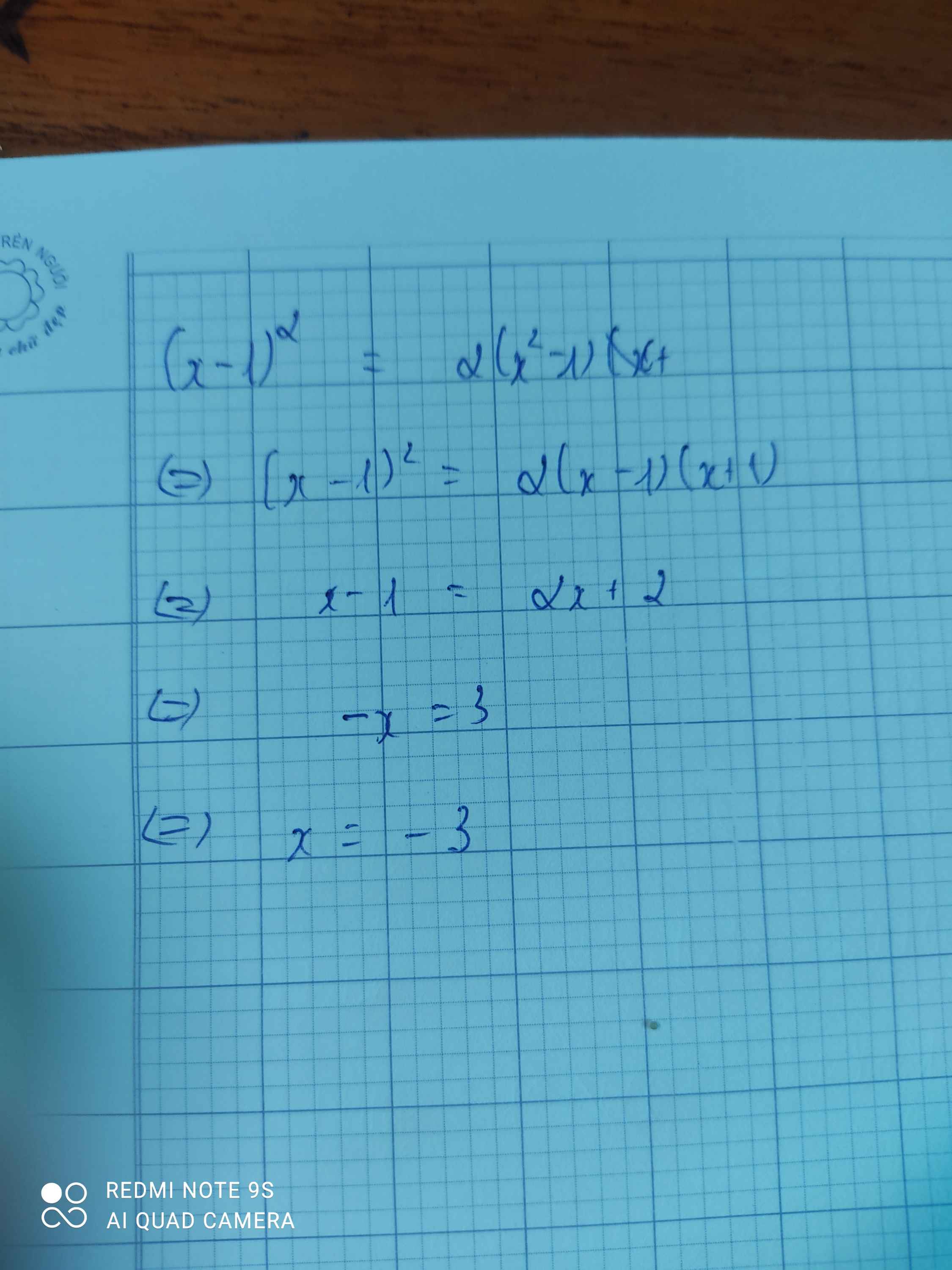\(\left(x-1\right)^2=2.\left(x^2-1\right)\\ \Leftrightarrow x^2-2x+1=2x^2-2\\ \Leftrightarrow2x^2-x^2+2x-2-1=0\\ \Leftrightarrow x^2+2x-3=0\\ \Leftrightarrow x^2+3x-x-3=0\\ \Leftrightarrow x.\left(x+3\right)-\left(x+3\right)=0\\ \Leftrightarrow\left(x-1\right).\left(x+3\right)=0\\ \Leftrightarrow\left[{}\begin{matrix}x-1=0\\x+3=0\end{matrix}\right.\Leftrightarrow\left[{}\begin{matrix}x=1\\x=-3\end{matrix}\right.\\ \Rightarrow S=\left\{-3;1\right\}\)