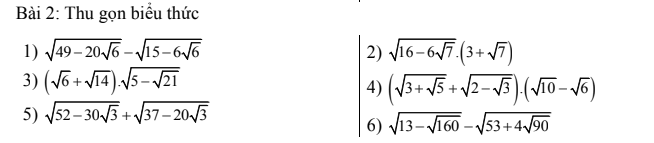`1)`\(K=2\left(\dfrac{1}{\sqrt{a}-1}-\dfrac{1}{\sqrt{a}}\right):\left(\dfrac{\sqrt{a}+1}{a^2-a}\right)\)
\(K=2\left(\dfrac{\sqrt{a}-\sqrt{a}+1}{\sqrt{a}\left(\sqrt{a}-1\right)}\right):\left(\dfrac{\sqrt{a}+1}{a\left(a-1\right)}\right)\)
\(K=\dfrac{2}{\sqrt{a}\left(\sqrt{a}-1\right)}.\dfrac{a\left(\sqrt{a}-1\right)\left(\sqrt{a}+1\right)}{\sqrt{a}+1}\)
\(K=2\sqrt{a}\)
`2)`\(K=\sqrt{2012}\)
\(\Leftrightarrow2\sqrt{a}=\sqrt{2012}\)
\(\Leftrightarrow4a=2012\)
\(\Leftrightarrow a=503\)
\(K=2\left(\dfrac{1}{\sqrt{a}-1}-\dfrac{1}{\sqrt{a}}\right):\dfrac{\sqrt{a}+1}{a^2-a}\\ =2\left(\dfrac{\sqrt{a}}{\sqrt{a}\left(\sqrt{a}-1\right)}-\dfrac{\sqrt{a}-1}{\sqrt{a}\left(\sqrt{a}-1\right)}\right).\dfrac{a\left(a-1\right)}{\sqrt{a}+1}\\ =2.\dfrac{\sqrt{a}-\sqrt{a}+1}{\sqrt{a}\left(\sqrt{a}-1\right)}.\dfrac{\sqrt{a}.\sqrt{a}\left(\sqrt{a}-1\right)\left(\sqrt{a}+1\right)}{\sqrt{a}+1}\\ =2\sqrt{a}\)
`2, K= sqrt2012`
`<=>2sqrt{a}=sqrt2012`
`<=>sqrt4 . sqrt{a}=sqrt2012`
`<=>sqrt{a}=503`
`<=>a=503(tm)`