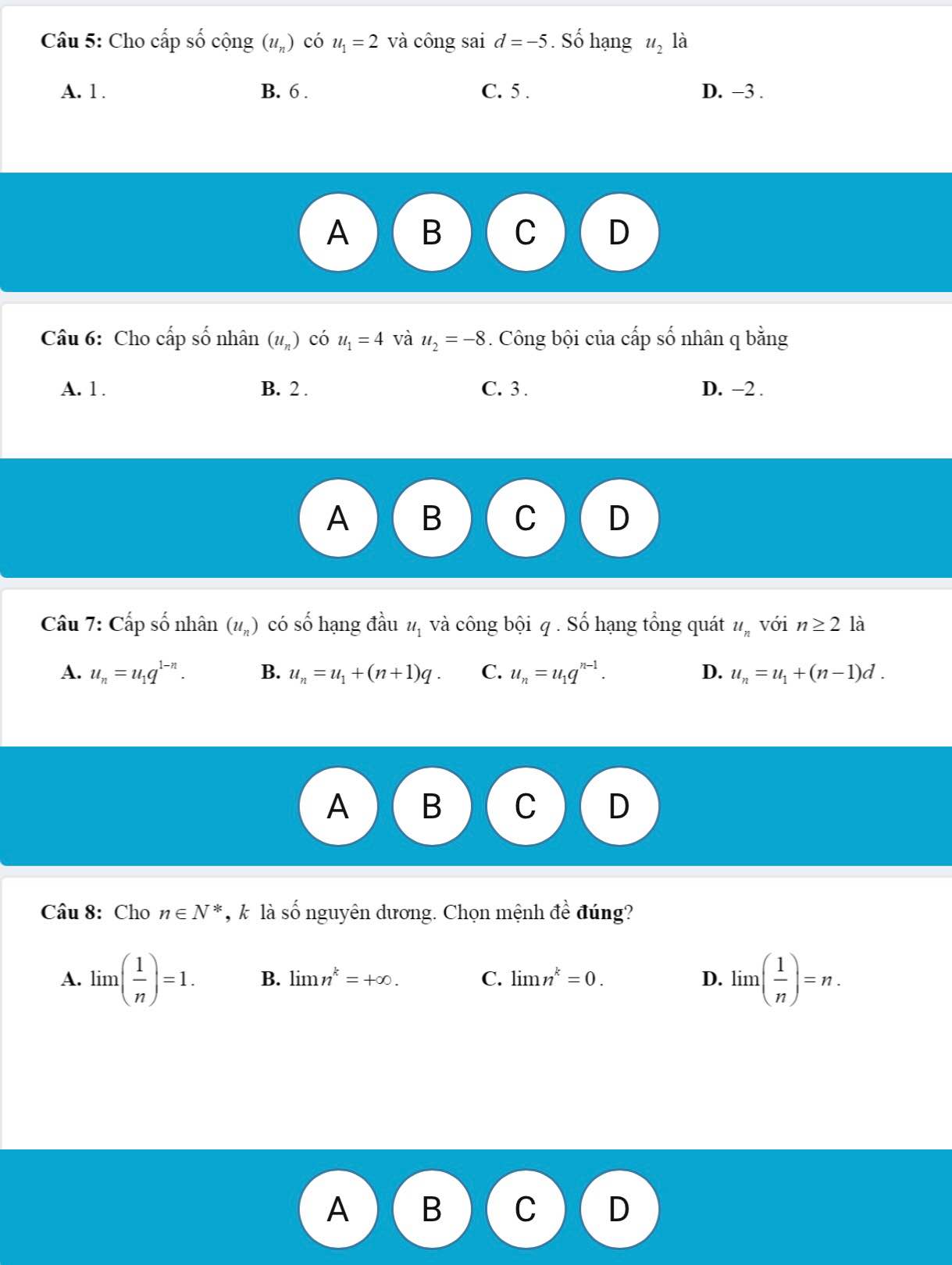
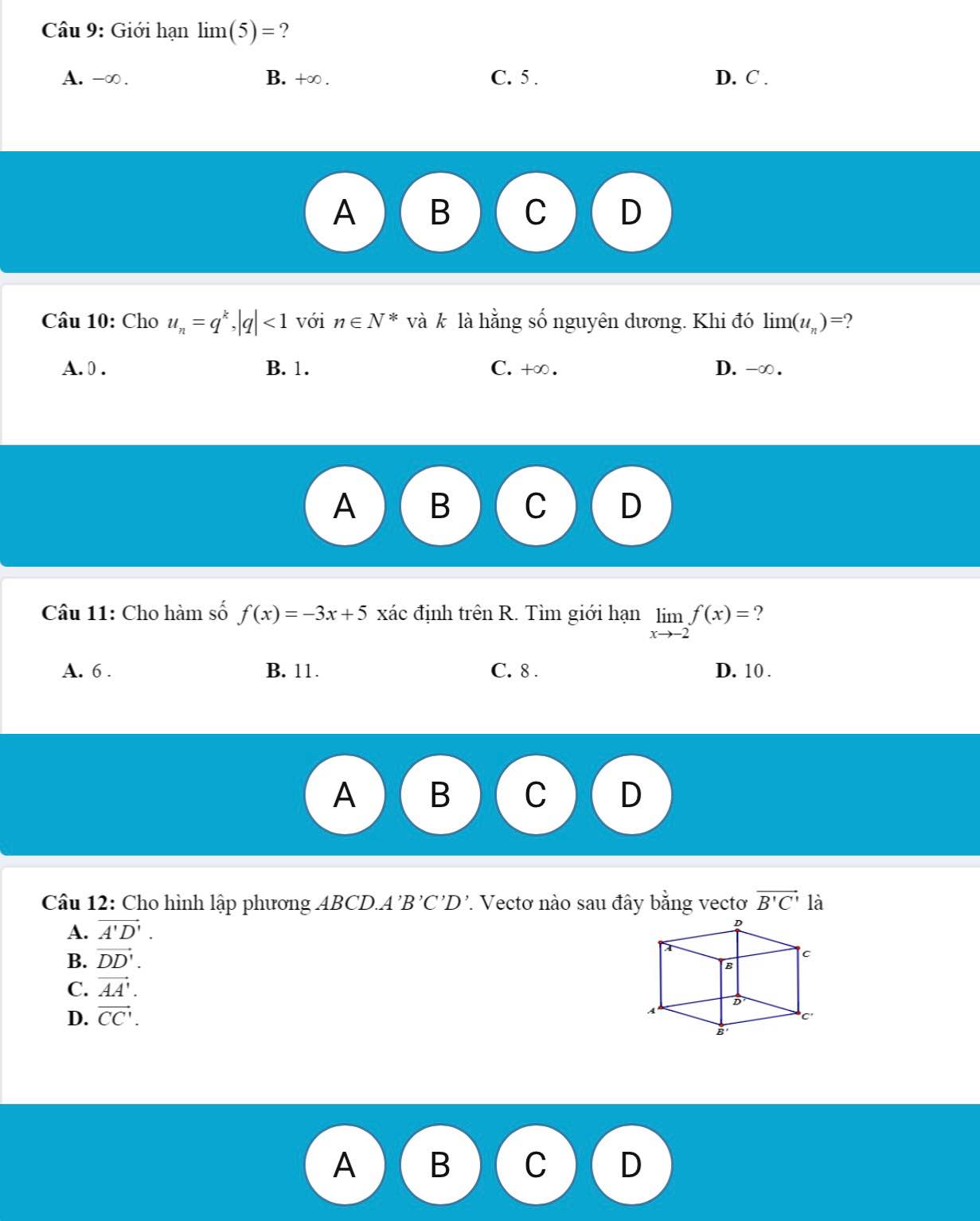
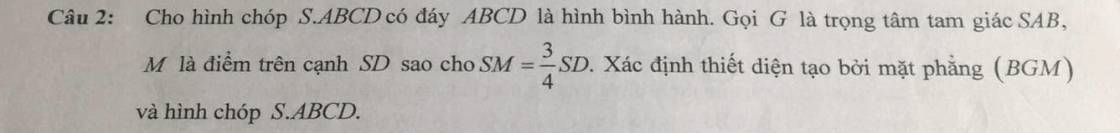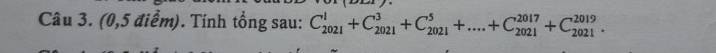Câu 1:
a:
TXĐ: D=R
=>F(x) luôn xác định trên R
Khi x\(\in\)D thì -x\(\in\)D
\(f\left(-x\right)=cos\left(-2x\right)=cos2x=f\left(x\right)\)
=>f(x) là hàm số chẵn
=>Đúng
b: f(x)=cos2x
=>f(x) tuần hoàn theo chu kì là \(T=\dfrac{2\Omega}{2}=\Omega\)
F(x)=cos2x
=>TGT là T=[-1;1]
=>Sai
c: f(x)=0
=>cos2x=0
=>\(2x=\dfrac{\Omega}{2}+k\Omega\)
=>\(x=\dfrac{\Omega}{4}+\dfrac{k\Omega}{2}\)
=>Đúng
d: f(x)=1/2
=>cos2x=1/2
=>\(\left[{}\begin{matrix}2x=\dfrac{\Omega}{3}+k2\Omega\\2x=-\dfrac{\Omega}{3}+k2\Omega\end{matrix}\right.\Leftrightarrow\left[{}\begin{matrix}x=\dfrac{\Omega}{6}+k\Omega\\x=-\dfrac{\Omega}{6}+k\Omega\end{matrix}\right.\)
\(x\in\left[0;\Omega\right]\)
=>\(\left[{}\begin{matrix}\dfrac{\Omega}{6}+k\Omega\in\left[0;\Omega\right]\\-\dfrac{\Omega}{6}+k\Omega\in\left[0;\Omega\right]\end{matrix}\right.\Leftrightarrow\left[{}\begin{matrix}k+\dfrac{1}{6}\in\left[0;1\right]\\k-\dfrac{1}{6}\in\left[0;1\right]\end{matrix}\right.\)
=>\(\left[{}\begin{matrix}k\in\left[-\dfrac{1}{6};\dfrac{5}{6}\right]\\k\in\left[\dfrac{1}{6};\dfrac{7}{6}\right]\end{matrix}\right.\Leftrightarrow\left[{}\begin{matrix}k=0\\k=1\end{matrix}\right.\)
Khi k=0 thì \(x=\dfrac{\Omega}{6}+0\cdot\Omega=\dfrac{\Omega}{6}\)
Khi k=1 thì \(x=-\dfrac{\Omega}{6}+\Omega=\dfrac{5}{6}\Omega\)
=>Tổng các nghiệm là \(\dfrac{\Omega}{6}+\dfrac{5}{6}\Omega=\Omega\)
=>Sai