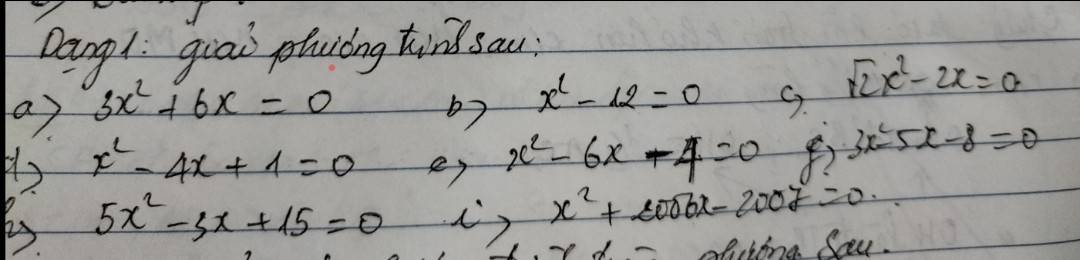a: \(3x^2+6x=0\)
=>\(3x\left(x+2\right)=0\)
=>x(x+2)=0
=>\(\left[{}\begin{matrix}x=0\\x+2=0\end{matrix}\right.\Leftrightarrow\left[{}\begin{matrix}x=0\\x=-2\end{matrix}\right.\)
b: \(x^2-12=0\)
=>\(x^2=12\)
=>\(x=\pm2\sqrt{3}\)
c: \(x^2\cdot\sqrt{2}-2x=0\)
=>\(x^2-x\cdot\sqrt{2}=0\)
=>\(x\left(x-\sqrt{2}\right)=0\)
=>\(\left[{}\begin{matrix}x=0\\x-\sqrt{2}=0\end{matrix}\right.\Leftrightarrow\left[{}\begin{matrix}x=0\\x=\sqrt{2}\end{matrix}\right.\)
d: \(x^2-4x+1=0\)
=>\(x^2-4x+4-3=0\)
=>\(\left(x-2\right)^2=3\)
=>\(\left[{}\begin{matrix}x-2=\sqrt{3}\\x-2=-\sqrt{3}\end{matrix}\right.\Leftrightarrow\left[{}\begin{matrix}x=2+\sqrt{3}\\x=2-\sqrt{3}\end{matrix}\right.\)
e: \(x^2-6x+4=0\)
=>\(x^2-6x+9-5=0\)
=>\(\left(x-3\right)^2=5\)
=>\(\left[{}\begin{matrix}x-3=\sqrt{5}\\x-3=-\sqrt{5}\end{matrix}\right.\Leftrightarrow\left[{}\begin{matrix}x=3+\sqrt{5}\\x=3-\sqrt{5}\end{matrix}\right.\)
g: \(3x^2-5x-8=0\)
=>\(3x^2+3x-8x-8=0\)
=>3x(x+1)-8(x+1)=0
=>(x+1)(3x-8)=0
=>\(\left[{}\begin{matrix}x+1=0\\3x-8=0\end{matrix}\right.\Leftrightarrow\left[{}\begin{matrix}x=-1\\x=\dfrac{8}{3}\end{matrix}\right.\)
h: \(5x^2-3x+15=0\)
\(\text{Δ}=\left(-3\right)^2-4\cdot5\cdot15=9-20\cdot15=9-300=-291< 0\)
=>Phương trình vô nghiệm
i: \(x^2+2006x-2007=0\)
=>\(x^2+2007x-x-2007=0\)
=>x(x+2007)-(x+2007)=0
=>(x+2007)(x-1)=0
=>\(\left[{}\begin{matrix}x+2007=0\\x-1=0\end{matrix}\right.\Leftrightarrow\left[{}\begin{matrix}x=-2007\\x=1\end{matrix}\right.\)