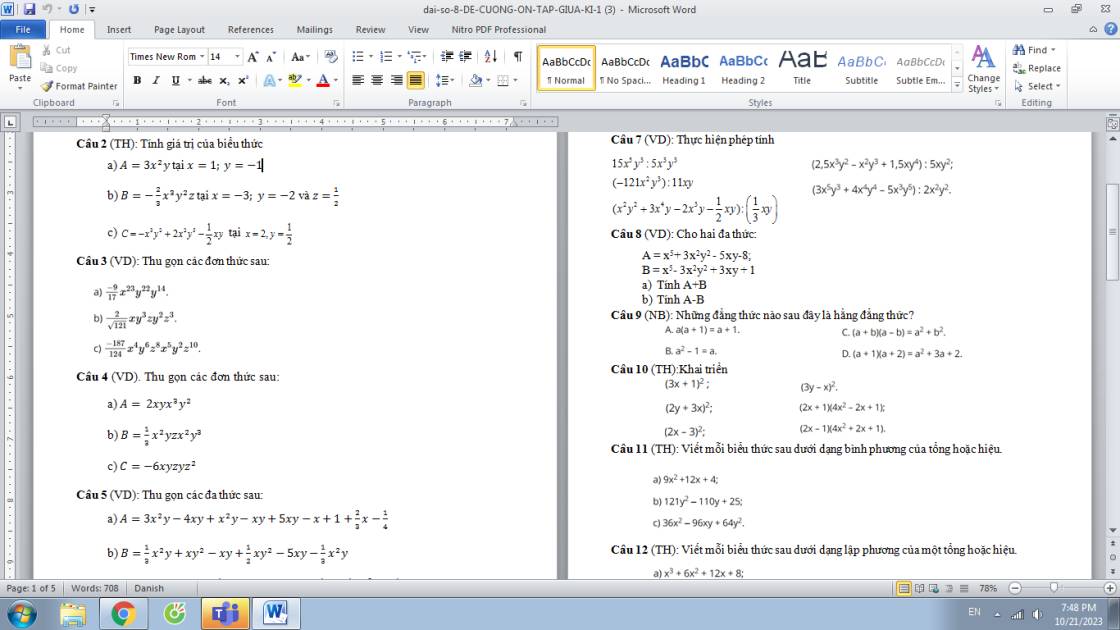
11:
a: \(9x^2+12x+4\)
\(=\left(3x\right)^2+2\cdot3x\cdot2+2^2\)
\(=\left(3x+2\right)^2\)
b: \(121y^2-110y+25\)
\(=\left(11y\right)^2-2\cdot11y\cdot5+5^2\)
\(=\left(11y-5\right)^2\)
c: \(36x^2-96xy+64y^2\)
\(=\left(6x\right)^2-2\cdot6x\cdot8y+\left(8y\right)^2\)
\(=\left(6x-8y\right)^2\)
10:
\(\left(3x+1\right)^2=9x^2+6x+1\)
\(\left(3y-x\right)^2=9y^2-6xy+x^2\)
\(\left(3x+2y\right)^2=\left(3x\right)^2+2\cdot3x\cdot2y+\left(2y\right)^2\)
\(=9x^2+12xy+4y^2\)
\(\left(2x+1\right)\left(4x^2-2x+1\right)=\left(2x\right)^3+1^3=8x^3+1\)
(2x-3)2
\(=\left(2x\right)^2-2\cdot2x\cdot3+3^2\)
\(=4x^2-12x+9\)
\(\left(2x-1\right)\left(4x^2+2x+1\right)\)
\(=\left(2x-1\right)\left[\left(2x\right)^2+2x\cdot1+1^2\right]\)
\(=\left(2x\right)^3-1^3=8x^3-1\)