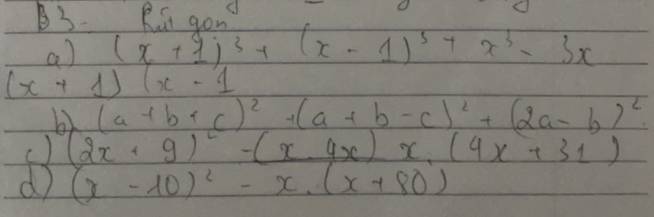a: \(=x^3+3x^2+3x+1+x^3-3x^2+3x-1+x^3-3x\left(x^2-1\right)\)
\(=3x^3+6x-3x^2+3x=9x\)
b: \(=\left(a+b\right)^2+2\left(a+b\right)\cdot c+c^2+\left(a+b\right)^2-2c\left(a+b\right)+c^2+\left(2a-b\right)^2\)
\(=2\left(a+b\right)^2+2c^2+4a^2-4ab+b^2\)
\(=2a^2+4ab+2b^2+2c^2+4a^2-4ab+b^2\)
\(=6a^2+3b^2+2c^2\)
d: \(=x^2-20x+100-x^2-80x=-100x+100\)
Đúng 1
Bình luận (0)