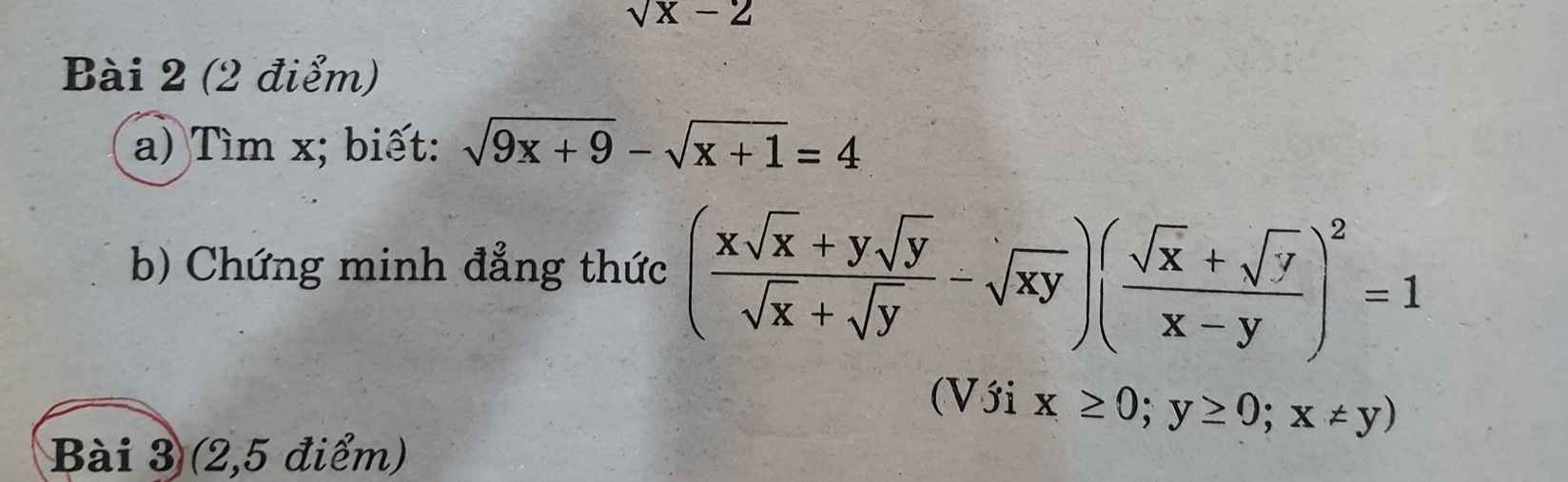Bài 2a: Thấy bạn khoanh 2a nên mình làm 2a thôi nhé.
ĐKXĐ: \(x\ge-1\)
\(\sqrt{9x+9}-\sqrt{x+1}=4\)
\(\Leftrightarrow\sqrt{9\left(x+1\right)}-\sqrt{x+1}=4\)
\(\Leftrightarrow3\sqrt{x+1}-\sqrt{x+1}=4\)
\(\Leftrightarrow2\sqrt{x+1}=4\)
\(\Leftrightarrow4\left(x+1\right)=16\Leftrightarrow x+1=4\Leftrightarrow x=3\) (Tm ĐKXĐ)
Vậy...
b) ĐKXĐ: \(x\ge0;y\ge0;x\ne y\)
Ta có: \(\left(\dfrac{x\sqrt{x}+y\sqrt{y}}{\sqrt{x}+\sqrt{y}}-\sqrt{xy}\right)\left(\dfrac{\sqrt{x}+\sqrt{y}}{x-y}\right)^2\)
\(=\left(\dfrac{\sqrt{x^3}+\sqrt{y^3}}{\sqrt{x}+\sqrt{y}}-\sqrt{xy}\right)\left(\dfrac{\sqrt{x}+\sqrt{y}}{\left(\sqrt{x}+\sqrt{y}\right)\left(\sqrt{x}-\sqrt{y}\right)}\right)^2\)
\(=\left(\dfrac{\left(\sqrt{x}+\sqrt{y}\right)\left(x-\sqrt{xy}+y\right)}{\sqrt{x}+\sqrt{y}}-\sqrt{xy}\right)\left(\dfrac{1}{\sqrt{x}-\sqrt{y}}\right)^2\)
\(=\left(x-\sqrt{xy}+y-\sqrt{xy}\right).\left(\dfrac{1}{\sqrt{x}-\sqrt{y}}\right)^2\)
\(=\left(x-2\sqrt{xy}+y\right).\left(\dfrac{1}{\sqrt{x}-\sqrt{y}}\right)^2\)
\(=\left(\sqrt{x}-\sqrt{y}\right)^2.\left(\dfrac{1}{\sqrt{x}-\sqrt{y}}\right)^2=1\)
=> Đpcm.
a: Ta có: \(\sqrt{9x+9}-\sqrt{x+1}=4\)
\(\Leftrightarrow3\sqrt{x+1}-\sqrt{x+1}=4\)
\(\Leftrightarrow2\sqrt{x+1}=4\)
\(\Leftrightarrow x+1=4\)
hay x=3
b: Ta có: \(\left(\dfrac{x\sqrt{x}+y\sqrt{y}}{\sqrt{x}+\sqrt{y}}-\sqrt{xy}\right)\cdot\left(\dfrac{\sqrt{x}+\sqrt{y}}{x-y}\right)^2\)
\(=\left(x-\sqrt{xy}+y-\sqrt{xy}\right)\cdot\left(\dfrac{1}{\sqrt{x}-\sqrt{y}}\right)^2\)
\(=\left(\sqrt{x}-\sqrt{y}\right)^2\cdot\dfrac{1}{\left(\sqrt{x}-\sqrt{y}\right)^2}\)
=1