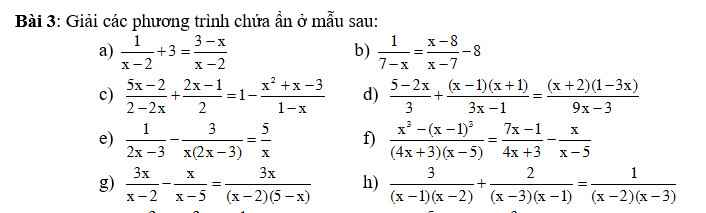a)
ĐKXĐ: \(x\ne2\)
Ta có: \(\dfrac{1}{x-2}+3=\dfrac{3-x}{x-2}\)
\(\Leftrightarrow\dfrac{1}{x-2}+\dfrac{3\left(x-2\right)}{x-2}=\dfrac{3-x}{x-2}\)
Suy ra: \(3x-6+1=3-x\)
\(\Leftrightarrow3x+x=3+6-1\)
\(\Leftrightarrow4x=8\)
hay x=2(loại)
b) ĐKXĐ: \(x\ne7\)
Ta có: \(\dfrac{1}{7-x}=\dfrac{x-8}{x-7}-8\)
\(\Leftrightarrow\dfrac{-1}{x-7}=\dfrac{x-8}{x-7}-\dfrac{8\left(x-7\right)}{x-7}\)
Suy ra: \(x-8-8x+56+1=0\)
\(\Leftrightarrow-7x+49=0\)
\(\Leftrightarrow-7x=-49\)
hay x=7(loại)
c) ĐKXĐ: \(x\ne1\)
Ta có: \(\dfrac{5x-2}{2-2x}+\dfrac{2x-1}{2}=1-\dfrac{x^2+x-3}{1-x}\)
\(\Leftrightarrow\dfrac{5x-2}{2\left(1-x\right)}+\dfrac{\left(2x-1\right)\left(1-x\right)}{2\left(1-x\right)}=\dfrac{2-2x}{2\left(1-x\right)}-\dfrac{2\left(x^2+x-3\right)}{2\left(1-x\right)}\)
Suy ra: \(5x-2+2x-2x^2-1+x=2-2x-2x^2-2x+6\)
\(\Leftrightarrow-2x^2+8x-3=-2x^2-4x+8\)
\(\Leftrightarrow8x+4x=8+3\)
\(\Leftrightarrow12x=11\)
hay \(x=\dfrac{11}{12}\)(nhận)
d) ĐKXĐ: \(x\ne\dfrac{1}{3}\)
Ta có: \(\dfrac{5-2x}{3}+\dfrac{\left(x-1\right)\left(x+1\right)}{3x-1}=\dfrac{\left(x+2\right)\left(1-3x\right)}{9x-3}\)
\(\Leftrightarrow\dfrac{\left(5-2x\right)\left(3x-1\right)}{3\left(3x-1\right)}+\dfrac{3\left(x^2-1\right)}{3\left(3x-1\right)}=\dfrac{\left(x+2\right)\left(1-3x\right)}{3\left(3x-1\right)}\)
Suy ra: \(15x-5-6x^2+2x+3x^2-3=x-3x^2+2-6x\)
\(\Leftrightarrow-3x^2+17x-8=-3x^2-5x+2\)
Suy ra: \(17x+5x=2+8\)
\(\Leftrightarrow x=\dfrac{5}{11}\)(thỏa ĐK)
e) ĐKXĐ: \(x\notin\left\{0;\dfrac{3}{2}\right\}\)
Ta có: \(\dfrac{1}{2x-3}-\dfrac{3}{x\left(2x-3\right)}=\dfrac{5}{x}\)
\(\Leftrightarrow\dfrac{x-3}{x\left(2x-3\right)}=\dfrac{5\left(2x-3\right)}{x\left(2x-3\right)}\)
Suy ra: \(x-3=10x-15\)
\(\Leftrightarrow-9x=-12\)
hay \(x=\dfrac{4}{3}\)(nhận)
a) \(\dfrac{1}{x-2}+3=\dfrac{3-x}{x-2}\)
dktc : x ≠ 2
MTC : x - 2
Quy đồng mẫu thức hai vế của phương trình :
⇒ \(\dfrac{1}{x-2}+\dfrac{3\left(x-2\right)}{x-2}=\dfrac{3-x}{x-2}\)
Suy ra : 1 + 3(x - 2) = 3 - x
\(\Leftrightarrow\) 1 + 3x - 6 - 3 + x = 0
\(\Leftrightarrow\) 4x - 8 = 0
\(\Leftrightarrow\) 4x = 8
\(\Leftrightarrow\) x = \(\dfrac{8}{4}=2\) (không thỏa mãn)
Vậy S = ∅
b) \(\dfrac{1}{7-x}=\dfrac{x-8}{x-7}-8\)
\(\dfrac{-1}{x-7}=\dfrac{x-8}{x-7}-8\)
dkxd : x ≠ 7
MTC : x - 7
Quy đồng mẫu thức hai vế của phương trình :
\(\Rightarrow\) \(\dfrac{-1}{x-7}=\dfrac{x-8}{x-7}-\dfrac{8\left(x-7\right)}{x-7}\)
Suy ra : -1 = x - 8 - 8(x - 7)
\(\Leftrightarrow\) -1 = x - 8 - 8x + 56
\(\Leftrightarrow\) -1 - x + 8 + 8x - 56 = 0
\(\Leftrightarrow\) -49 + 7x = 0
\(\Leftrightarrow\) 7x = 49
\(\Leftrightarrow\) x = \(\dfrac{49}{7}=7\) (không thỏa mãn)
Vậy S = ∅
c) \(\dfrac{5x-2}{2-2x}+\dfrac{2x-1}{2}=1-\dfrac{x^2+x-3}{1-x}\)
\(\dfrac{5x-2}{2\left(1-x\right)}+\dfrac{2x-1}{2}=1-\dfrac{x^2+x-3}{1-x}\)
dkxd : x ≠ 1
MTC : 2(1 - x)
Quy đồng mẫu thức hai vế của phương trình :
\(\Rightarrow\) \(\dfrac{5x-2}{2\left(1-x\right)}+\dfrac{\left(2x-1\right)\left(1-x\right)}{2\left(1-x\right)}=\dfrac{2\left(1-x\right)}{2\left(1-x\right)}\) - \(\dfrac{2\left(x^2+x-3\right)}{2\left(1-x\right)}\)
Suy ra : 5x - 2 + (2x - 1)(1 - x) = 2(1 - x) - 2(x2 + x - 3)
\(\Leftrightarrow\) 5x - 2 + 3x - 2x2 - 1 = 2 - 2x - 2x2 - 2x + 6
\(\Leftrightarrow\) 5x - 2 + 3x - 2x2 - 1 - 2 + 2x + 2x2 + 2x - 6 = 0
\(\Leftrightarrow\) 12x - 11 = 0
\(\Leftrightarrow\) 12x = 11
\(\Leftrightarrow\) x = \(\dfrac{11}{12}\) (thỏa mãn)
Vậy S = \(\left\{\dfrac{11}{12}\right\}\)
d) \(\dfrac{5-2x}{3}+\dfrac{\left(x-1\right)\left(x+1\right)}{3x-1}=\dfrac{\left(x+2\right)\left(1-3x\right)}{9x-3}\)
\(\dfrac{5-2x}{3}+\dfrac{\left(x-1\right)\left(x+1\right)}{3x-1}=\dfrac{\left(x+2\right)\left(1-3x\right)}{3\left(3x-1\right)}\)
dkxd : x ≠ \(\dfrac{1}{3}\)
MTC : 3(3x - 1)
Quy đồng mẫu thức hai vế của phương trình :
\(\Rightarrow\) \(\dfrac{\left(5-2x\right)\left(3x-1\right)}{3\left(3x-1\right)}+\dfrac{3\left(x-1\right)\left(x+1\right)}{3\left(3x-1\right)}\) = \(\dfrac{\left(x+2\right)\left(1-3x\right)}{3\left(3x-1\right)}\)
Suy ra : (5 - 2x)(3x - 1) + 3(x - 1)(x + 1) = (x + 2)(1 - 3x)
\(\Leftrightarrow\) 17x - 5 - 6x2 + 3(x2 - 1) = -5x - 3x2 + 2
\(\Leftrightarrow\) 17x - 5 - 6x2 + 3x2 - 3 + 5x + 3x2 - 2 = 0
\(\Leftrightarrow\) 22x - 10 = 0
\(\Leftrightarrow\) 22x = 10
\(\Leftrightarrow\) x = \(\dfrac{10}{22}=\dfrac{5}{11}\) (thỏa mãn)
Vậy S = \(\left\{\dfrac{5}{11}\right\}\)
Chúc bạn học tốt
f)
ĐKXĐ: \(x\notin\left\{-\dfrac{3}{4};5\right\}\)
Ta có: \(\dfrac{x^3-\left(x-1\right)^3}{\left(4x+3\right)\left(x-5\right)}=\dfrac{7x-1}{4x+3}-\dfrac{x}{x-5}\)
\(\Leftrightarrow\dfrac{x^3-x^3+3x^2-3x+1}{\left(4x+3\right)\left(x-5\right)}=\dfrac{\left(7x-1\right)\left(x-5\right)}{\left(4x+3\right)\left(x-5\right)}-\dfrac{x\left(4x+3\right)}{\left(4x+3\right)\left(x-5\right)}\)
Suy ra: \(3x^2-3x+1=7x^2-35x-x+5-4x^2-3x\)
\(\Leftrightarrow3x^2-3x+1=3x^2-39x+5\)
\(\Leftrightarrow-3x+39x=5-1\)
\(\Leftrightarrow36x=4\)
hay \(x=\dfrac{1}{9}\)(thỏa ĐK)
g)
ĐKXĐ: \(x\notin\left\{2;5\right\}\)
Ta có: \(\dfrac{3x}{x-2}-\dfrac{x}{x-5}=\dfrac{3x}{\left(x-2\right)\left(5-x\right)}\)
\(\Leftrightarrow\dfrac{3x\left(x-5\right)}{\left(x-2\right)\left(x-5\right)}-\dfrac{x\left(x-2\right)}{\left(x-2\right)\left(x-5\right)}=\dfrac{-3x}{\left(x-2\right)\left(x-5\right)}\)
Suy ra: \(3x^2-15x-x^2+2x+3x=0\)
\(\Leftrightarrow2x^2-9x=0\)
\(\Leftrightarrow x\left(2x-9\right)=0\)
\(\Leftrightarrow\left[{}\begin{matrix}x=0\left(nhận\right)\\x=\dfrac{9}{2}\left(nhận\right)\end{matrix}\right.\)