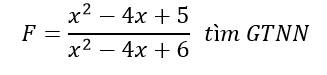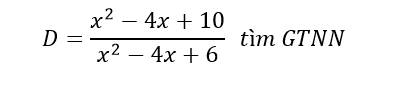Phương trình bậc nhất một ẩn

c: =>4y^2-4y+1=0

=>(2y-1)^2=0

=>2y-1=0

=>y=1/2

d: =>y^2-2y-80=0

=>(y-10)(y+8)=0

=>y=10 hoặc y=-8

\(S=\left(3x+1\right)\left(6-x\right)=18-3x^2+6-x=-3x^2+17x+6\)

Đặt \(A=-3x^2+17x+6\)

\(S_{max}\Leftrightarrow A_{max}=-3\left(x^2-\dfrac{17}{3}x-2\right)=-3\left[x^2-2.x.\dfrac{17}{6}+\left(\dfrac{17}{6}\right)^2-\left(-\dfrac{17}{6}\right)^2-2\right]\)

\(=-3\left[\left(x-\dfrac{17}{6}\right)^2-\dfrac{361}{36}\right]\)

\(=-3\left(x-\dfrac{17}{6}\right)^2+\dfrac{361}{12}\)

Vì \(-3\left(x-\dfrac{17}{6}\right)^2\le0\forall x\in R\)

\(\Rightarrow-3\left(x-\dfrac{17}{6}\right)^2+\dfrac{361}{12}\le\dfrac{361}{12}\forall x\in R\)

\(\Rightarrow A_{max}=\dfrac{361}{12}\Leftrightarrow\left(x-\dfrac{17}{6}\right)^2=0\Leftrightarrow x=\dfrac{17}{6}\)

\(\Rightarrow S_{max}\Leftrightarrow x=\dfrac{17}{6}\)

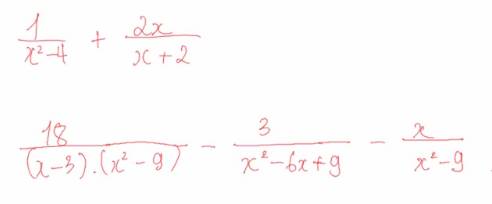
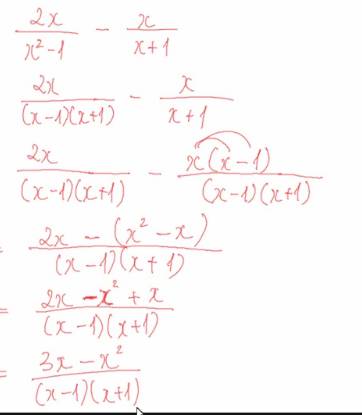
\(\dfrac{1}{x^2-4}+\dfrac{2x}{x+2}=\dfrac{1}{\left(x-2\right)\left(x+2\right)}+\dfrac{2x}{x+2}=\dfrac{1+2x\left(x-2\right)}{\left(x-2\right)\left(x+2\right)}=\dfrac{1+2x^2-4x}{\left(x+2\right)\left(x-2\right)}\)

trên bài mink đã ẩn đi bước quy đồng!!

\(\dfrac{18}{\left(x-3\right)\left(x^2-9\right)}-\dfrac{3}{x^2-6x+9}-\dfrac{x}{x^2-9}=\dfrac{18}{\left(x-3\right)\left(x+3\right)\left(x-3\right)}-\dfrac{3}{\left(x-3\right)^2}-\dfrac{x}{\left(x-3\right)\left(x+3\right)}\)

\(=\dfrac{18}{\left(x-3\right)^2\left(x+3\right)}-\dfrac{3}{\left(x-3\right)^2}-\dfrac{x}{\left(x-3\right)\left(x+3\right)}=\dfrac{18-3\left(x+3\right)-x\left(x-3\right)}{\left(x-3\right)^2\left(x+3\right)}\)

\(=\dfrac{18-3x-9-x^2+3x}{\left(x-3\right)^2\left(x+3\right)}=\dfrac{9-x^2}{\left(x-3\right)^2\left(x+3\right)}=\dfrac{-\left(x-3\right)\left(x+3\right)}{\left(x-3\right)^2\left(x+3\right)}=\dfrac{-1}{x-3}\)

Bạn ơi làm ý nào đéy ??

2 ý trên à ?????

`F=[x^2-4x+5]/[x^2-4x+6]=1-1/[x^2-4x+6]=1-1/[(x-2)^2+2]`

Vì `(x-2)^2 >= 0 AA x<=>(x-2)^2+2 >= 2 AA x`

        `=>1/[(x-2)^2+2] <= 1/2 AA x`

        `=>-1/[(x-2)^2+2] >= -1/2 AA x<=>F >= 1/2 AA x`

Dấu "`=`" xảy ra `<=>x-2=0<=>x=2`

______________________________________________

`D=[x^2-4x+10]/[x^2-4x+6]=1+4/[x^2-4x+6]=1+4/[(x-2)^2+2]`

Vì `(x-2)^2 >= 0 AA x<=>(x-2)^2+2 >= 2 AA x`

           `<=>4/[(x-2)^2+2] <= 2 AA x<=>D <= 3 AA x`

Dấu "`=`" xảy ra `<=>x-2=0<=>x=2`

lỗi nữa

k0 spam

bai dau???????????????????????????

=>x²=7

hay \(x=\pm\sqrt{7}\)

a: \(=\dfrac{2x+2-2}{\left(x-2\right)\left(x+1\right)}\cdot\left(1+x\right)\)

=2x/x-2

b: \(=\dfrac{2}{xy}:\dfrac{y-x}{xy}-\dfrac{x+y}{x-y}\)

\(=\dfrac{-2}{x-y}-\dfrac{x+y}{x-y}=\dfrac{-2-x-y}{x-y}\)

c: \(=\dfrac{x^2+2xy+y^2-x^2+2xy-y^2+4y^2}{2\left(x+y\right)\left(x-y\right)}\cdot\dfrac{x-y}{2y}\)

\(=\dfrac{4y^2+4xy}{2\left(x+y\right)}\cdot\dfrac{1}{2y}=\dfrac{4y\left(x+y\right)}{4y\left(x+y\right)}=1\)

a: Để phương trình vô nghiệm thì \(m\in\varnothing\)

b: Để phươg trình có vô số nghiệm thì m-1=0

hay m=1

c: Để phương trình có nghiệm duy nhất thì m-1<>0

hay m<>1

Chọn B