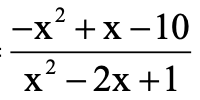Question

tìm giá trị lớn nhất của e = 6a+3-9a^2

Answer 1

\(E=6a+3-9a^2\)

\(=-\left(9a^2-6a-3\right)\)

\(=-\left(\left(3a\right)^2-2.3a+1-4\right)\)

\(=-\left(3a-1\right)^2+4\)

Vì \(-\left(3a-1\right)^2\le0\forall a\)

\(\Rightarrow E\le4\forall x\)

\(MaxE=4\Leftrightarrow x=\dfrac{1}{3}\)

Answer 2

\(e=6a+3-9a^2\)

\(=-9a^2+6a-1+4\)

\(=-\left(9a^2-6a+1\right)+4\)

\(=-\left[\left(3a\right)^2-2\cdot3a\cdot1+1^2\right]+4\)

\(=-\left(3a-1\right)^2+4\)

Ta thấy: \(\left(3a-1\right)^2\ge0\forall a\)

\(\Rightarrow-\left(3a-1\right)^2\le0\forall a\)

\(\Rightarrow-\left(3a-1\right)^2+4\le4\forall a\)

Dấu \("="\) xảy ra \(\Leftrightarrow3a-1=0\Leftrightarrow a=\dfrac{1}{3}\)

Vậy \(e_{max}=4\) khi \(a=\dfrac{1}{3}.\)

#\(Toru\)