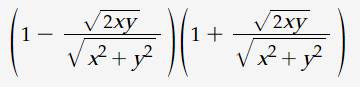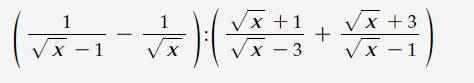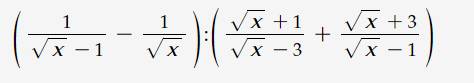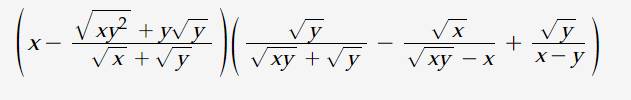Sửa đề: a = b => x = y
\(P=\left(1-\dfrac{\sqrt{2xy}}{\sqrt{x^2+y^2}}\right)\left(1+\dfrac{\sqrt{2xy}}{\sqrt{x^2+y^2}}\right)\) (ĐK: \(x,y>0\))
\(=1-\left(\dfrac{\sqrt{2xy}}{\sqrt{x^2+y^2}}\right)^2\)
\(=1-\dfrac{\left(\sqrt{2xy}\right)^2}{\left(\sqrt{x^2+y^2}\right)^2}\)
\(=1-\dfrac{2xy}{x^2+y^2}\)
\(=\dfrac{x^2+y^2-2xy}{x^2+y^2}\)
\(=\dfrac{\left(x-y\right)^2}{x^2+y^2}\)
Khi x = y, ta được: \(P=\dfrac{\left(x-y\right)^2}{x^2+y^2}=\dfrac{\left(x-x\right)^2}{x^2+y^2}=0\)
#Urushi